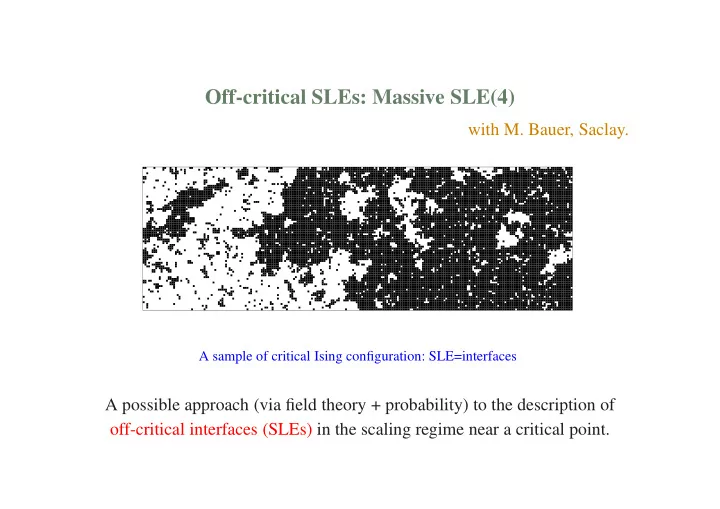

Off-critical SLEs: Massive SLE(4) with M. Bauer, Saclay. A sample of critical Ising configuration: SLE=interfaces A possible approach (via field theory + probability) to the description of off-critical interfaces (SLEs) in the scaling regime near a critical point.
Many examples: Loop Erased Random Walks: – Ising model at T → T c ; – Percolation at p → p c ; – LERW at x → x c ; – SAW at different fugacity; – etc... Break conformal invariance Weight: w γ = ∑ r → γ x | r | At criticality x c
Plan: • Toy model: random walks • SAW as an example • Drift, partition function and field theory • Massive SLE(4) • Massive LERW
A toy model: random walks j = 1 ε j be a (biaised) random walks of step a , • Let X N = a ∑ N with ε j = ± with proba p , 1 − p . Set X t = a ( N + − N − ) and t = a 2 N = a 2 ( N + + N − ) . The scaling limit is for N → ∞ , a → 0 at t fixed: At ”criticality” p c = 1 / 2, X t = Brownian motion: ( dX t ) 2 = dt . • ”Near criticality” p → 1 / 2 as a → 0? The ratio of proba of a walk at p and p c is ( 2 p ) N + ( 2 ( 1 − p )) N − , so: M ≡ e S = ( 2 p ) N + ( 2 ( 1 − p )) N − E p [ ··· ] = E p = 1 / 2 [ M ··· ] with To get a finite weight M , we need p ≃ ( 1 + aµ ) / 2 as a → 0 (scaling limit). The ’action’ is then S t = µX t − µ 2 t / 2 and in the continuum: M t ≡ e S t = e µX t − µ 2 t / 2 E µ [ ··· ] = E Br [ M t ··· ] ( a martingale ) with W.r.t. E µ , X t now has a drift: dX t = dB t + µdt .
• A martingale for some stochastic process is a time dependent random variable, say t → M t , such that its expectation conditioned on the process up to time s is M s , i.e. statistically conserved quantity. • Going off-criticality amounts to weight by a martingale (which depends on the perturbation). It modifies (adds a drift to) the stochastic equation. This is the approach we (try to) adapt to off-critical SLEs....... • Grisanov theorem: If M t martingale for Brownian motion X t : M − 1 dM t = F t dX t , t then w.r.t. to ˆ E [ ··· ] = E Br [ M t ··· ] , B t a ˆ X t satisfies dX t = d ˆ t dt with ˆ B t + F E -Brownian motion. In physics, this is known as the M.S.R. path integral representation. • Other works on off-critical SLEs: Nolin-Werner, Makarov-Smirnov, ...
SAW as an example SAW= self-avoiding walk Weight: w γ = x | γ | with | γ | = nbr . steps. Proba: w γ / Z D with Z D = ∑ γ w γ the partition function. At criticality x = x c , conjecturally SAW=SLE(8/3) in the continuum, a → 0. • How to take the scaling limit? x | γ | − 1 ∑ − 1 ∑ x | γ | = Z crit . c ( x / x c ) | γ | = E crit . [( x / x c ) | γ | ] Z D / Z crit . = Z crit . D D D γ γ At criticality, | γ | is related to the macroscopic size via the fractal dimension: | γ | ≃ [ ℓ ( γ ) / a ] d κ . The scaling limit is x − x c ≃ − a d κ µ and: x c = E crit . [ e − µL D ( γ ) ] L D ( γ ) ≃ a d κ | γ | ≡ ′ natural parametrisation ′ Z D / Z crit . , D
• Off-critical weight, off-critical drift and partition function. (For SAW) the off-critical weighting is by the natural parametrization: D E crit . [ e − µL D ( γ ) O ] with Z D = E crit . [ e − µL D ( γ ) ] . E µ [ O ] = Z − 1 If the observable O only depends on the curve up to time t ( F t -measurable): D E crit . [ e − µL D ( γ ) | F t ] M t = Z − 1 E µ [ O ] = E crit . [ M t O ] with Girsanov theorem tells that the off-critical drift is M − 1 dM t . t Since L D ( γ ) counts the number of steps, we expect an additivity pro- perty: L D ( γ [ 0 , s ] ) = L D ( γ [ 0 , t ] )+ L D \ γ [ 0 , t ] ( γ [ t , s ] ) ⇒ M t = e − µL D ( γ [ 0 , t ] ) Z D \ γ [ 0 , t ] For the off-critical weighting martingale = Z D Ie. → ”surface energy” + ”ratio of partition function”.
Off-critical SLE and field theory Loewner equation: dg t ( z ) 2 = g t ( z ) − ξ t dt ξ t : driving source. • At criticality, ξ t is a 1D Brownian motion, E [ ξ 2 t ] = κ t . • Off-criticality, ξ t not a Brownian motion (it depends on the perturbation): — short distance: by scaling we expect: λ − 1 ξ λ 2 t → √ κ B t as λ → 0. — decomposition: from above, we expect: d ξ t = √ κ dB t + F t dt F t off-critical drift term (perturbation dependent). — But off-critical measure can be singular w.r.t. critical one (cf percolation [Nolin-Werner] or infinite domain).
• From field theory, the off-critical drift reads dM t = κ∂ ξ t log ( e E t ( γ [ 0 , t ] ) Z t ) . t = M − 1 . F t The off-critical weighting martingale M t = e E t Z t with Z t = Z off − crit . / Z crit . , t t the ratio of the partition functions in the domain cut along the curve. • Previous discussion follows (naively) from basic stat. mech. principles. (because the measure on curves induced by the Boltzmann weights is the ratio of partition functions). • The measure on curves is induced (via the Loewner equation) from that on the driving source ξ t solution of the SDE: d ξ t = √ κ dB t + F t dt .
Massive SLE(4) To appear... SLE(4) in a perturbed environment. Massive bosonic free field Z d 2 x 8 π [( ∂ X ) 2 + m 2 ( x ) X 2 ] S = with Dirichlet boundary conditions. • SLE(4) = discontinuity curve of a massless boson (Sheffield-Schramm). • At criticality, Gaussian free field (in upper half plane H ): √ = G 0 ( z , w ) = − log | z − w � X ( z ) X ( w ) � conn . w | 2 � X ( z ) � H = 2 Im ( log z ) , H z − ¯ Perturbing (composite) operator: X 2 ( z ) = lim w → z X ( z ) X ( w )+ log | z − w | 2 . R d 2 x t = 4 ∂ ξ log Z [ m ] with Z [ m ] 8 π m 2 ( x ) X 2 ( x ) � H t . = � e − • Off-critical drift F t t H t = the cutted upper half plane.
• First order computation. X 2 is not a scalar but � X 2 ( z ) � H t is a (local) martingale: √ � X 2 ( z ) � H t = ϕ t ( z ) 2 + log ρ t ( z ) , ϕ t ( z ) = ρ t ( z ) = conf . radius 2 Im ( log g t ( z )) , R d 2 x To 1rst order, Z [ m ] 8 π m 2 ( x ) � X 2 ( z ) � H t + ··· . = 1 − t As a CFT correlation, ϕ t ( z ) is a martingale: d ϕ t ( z ) = θ t ( z ) dB t . R d 2 x 4 π m 2 ( x ) θ t ( x ) ϕ t ( x )+ ··· . t = − To 1rst order, the drift is: F • All order computation. Computable since the theory is Gaussian (only connected diagrams contribute), and to all orders: Z d 2 x Z d 2 x 4 π m 2 ( x ) θ t ( x ) Φ [ m ] 4 π m 2 ( x ) Θ [ m ] t ( x ) ϕ t ( x ) t = − ( x ) = − F t with ( − ∆ + m 2 ) Φ [ m ] = 0 in H t with Dirichlet b . c . t
• Massive � X ( x ) � H t = (conditioned) proba for γ to be on the right of x . It should be/It is a (massive) martingale with this drift. This follows from Hadamard formula. This is the way Makarov-Smirnov computed the drift. • Perfect matching (should be true from basic stat. mech.): E [ m ] [ �··· ( any X correlation ) ···� H t ] = �··· ( any X correlation ) ···� H → Reorganisation of the statistical sum: – first sum over fields at fixed interfaces – then on possible interfaces shapes. • The curve γ is (a.s.) the discontinuity curve. • Massive harmonic navigator (with killing)
Massive LERW LERW=random walk with loop erased. Weight: w γ = ∑ r → γ x | r | At criticality x c SLE(2) = [c=-2] = symplectic fermions. • Scaling limit x − x c = − a 2 µ and the partition function Z D = ∑ r x | r | : x c Z Z D = E Br . [ e − µ τ D ] = E Br . [ exp ( − d 2 x µ ( x ) ℓ D ( x ))] Brownian local time ℓ D ( x ) is conformal of dimension zero. • Fugacity perturbation is the massive perturbation: Z d 2 x R d 2 x Z 8 π µ ( x )( χ + χ − ) Z t = � ψ + e − 8 π [( ∂χ + )( ∂χ − )+ µ ( x )( χ + χ − )] , ψ − � S = ψ ± = ∂χ ± are creating/annihilating the curve. • and a similar story.......
....... Thank You .......
Recommend
More recommend