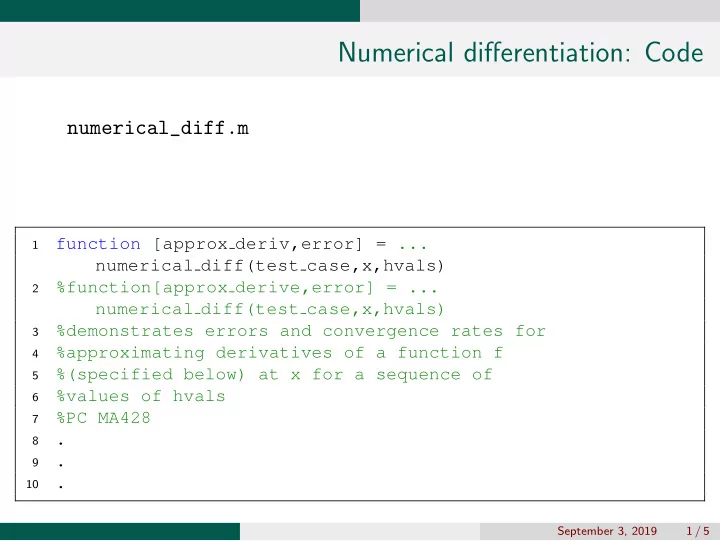
Numerical differentiation: Code numerical_diff.m function [approx - PowerPoint PPT Presentation

Numerical differentiation: Code numerical_diff.m function [approx deriv,error] = ... 1 numerical diff(test case,x,hvals) %function[approx derive,error] = ... 2 numerical diff(test case,x,hvals) %demonstrates errors and convergence rates for 3 %approximating derivatives of a function f 4 %(specified below) at x for a sequence of 5 %values of hvals 6 %PC MA428 7 . 8 . 9 . 10 September 3, 2019 1 / 5

Numerical differentiation: Code run_numerical_diff.m %This script runs the numerical diff to print out 1 %the error for each h. Test cases: 2 % A - first order forward difference 3 % B - Centred finite difference 4 % C - Richard Extrapolation formula 5 h0 =1; % initial value of h 6 x0 = (1/3)*pi; %point to estimate derivative 7 n = 17; %number of refinements of h 8 hvals=zeros(n,1); 9 for i=1:n 10 hvals(i) = h0*(1/10ˆ(i-1)); 11 end 12 disp(' h Approx Deriv Error'); 13 [approx deriv,error]=numerical diff('C',x0,hvals); 14 disp vec = [hvals,approx deriv,error]; 15 format short e 16 disp(disp vec); 17 September 3, 2019 2 / 5

Numerical differentiation First order forward difference f ′ ( x 0 ) = f ( x 0 + h ) − f ( x 0 ) − h 2 f ′′ ( c x ) , x 0 = π f ( x ) = sin( x ) h 3 , Errors: >> run_numerical_diff h Approx Deriv Error 1.0000e+00 2.2626e-02 4.7737e-01 1.0000e-01 4.5590e-01 4.4098e-02 1.0000e-02 4.9566e-01 4.3384e-03 1.0000e-03 4.9957e-01 4.3310e-04 1.0000e-04 4.9996e-01 4.3302e-05 1.0000e-05 5.0000e-01 4.3301e-06 1.0000e-06 5.0000e-01 4.3303e-07 1.0000e-07 5.0000e-01 4.3007e-08 1.0000e-08 5.0000e-01 3.0387e-09 1.0000e-09 5.0000e-01 4.1370e-08 1.0000e-10 5.0000e-01 4.1370e-08 . . . 1.0000e-16 0 5.0000e-01 September 3, 2019 3 / 5

Numerical differentiation Centered finite difference formula f ′ ( x 0 ) = f ( x 0 + h ) − f ( x 0 − h ) − h 2 2 ( f ′′ ( c 1 ) + f ′′ ( c 2 )) , x 0 = π f ( x ) = sin( x ) 2 h 3 , Errors: >> run_numerical_diff h Approx Deriv Error 1.0000e+00 4.2074e-01 7.9265e-02 1.0000e-01 4.9917e-01 8.3292e-04 1.0000e-02 4.9999e-01 8.3333e-06 1.0000e-03 5.0000e-01 8.3333e-08 1.0000e-04 5.0000e-01 8.3383e-10 1.0000e-05 5.0000e-01 7.8268e-12 1.0000e-06 5.0000e-01 4.1133e-11 1.0000e-07 5.0000e-01 2.9193e-10 . . . 1.0000e-13 4.9960e-01 3.9964e-04 1.0000e-14 4.9960e-01 3.9964e-04 1.0000e-15 5.5511e-01 5.5112e-02 1.0000e-16 0 5.0000e-01 September 3, 2019 4 / 5

Numerical differentiation Richardson Extrapolation formula f ′ ( x 0 ) = 4 3 F ( h 2 ) − 1 3 F ( h ) + O ( h 4 ) , x 0 = π f ( x ) = sin( x ) 3 , Errors: >>run_numerical_diff h Approx Deriv Error 1.0000e+00 4.9899e-01 1.0111e-03 1.0000e-01 5.0000e-01 1.0414e-07 1.0000e-02 5.0000e-01 1.0419e-11 1.0000e-03 5.0000e-01 5.5178e-14 1.0000e-04 5.0000e-01 1.0550e-12 1.0000e-05 5.0000e-01 6.9763e-12 1.0000e-06 5.0000e-01 1.0690e-10 1.0000e-07 5.0000e-01 1.1884e-09 1.0000e-08 5.0000e-01 3.0387e-09 . . . 1.0000e-14 5.1440e-01 1.4403e-02 1.0000e-15 4.0708e-01 9.2918e-02 1.0000e-16 0 5.0000e-01 September 3, 2019 5 / 5