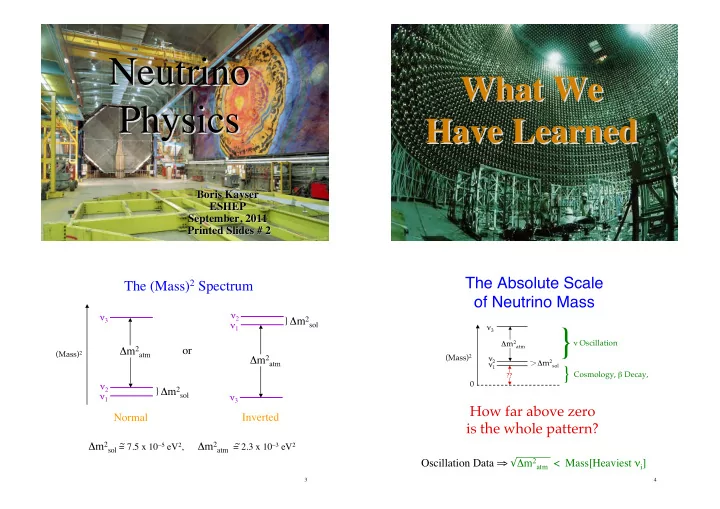

Neutrino Neutrino What We What We Physics Physics Have Learned Have Learned Boris Kayser Kayser Boris ESHEP ESHEP September, , 2011 2011 September Printed Slides # 2 Printed Slides # 2 1 2 The Absolute Scale The (Mass) 2 Spectrum of Neutrino Mass ! 2 ! 3 } " m 2sol ! 1 } ! 3 ! Oscillation " m 2 atm " m 2atm or (Mass) 2 (Mass) 2 " m 2atm ! 2 " m 2 ! 1 sol } Cosmology, # Decay, ?? 0 ! 2 } " m 2sol ! 1 ! 3 How far above zero Normal Inverted is the whole pattern? ~ ~ " m 2sol = 7.5 x 10 –5 eV 2 , " m 2atm = 2.3 x 10 –3 eV 2 Oscillation Data $ %" m 2atm < Mass[Heaviest ! i ] 3 4
The Upper Bound From Tritium The Upper Bound From Cosmology Cosmology is wonderful, but there are known Neutrino mass affects large scale structure. loopholes in its argument concerning neutrino mass. Cosmological Data + Cosmological Assumptions $ The absolute neutrino mass can in principle & m i < (0.17 – 1.0) eV . also be measured by the kinematics of ! decay. ) ( Seljak, Slosar, McDonald 3 H " 3 He + e # + $ i ; i = 1, 2, or 3 Mass( ! i ) Tritium decay: Hannestad; Pastor BR 3 H " 3 He + e # + $ i ( ) % U ei 2 If there are only 3 neutrinos, 3 H " 3 He + e # + $ i In , the bigger m i is, the 0.04 eV < Mass[Heaviest ! i ] < (0.07 – 0.4) eV ~ smaller the maximum electron energy is. %" m 2atm Cosmology There are 3 separate thresholds in the ! energy spectrum. 5 6 Leptonic Mixing The ! energy spectrum is modified according to — ) 2 " m i ) 2 # E 0 " E 2 2 # E 0 " m i [ ] ( E 0 " E [ ] $ U ei ( E 0 " E ) ( E 0 " E ( ) " E % i This has the consequence that — Maximum ! energy when ! energy Mass eigenstate Flavor eigenstate there is no neutrino mass | ! i > = & U ' i | ! ' > . Present experimental energy resolution ' e, µ , or ( Leptonic Mixing Matrix is insufficient to separate the thresholds. Flavor- ' fraction of ! i = |U ' i | 2 . Measurements of the spectrum bound the average neutrino mass — When a ! i interacts and produces a charged lepton, 2 m i 2 m " = U ei # the probability that this charged lepton will be of i flavor ' is |U ' i | 2 . Mainz & Presently: m " < 2 eV Troitzk 7 8
The spectrum, showing its approximate flavor content, is Bounded by reactor exps. with L ~ 1 km " 3 # " µ + " $ From max. atm. mixing, 2 sin 2 ) 13 ! 3 ! 3 ! 2 } " m 2 { sol From ! µ (Up) oscillate ! 1 " m 2 atm but ! µ (Down) don’t " m 2 (Mass) 2 atm or { In LMA–MSW, P sol ( ! e * ! e ) (Mass) 2 " m 2 = ! e fraction of ! 2 atm ! 2 } From distortion of ! e (reactor) " m 2 ! 2 sol and ! e (solar) spectra } ! 1 " m 2 sol ! 1 ! 3 { From max. atm. mixing, ! 1 + ! 2 includes ( ! µ – ! ( )/ √ 2 sin 2 ) 13 Inverted Normal ! ( [|U ( i | 2 ] ! e [|U ei | 2 ] ! µ [|U µ i | 2 ] ! e [|U ei | 2 ] ! µ [|U µ i | 2 ] ! ( [|U ( i | 2 ] 9 10 Recent Evidence For Non-Zero " 13 The Mixing Matrix In an experiment where L/E is too small for the small Solar Atmospheric Cross-Mixing 2 # m 2 2 $ m 1 2 splitting to be seen, " m 21 # s 13 e " i * & # 1 0 0 & c 13 0 # c 12 s 12 0 & % ( % ( % ( 2 sin 2 % m 31 U = 0 c 23 s 23 0 1 0 " s 12 c 12 0 & L ) ) ) % ( ( ) $ 4 U µ 3 U e 3 2 % ( % ( P " µ # " e ( + % " s 13 e i * ( ' 4 E * % ( % ( 0 " s 23 c 23 0 c 13 0 0 1 $ ' $ ' $ ' & ) L = sin 2 2 , 13 sin 2 , 23 sin 2 % m 31 2 # e i + 1 /2 & ( + 0 0 Hints?? 4 E ' * % ( c ij , cos ) ij e i + 2 /2 0 0 ) % ( s ij , sin ) ij % ( 0 0 1 $ ' T2K has looked for in a long-baseline " µ # " e Majorana CP experiment: ) 12 ! ) sol ! 34°, ) 23 ! ) atm ! 39-51°, ) 13 < 12° ~ phases + would lead to P( ! ' * ! # ) " P( ! ' * ! # ). CP But note the crucial role of s 13 , sin ) 13 . 11 12
The T2K experiment (Designed to seek $ µ * $ e ) Source Far Detector # * µ + $ µ Super-K $ These take . ” e g the " m 2 r 295 km a 21 l “ contributions e b d Near l and matter effects E = 0.6 GeV u o c Detector into account. " 13 The far detector is near the first 2 oscillation maximum. " m 31 Expectation Observation T2K sees 6 $ e candidate events in the far detector, whereas 1.5 are expected if " 13 = 0. 13 14 There Is Nothing Special About ) 13 MINOS, not designed to look for , sees " µ # " e All mixing angles must be nonzero for CP in oscillation. 62 candidate events where 50 are expected if " 13 = 0. For example — ( ) $ P " µ # " e ( ) = 2cos % 13 sin2 % 13 sin2 % 12 sin2 % 23 sin & While not highly significant by itself, P " µ # " e this result is consistent with that from T2K. ) , ) , ) , ' sin ( m 231 L sin ( m 232 L sin ( m 221 L + . + . + . * 4 E - * 4 E - * 4 E - In the factored form of U, one can put + next to ) 12 instead of ) 13 . 15 16
The Majorana CP Phases Looking to the Future Looking to the Future The phase ' i is associated with neutrino mass eigenstate ! i : The Open The Open U ' i = U 0 ' i exp(i ' i /2) for all flavors ' . Questions Questions Amp( ! ' * ! # ) = & U ' i* exp(– im i2 L/2E) U # i i is insensitive to the Majorana phases ' i . Only the phase + can cause CP violation in neutrino oscillation. 17 18 • What is the absolute scale What is ) 13 ? of neutrino mass? How close to maximal is ) 23 ? •Are neutrinos their own antiparticles? •Is the spectrum like or ? •Are there more than 3 mass eigenstates? •Are there “sterile” neutrinos? •Do neutrino interactions violate CP? •What are the neutrino magnetic Is P( ! ' * ! # ) - P( ! ' * ! # ) ? and electric dipole moments? 19 20
Why the Why the • What can neutrinos and the universe tell us about one another? Questions Are Questions Are • Is CP violation involving neutrinos the key to understanding the matter – Interesting, , and and Interesting antimatter asymmetry of the universe? How They May Be How They May Be •What physics is behind neutrino mass? Answered Answered •What surprises are in store? 21 22 What Is the Question? For each mass eigenstate ! i , and given helicty h, does — Does ! = ! ? Does ! = ! ? • ! i (h) = ! i (h) (Majorana neutrinos) or • ! i (h) ≠ ! i (h) (Dirac neutrinos) ? Equivalently, do neutrinos have Majorana masses ? If they do, then the mass eigenstates are Majorana neutrinos . 23 24
Majorana Masses A Majorana mass for any fermion f causes f f. Majorana Majorana Quark and charged-lepton Majorana masses mass mass are forbidden by electric charge conservation. Their effect: X X or ! ! ! ! Neutrino Majorana masses would make the neutrinos very distinctive. Majorana masses mix ! and ! , so they do not conserve the Lepton Number L that distinguishes Majorana neutrino masses have a different origin leptons from antileptons: than the quark and charged-lepton masses. L( ! ) = L( ! – ) = –L( ! ) = –L( ! + ) = 1 25 26 In the SM, the top quark mass comes from — Why Majorana Masses Majorana Neutrinos Top quark mass m t Higgs field As a result of K 0 K 0 mixing, the neutral K G t H 0 t R t L " G t H 0 mass eigenstates are — 0 t R t L K S,L . (K 0 ± K 0 )/ % 2 . K S,L = K S,L . Coupling Vacuum expectation value constant Such an operator does not mix quark and antiquark. Majorana masses induce ! ! mixing. ( ) ( ) t t Its effect: X As a result of ! ! mixing, the neutrino mass m t eigenstate is — A Majorana mass term does mix neutrino and antineutrino. ! i = ! + ! . ! i = ! i . A Majorana mass term must have a different origin than the quark and charged-lepton masses. 27 28
SM Interactions Of SM Interactions Of A Majorana Neutrino A Dirac Neutrino We have 4 mass-degenerate states: We have only 2 mass-degenerate states: Conserved L makes ! – ! makes ! – ! +1 makes ! + ! makes ! + ! –1 The weak interactions violate parity . These states, when Ultra ! Rel., do not interact. (They can tell Left from Right .) ( ( The weak interaction ! An incoming left-handed neutral lepton makes ! – . is Left Handed. An incoming right-handed neutral lepton makes ! + . 29 30 Majorana Masses Split Can a Majorana Neutrino Have Dirac Neutrinos an Electric Charge Distribution Distribution ? No! A Majorana mass term splits a Dirac neutrino into two Majorana neutrinos. – + 2 Majorana neutrino + – = Anti 4 Splitting due to Majorana mass Dirac Majorana neutrino 2 neutrino But for a Majorana neutrino — Anti ( ! ) = ! 31 32
Why Most Theorists To Determine Expect Majorana Masses To Determine The Standard Model (SM) is defined by the fields it Whether Whether contains, its symmetries (notably weak isospin invariance), and its renormalizability. Majorana Masses Majorana Masses Leaving neutrino masses aside, anything allowed by the SM symmetries occurs in nature. Occur in Nature Occur in Nature Majorana mass terms are allowed by the SM symmetries. Then quite likely Majorana masses 34 occur in nature too. 33 The Promising Approach — Seek Whatever diagrams cause 0 !## , its observation would imply the existence of a Majorana mass term: Neutrinoless Double Beta Decay [0 !## ] (Schechter and Valle) e – e – e – e – 0 !## ! ! Nucl Nucl’ u d d u W W We are looking for a small Majorana neutrino mass. Thus, we will need a lot of parent nuclei (say, one ton of them). ! * ! : A (tiny) Majorana mass term / 0 !## ! i = ! i 35 36
Recommend
More recommend