Network Calculus: Reference Material: J.-Y. LeBoudec and Patrick - PDF document

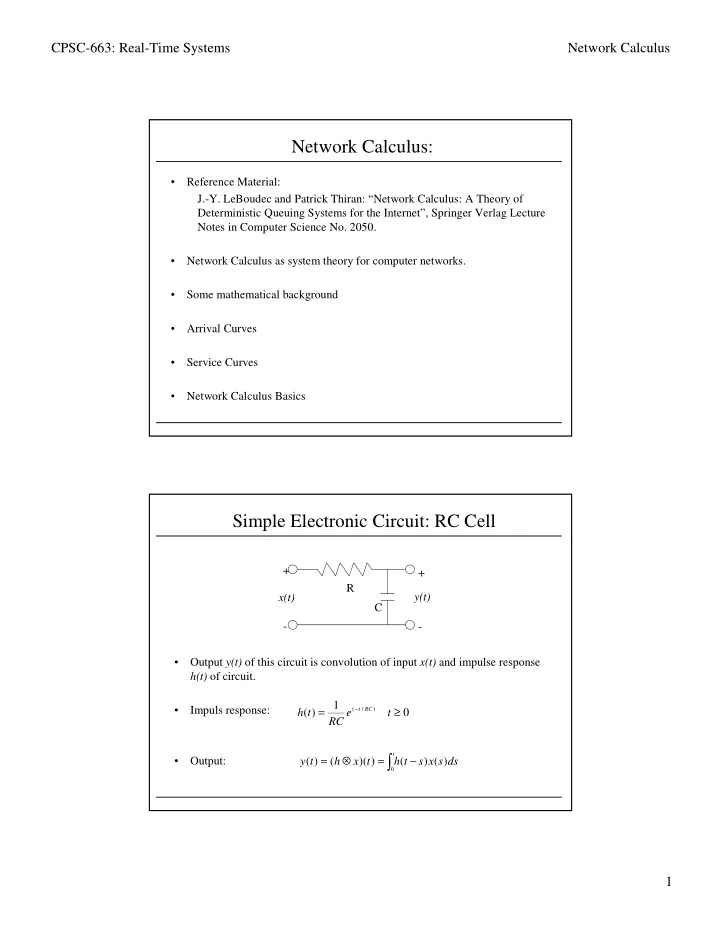
CPSC-663: Real-Time Systems Network Calculus Network Calculus: • Reference Material: J.-Y. LeBoudec and Patrick Thiran: “Network Calculus: A Theory of Deterministic Queuing Systems for the Internet”, Springer Verlag Lecture Notes in Computer Science No. 2050. • Network Calculus as system theory for computer networks. • Some mathematical background • Arrival Curves • Service Curves • Network Calculus Basics Simple Electronic Circuit: RC Cell + + R x(t) y(t) C - - • Output y(t) of this circuit is convolution of input x(t) and impulse response h(t) of circuit. 1 = ≥ • Impuls response: − ( t / RC ) h ( t ) e t 0 RC ∫ t = ⊗ = − • Output: y ( t ) ( h x )( t ) h ( t s ) x ( s ) ds 0 1

CPSC-663: Real-Time Systems Network Calculus Greedy Shaper • A shaper forces an input traffic flow x(t) to have an output y(t) which adheres to an envelope σ . • The output function y(t) can be derived as follows: = σ ⊗ = σ − + y ( t ) ( x )( t ) inf { ( t s ) x ( s )} ≤ ≤ 0 s t • Other analogies apply as well (commutativity and associativity), which allow to extend this analysis to large-scale systems. • There are significant differences, though! Min-Plus Calculus: Infimum vs. Minimum • Let S be nonempty subset of R . Definition [Infimum] inf( S ) = ( M s.t. s ≥ M ∀ s ∈ S ) inf( ø ) = + ∞ Definition [Minimum] min(S) = ( M ∈ S s.t. s ≥ M ∀ s ∈ S ) • Notation: ^ denotes infimum (e.g. a ^ b = min{ a , b }) 2

CPSC-663: Real-Time Systems Network Calculus The Dioid ( R ∪ {+ ∞ }, ^, +) • Conventional (“plus-times”) algebra operates on algebraic structure ( R ,+,*). • Min-plus algebra replaces operations: – “addition” becomes “computation of infimum” – “multiplication” becomes “addition” Resulting algebraic structure becomes ( R ∪ {+ ∞ }, ^, +) • • Example: – Conventional algebra: (3+4) * 5 = (3*5) + (4*5) = 15 + 20 – min-plus algebra: (3^4) + 5 = (3 + 5) ^ (4 + 5) = 8 ^ 9 = 8 Properties of ( R ∪ {+ ∞ }, ^, +) (Closure of ^) For all a , b ∈ R ∪ {+ ∞ }, a ^ b ∈ R ∪ {+ ∞ } • (Associativity of ^) For all a , b , c ∈ R ∪ {+ ∞ }, ( a ^ b )^ c = a ^( b ^ c ) • (Existence of a zero element of ^) There is some e ∈ R ∪ {+ ∞ }, such that • for all a ∈ R ∪ {+ ∞ }, a ^ e = a. (Idempotency of ^) For all a ∈ R ∪ {+ ∞ }, a ^ a = a. • (Commutativity of ^ ) For all a , b ∈ R ∪ {+ ∞ }, a ^ b = b ^ a. • (Closure of +) For all a , b ∈ R ∪ {+ ∞ }, a + b ∈ R ∪ {+ ∞ } . • (Zero element of ^ is absorbing for +) For all a ∈ R ∪ {+ ∞ }, • a + e = e = e + a. (Existence of neutral element for +) There is some u ∈ R ∪ {+ ∞ } such • that for all a ∈ R ∪ {+ ∞ }, a + u = a = u + a. (Distributivity of + with respect to ^) For all a , b , c ∈ R ∪ {+ ∞ }, • ( a ^ b ) + c = ( a + c ) ^ ( b + c ) = c + ( a ^ b ) 3

CPSC-663: Real-Time Systems Network Calculus Wide-Sense Increasing Functions Definition [wide-sense increasing] A function is wide-sense increasing iff f(s) ≤ f(t) for all s ≤ t . • Define G as the set of non-negative wide-sense increasing functions. • Define F as the set of non-negative wide-sense increasing functions with f(t) = 0 for t < 0. • Operations on functions: (f + g)(t) = f(t) + g(t) (f ^ g)(t) = f(t) ^ g(t) Wide-Sense Increasing Functions Peak rate function λ R : • “Rate” R >  Rt if t 0 λ =  ( t ) R  0 otherwise R Burst delay function δ T : • “Delay” T + ∞ >  if t T δ =  ( t ) T  0 otherwise T 4

CPSC-663: Real-Time Systems Network Calculus Wide-Sense Increasing Functions (2) Rate latency function β R,T : • “Rate” R , “Delay” T − >  R ( t T ) if t T β =  ( t ) R , T  0 otherwise R T Affine functions γ r,b : • “Rate” r , “Burst” b + >  rt b if t 0 γ =  ( t ) r r , b  0 otherwise b Wide-Sense Increasing Functions (3) Step function υ T : • >  1 if t T υ =  ( t ) 1 T  0 otherwise T • Staircase function u T, τ : “Interval” T , “Tolerance” τ  + τ   t  > if t 0   3 =    u T ( t ) T τ , 2   0 otherwise 1 τ T 5

CPSC-663: Real-Time Systems Network Calculus Wide-Sense Increasing Functions (4) • More general functions in F can be constructed by combining basic functions. • Example 1: r 1 >r 2 >...>r I and b 1 <b 2 <...<b I r 3 b 3 { } r 2 = γ ∧ γ ∧ ∧ γ = γ K b 2 f min 1 r , b r , b r , b r , b r 1 ≤ ≤ b 1 1 1 2 2 I I 1 i I i i • Example 2: = λ ∧ β + ∧ β + ∧ K f { RT } { 2 RT } 3 RT 2 R R , 2 T R , 4 T { } = β + 2 RT inf iRT R , 2 iT ≥ i 0 RT T 2T 3T 4T 5T Pseudo-Inverse of Wide-Sense Increasing Functions Definition [Pseudo-inverse] Let f be a function of F . The pseudo-inverse of f is the function f -1 (x) = inf{ t such that f(t) ≥ x } . • Examples: λ R λ 1/R -1 = δ T δ 0 ^ T -1 = β R,T γ 1/R,T -1 = γ r,b β 1/r,b -1 = 6

CPSC-663: Real-Time Systems Network Calculus Properties of Pseudo-Inverse • (Closure) ∈ = − − 1 1 f F and f ( 0 ) 0 • (Pseudo-inversion) We have that ≥ ⇒ ≤ − 1 f ( t ) x f ( x ) t < ⇒ ≥ − 1 f ( x ) t f ( t ) x • (Equivalent definition) = < − 1 f ( x ) sup{ t such that f ( t ) x } Min-Plus Convolution Integral of function f(t) ( f(t) = 0 for t ≤ 0 ) in conventional algebra: • ∫ t f ( s ) ds 0 • “Integral” for same function f(t) in min-plus algebra: { } inf f ( s ) ∈ ℜ ≤ ≤ s such that 0 s t • Convolution of two functions f(t) and g(t) that are zero for t < 0 in conventional algebra: ( ) ∫ t ⊗ = − + f g ( t ) f ( t s ) g ( s ) ds 0 Definition [Min-plus convolution] Let f and g be two functions of F . The min-plus convolution of f and g is the function { } ⊗ = − + ( f g )( t ) inf f ( t s ) g ( s ) ≤ ≤ 0 s t 7

CPSC-663: Real-Time Systems Network Calculus Min-Plus Convolution: Example 1 Compute ( γ r,b ⊗ β R,T )( t ) • Case 1: 0 ≤ t ≤ T • { } γ ⊗ β = γ − + β ( )( t ) inf ( t s ) ( s ) r , b R , T r , b R , T ≤ ≤ { } 0 s t = γ − + = γ + = inf ( t s ) 0 ( 0 ) 0 0 r , b r , b ≤ ≤ 0 s t Case 2: t > T γ ⊗ β ( )( t ) r , b R , T { } = γ − + β inf ( t s ) ( s ) r , b R , T ≤ ≤ 0 s t { } { } { } = γ − + β ∧ γ − + β ∧ γ − + β inf ( t s ) ( s ) inf ( t s ) ( s ) inf ( t s ) ( s ) ≤ ≤ r , b R , T ≤ ≤ r , b R , T = r , b R , T 0 s T T s t s t { } { } = + − + ∧ + − + − ∧ + − inf b r ( t s ) 0 inf b r ( t s ) R ( s T ) { 0 R ( t T )} ≤ ≤ { ≤ ≤ } 0 s T T s t { } = + − ∧ + − + − ∧ − { b r ( t T )} b rt RT inf ( R r ) s { R ( t T )} ≤ ≤ T s t = + − ∧ + − ∧ − { b r ( t T )} { b r ( t T )} { R ( t T )} = + − ∧ − { b r ( t T )} { R ( t T )} Min-Plus Convolution: Example 1 (2) ( γ r,b ⊗ β R,T )( t ) r b R T 8

CPSC-663: Real-Time Systems Network Calculus Min-Plus Convolution: Example 2 δ T ⊗ λ R = ? { } δ ⊗ λ = δ − + λ ( )( t ) inf ( t s ) ( s ) T R T R ≤ ≤ 0 s t { } ≤ ≤ δ ⊗ λ = δ − + λ Case 1 ( 0 t T ) : ( )( t ) inf ( t s ) ( s ) T R T R ≤ ≤ 0 s t { } = + λ = inf 0 ( s ) 0 R ≤ ≤ 0 s t > δ ⊗ λ = λ ⊗ δ Case 2 ( t T ) : ( )( t ) ( )( t ) T R R T { } = λ − + δ inf ( t s ) ( s ) R T ≤ ≤ 0 s T { } ∧ λ − + δ inf ( t s ) ( s ) R T < ≤ T s t { } { } = λ − + ∧ λ − + ∞ inf ( t s ) 0 inf ( t s ) R R ≤ ≤ < ≤ 0 s T T s t = λ − = β ( t T ) R R , T Models for Data Flow S R(t) R*(t) • Consider system S : receives input data, and delivers data after a variable delay. • R(t) is cumulative input function at time t . • R*(t) is cumulative output function at time t . Definition [Backlog] The backlog at time t is R(t)-R*(t) . Definition [Virtual Delay] The virtual delay at time t is d(t) = inf{ τ ≥ 0 : R(t) ≤ R*(t + τ ) } 9

CPSC-663: Real-Time Systems Network Calculus Virtual Delay d(t) = inf{ τ ≥ 0 : R(t) ≤ R*(t + τ ) } R(t) R*(t) t 1 t 2 • If input and output are continuous R*(t + d(t)) = R(t) (*) d(t) is smallest value satisfying (*) Arrival Curves Definition [Arrival Curve α(.) α(.) ] α(.) α(.) Given a wide-sense increasing function α (.) defined for t ≥ 0 (i.e. α (.) ∈ F ) we say that a flow R is constrained by α (.) iff for all s ≤ t : R(t) – R(s) ≤ α (t – s). “R has α(.) as arrival curve.” • “R is bounded by α (.).” • “R is α -smooth.” • • Note: α (.) is in the interval-domain. – – for all s ≥ 0 and I ≥ 0, R(s + I) – R(s) ≤ α (I) . 10