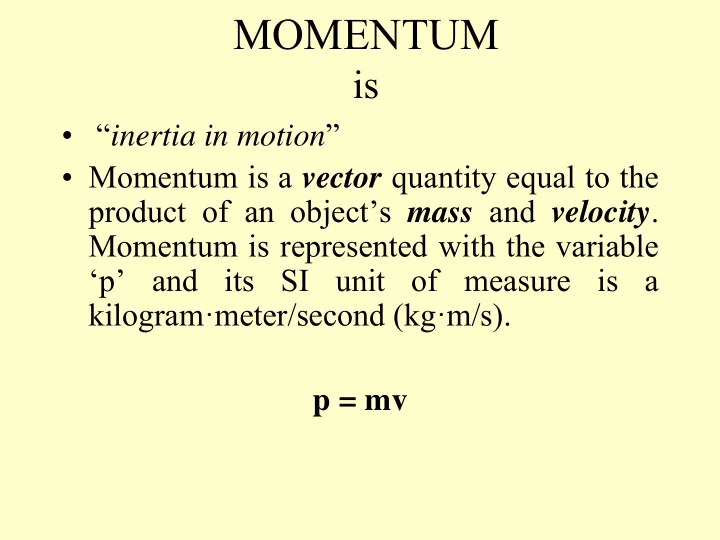
MOMENTUM is inertia in motion Momentum is a vector quantity - PowerPoint PPT Presentation

MOMENTUM is • “ inertia in motion ” • Momentum is a vector quantity equal to the product of an object’s mass and velocity . Momentum is represented with the variable ‘p’ and its SI unit of measure is a kilogram·meter/second (kg·m/s). p = mv

p = mv • momentum will always be in the same direction as the velocity • the momentum of that object will be directly proportional to the velocity • momentum will remain constant unless acted upon by an external force.

p = mv p x v m

Change in Momentum Δ p = p 2 – p 1 = mv 2 – mv 1 = m(v 2 – v 1 ) Δ p = m Δ v

Δ Momentum = Impulse • Newton’s second law of motion F = ma = m Δ v/ Δ t Or F Δ t = m Δ v • The left side of the equation is a quantity called impulse . • The impulse is the product of the average force and the time interval, measured in Newton·seconds (N·s), which are equal to (kg·m/s) The impulse placed on an object is ALWAYS equal to the Δ p experienced by the object.

A Summer Driving Experience or The tale of an unlucky Bug! (refer to handout provided by your teacher)

p = mv • momentum will always be in the same direction as the velocity • the momentum of that object will be directly proportional to the velocity • momentum will remain constant unless acted upon by an external force.

Δ Momentum = Impulse • Newton’s second law of motion F = ma = m Δ v/ Δ t Or F Δ t = m Δ v • The left side of the equation is a quantity called impulse . • The impulse is the product of the average force and the time interval, measured in Newton·seconds (N·s), which are equal to (kg·m/s) The impulse placed on an object is ALWAYS equal to the Δ p experienced by the object.

SOLVING Δ momentum/impulse problems Ask yourself this Question Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v. If no continue to evaluate. Equation F Δ t = m Δ v Or FAT – MAV

Impulse Change in Momentum an example problem Tiger Woods hits a 0.050 kg golf ball, giving it a speed of 75 m/s. What impulse does he impart on the ball?

Tiger Woods hits a 0.050 kg golf ball, giving it a speed of 75 m/s. What impulse does he impart on the ball? Ask yourself this Question Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v. If no continue to evaluate .

Impulse Change in Momentum Tiger Woods hits a 0.050 kg golf ball, giving it a speed of 75 m/s. What impulse does he impart on the ball? ∆ p F Δ t = m Δ v impulse

Impulse Change in Momentum Tiger Wood hits a 0.050 kg golf ball, giving it a speed of 75 m/s. What impulse does he impart on the ball? F Δ t = m Δ v m = 0.050 kg v 1 = 0 v 2 = 75 m/s

Impulse Change in Momentum Tiger Woods hits a 0.050 kg golf ball, giving It a speed of 75 m/s. What impulse does he impart on the ball? m = 0.050 kg v 1 = 0 v 2 = 75 m/s F Δ t = 0.050kgx(75m/s-0)

Impulse Change in Momentum F Δ t = 0.050kgx(75m/s-0) Since the impulse impressed on an object ALWAYS equals the change in momentum it experiences Impulse = 3.8 kgxm/s or (Nxs)

Another example Wayne hits a stationary 0.12kg hockey puck with a force that lasts 0.010 s and makes the puck shoot across the ice at 20.0 m/s. What force was applied to the puck?

Another example Wayne hits a stationary 0.12kg hockey puck with a force that lasts 0.010 s and makes the puck shoot across the ice at 20.0 m/s. What force was applied to the puck? Ask yourself this Question Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v. If no continue to evaluate.

Another example Wayne hits a stationary 0.12kg hockey puck with a force that lasts 0.010 s and makes the puck shoot across the ice at 20.0 m/s. What force was applied to the puck? F Δ t = m Δ v m = 0.12 kg v 1 = 0 v 2 = 20.0 m/s t 1 = 0 t 2 = 0.010 s

Another example Wayne hits a stationary 0.12kg hockey puck with a force that lasts 0.010 s and makes the puck shoot across the ice at 20.0 m/s. What force was applied to the puck? m = 0.12 kg F Δ t = m Δ v v 1 = 0 F = (m Δ v)/ Δ t F = 0.12kgx(20.0m/s-0)/(0.010 s – 0) v 2 = 20.0 m/s t 1 = 0 t 2 = 0.010 s

Another example F Δ t = m Δ v F = 0.12kgx(20.0m/s-0)/(0.010 s – 0) F = 240 N

Still another example A 0.60 kg tennis ball traveling at 10 m/s is returned. It leaves the racket with a speed of 36 m/s in the opposite direction. What is the Δ p of the ball? If the ball is in contact with the racket 0.020 s what is the force impressed on the ball by the racket? Ask yourself this Question Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v. If no continue to evaluate.

Still another example A 0.60 kg tennis ball traveling at 10 m/s is returned. It leaves the racket with a speed of 36 m/s in the opposite direction. What is the Δ p of the ball? If the ball is in contact with the racket 0.020 s what is the force impressed on the ball by the racket? F Δ t = m Δ v m = 0.060 kg v 1 = -10 m/s ∆ p v 2 = 36 m/s t 1 = 0 t 2 = 0.020 s

Still another example A 0.60 kg tennis ball traveling at 10 m/s is returned. It leaves the racket with a speed of 36 m/s in the opposite direction. What is the Δ p of the ball? If the ball is in contact with the racket 0.020 s what is the force impressed on the ball by the racket? m = 0.60 kg ∆ p = m ∆ v v 1 = -10 m/s = 0.60kgx(36-(-10))m/s v 2 = 36 m/s = 2.8 kgxm/s t 1 = 0 t 2 = 0.020 s

Still another example A 0.60 kg tennis ball traveling at 10 m/s is returned. It leaves the racket with a speed of 36 m/s in the opposite direction. What is the Δ p of the ball? If the ball is in contact with the racket 0.020 s what is the force impressed on the ball by the racket? m = 0.60 kg F ∆ t = m ∆ v v 1 = -10 m/s F = (m ∆ v)/ ∆ t v 2 = 36 m/s = 0.60kgx(36-(-10))m/s/(0.020s – 0) t 1 = 0 F = 140 N t 2 = 0.020 s

Δ Momentum = Impulse Problems Your Teacher will now give you a problem packet and assign Δ p = Impulse problems on which you will work.

LAW OF CONSERVATION OF MOMENTUM The momentum of a system before an event or incident is equal to the momentum of the system after the event or incident if the system is closed. A closed system is a system that doesn’t have objects entering or leaving, and an isolated system is one without external forces acting on it.

Types of events Inelastic: Objects are tangled or stuck together after the event. Objects become deformed and heat & sound are generated during the event. Elastic: Objects are apart after the event. Objects are not deformed and no heat or sound are generated during the event.

SOLVING conservation of momentum problems Remember these 5 steps 1) Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v If no continue to # 2. 2) Identify the objects. 3) Identify the incident or event. 4) Are the objects together or apart AFTER the event? 5) If the objects are together AFTER the event this is an inelastic event. - If the objects are apart AFTER the event this is an elastic event.

Inelastic Events In a closed system when the objects are together AFTER the event the event is inelastic. Momentum of the system before the event = Momentum of the system after the event = p a p b m 1 v 1 + m 2 v 2 = (m 1 + m 2 )v a

Inelastic Events In a closed system when the objects are together AFTER the event the event is inelastic. m 1 – mass of the first object m 2 - mass of the second object v 1 - velocity of the first object before the event v 2 - velocity of the second object before the event v a - velocity of the combined objects after the event

Family fun at Happy Wheels or Adventures of an ancient Roller Derby Queen

Inelastic Events Granny (aka Roller Ruth) whizzes around the rink at 3m/s and confronts Ambrose scared motionless in front of her. Thinking quickly she picks up Ambrose and continues on. What is their velocity after averting this near disaster? 1) Does the problem mention Δ p, impulse, time, or force? If yes it’s a F Δ t = m Δ v If no continue to # 2. 2) Identify the objects. 3) Identify the incident or event. 4) Are the objects together or apart AFTER the event? 5) If the objects are together AFTER the event this is an inelastic event. - If the objects are apart AFTER the event this is an elastic event.

Inelastic Events Granny (aka Roller Ruth) whizzes around the rink at 3m/s and confronts Ambrose scared motionless in front of her. Thinking quickly she picks up Ambrose and continues on. What is their velocity after averting this near disaster? = p a p b m 1 v 1 + m 2 v 2 = (m 1 + m 2 )v a m 1 = 80kg m 2 = 40kg v 1 = 3m/s V 2 = 0 v a - ?

Inelastic Events Granny (aka Roller Ruth) whizzes around the rink at 3m/s and confronts Ambrose scared motionless in front of her. Thinking quickly she picks up Ambrose and continues on. What is their velocity after averting this near disaster? = p a p b m 1 v 1 + m 2 v 2 = (m 1 + m 2 )v a m 1 = 80kg 80kgx3m/s + 40 kgx0 = (80+40)kgxv a m 2 = 40kg 240kgxm/s = 120kgxv a v 1 = 3m/s 120kg 120 kg v 2 = 3m/s (240kgxm/s)/120kg = v a v a - ? 2m/s = v a