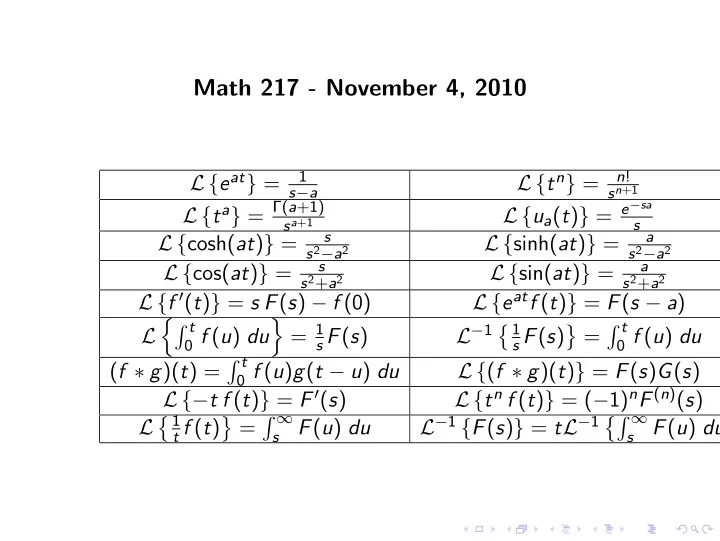
Math 217 - November 4, 2010 1 n ! L { e at } = L { t n } = s n +1 s - PowerPoint PPT Presentation

Math 217 - November 4, 2010 1 n ! L { e at } = L { t n } = s n +1 s − a L { t a } = Γ( a +1) L { u a ( t ) } = e − sa s a +1 s L { cosh( at ) } = s L { sinh( at ) } = a s 2 − a 2 s 2 − a 2 L { cos( at ) } = s L { sin( at ) } = a s 2 + a 2 s 2 + a 2 L { f ′ ( t ) } = s F ( s ) − f (0) L { e at f ( t ) } = F ( s − a ) L − 1 � 1 �� t � t � = 1 � L 0 f ( u ) du s F ( s ) s F ( s ) = 0 f ( u ) du � t ( f ∗ g )( t ) = 0 f ( u ) g ( t − u ) du L { ( f ∗ g )( t ) } = F ( s ) G ( s ) L { t n f ( t ) } = ( − 1) n F ( n ) ( s ) L {− t f ( t ) } = F ′ ( s ) � 1 � ∞ L − 1 { F ( s ) } = t L − 1 �� ∞ � L t f ( t ) = F ( u ) du F ( u ) du s s

1. Set up the partial fractions for the following fractions. (If you run out of things to be doing, work on the solutions). (a) s 2 ( s − 1)( s − 2)( s − 3) = (b) s 2 ( s − 1) 3 ( s − 2)( s − 3) = (c) s 2 ( s 2 + 1)( s 2 + 2) 2 ( s − 3) =

1. Set up the partial fractions for the following fractions. (If you run out of things to be doing, work on the solutions). (a) s 2 A B C ( s − 1)( s − 2)( s − 3) = s − 1 + s − 2 + s − 3 Solution: A = 1 / 2 , B = − 4 , C = 9 / 2. (b) s 2 A B C D ( s − 1) 3 ( s − 2)( s − 3) = s − 1 + ( s − 1) 2 + ( s − 1) 3 + s − 2 + Solution: A = 23 / 8 , B = 7 / 4 , C = 1 / 2 , D = 4 , E = 9 / 8. (c) s 2 ( s 2 + 1)( s 2 + 2) 2 ( s − 3) = As + B s 2 + 1 + Cs + D s 2 + 2 + Es + F G ( s 2 + 2) 2 + s − Solution: 2 s + 6 19 s + 57 s + 3 9 11 ( s 2 + 2) − 100 ( s 2 + 1) + 10 ( s 2 + 1) 2 + 1100 ( s − 3)

2. Find the inverse laplace transform for the functions in the previous problem. (Even if you did not solve the partial fractions, you can still do this problem, just leave the constants as A , B , C , etc.)

2. Find the inverse laplace transform for the functions in the previous problem. (Even if you did not solve the partial fractions, you can still do this problem, just leave the constants as A , B , C , etc.) Solution: Here are a few basics: � 1 � L − 1 = e at s − a � 1 � L − 1 = te at ( s − a ) 2 � 1 � =1 L − 1 2 t 2 e at ( s − a ) 3 � 1 � =1 L − 1 a sin( at ) s 2 + a 2 � � s L − 1 = cos( at ) s 2 + a 2 � 1 � L − 1 =???? ( s 2 + a 2 ) 2 � s � L − 1 =???? ( s 2 + a 2 ) 2

Lecture Problems 3. Solve the system using the Laplace Transform x ′ = x − y − 2 x (0) = − 1 y ′ =2 x − y + 1 y (0) = 2 (a) What are the transformed equations? (b) Set up a nice linear system of equations in X and Y . (c) Solve your system for X and Y . (d) Do partial fractions on X and Y . (e) Find the inverse Laplace transform (solve for x and y ).

Lecture Problems 3. Solve the system using the Laplace Transform x ′ = x − y − 2 x (0) = − 1 y ′ =2 x − y + 1 y (0) = 2 (a) What are the transformed equations? sX + 1 = X − Y − 2 s sY − 2 =2 X − Y + 1 s (b) Set up a nice linear system of equations in X and Y . ( s − 1) X + Y = − 2 − 2 s − 2 X + ( s + 1) Y = 2 + 1 s (c) Solve your system for X and Y . X = − s 2 + 5 x + 3 s ( s 2 + 1) Y = 2 s 2 − 3 s − 5

4. Find the inverse transforms (transformation of integrals) (a) � 1 � L − 1 = s − 3 (b) � � 1 L − 1 = s ( s − 3) (c) � 1 � L − 1 = s 2 ( s − 3) (d) � 1 � L − 1 = s 3 ( s − 3)

4. Find the inverse transforms (transformation of integrals) (a) � 1 � L − 1 = e 3 t s − 3 (b) � t � � 1 e 3 u du = 1 3( e 3 t − 1) L − 1 = s ( s − 3) 0 (c) � t 3( e 3 u − 1) du = e 3 t � 1 � 1 9 − t 3 − 1 L − 1 = s 2 ( s − 3) 9 0 (d) = e 3 t 27 − t 2 � 1 � 9 − 1 6 − t L − 1 s 3 ( s − 3) 27