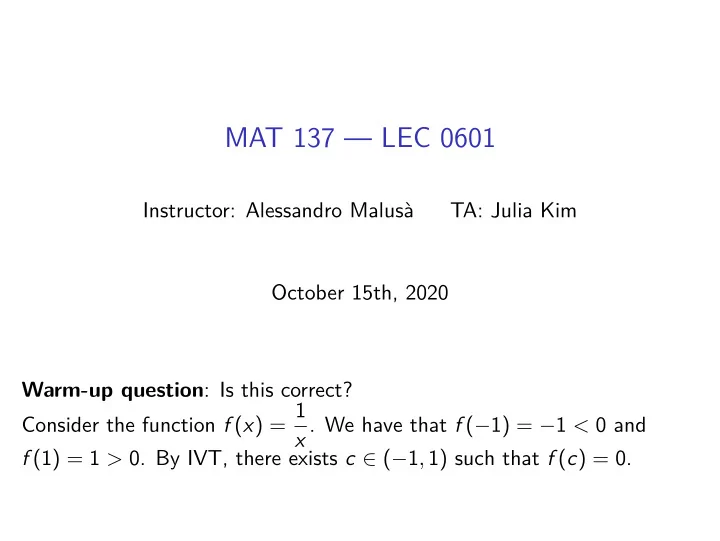
MAT 137 LEC 0601 Instructor: Alessandro Malus TA: Julia Kim - PowerPoint PPT Presentation

MAT 137 — LEC 0601 Instructor: Alessandro Malusà TA: Julia Kim October 15th, 2020 Warm-up question : Is this correct? Consider the function f ( x ) = 1 x . We have that f ( − 1) = − 1 < 0 and f (1) = 1 > 0. By IVT, there exists c ∈ ( − 1 , 1) such that f ( c ) = 0.

Existence of solutions Prove that the equation x 4 − 2 x = 100 has at least two solutions.

Can this be proven? (Use IVT) 1 Prove that the hour hand and the minute hand of a clock form an angle of exactly 23 degrees at least once a day.

Can this be proven? (Use IVT) 1 Prove that the hour hand and the minute hand of a clock form an angle of exactly 23 degrees at least once a day. 2 During a Raptors basketball game, at half time the Raptors have 52 points. Prove that at some point they had exactly 26 points.

Extrema In each of the following cases, does the function f have a maximum and a minimum on the interval I ? 1 f ( x ) = x 2 , I = ( − 1 , 1). 2 f ( x ) = ( e x + 2) sin x − cos x + 3, I = [2 , 6] x 3 f ( x ) = ( e x + 2) sin x − cos x + 3, I = (0 , 5] x

Before next class... • Watch videos 3.1, 3.2, and 3.3. • Download the next class’s slides (no need to look at them!)