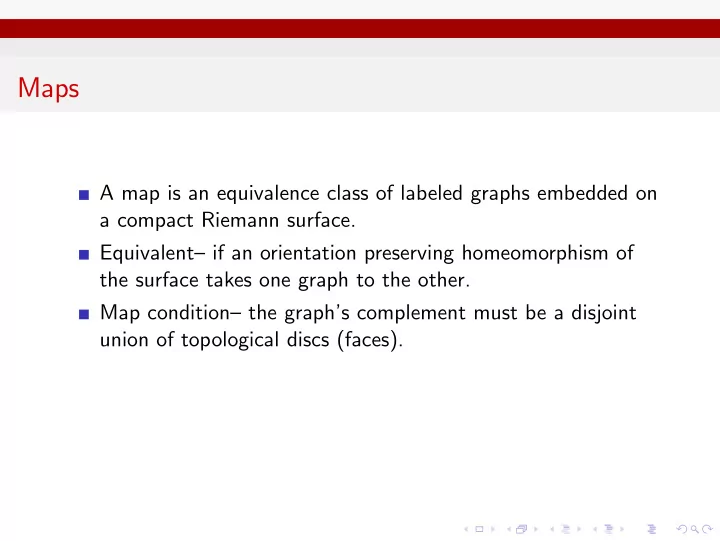
Maps A map is an equivalence class of labeled graphs embedded on a - PowerPoint PPT Presentation

Maps A map is an equivalence class of labeled graphs embedded on a compact Riemann surface. Equivalent– if an orientation preserving homeomorphism of the surface takes one graph to the other. Map condition– the graph’s complement must be a disjoint union of topological discs (faces).

Maps A map is an equivalence class of labeled graphs embedded on a compact Riemann surface. Equivalent– if an orientation preserving homeomorphism of the surface takes one graph to the other. Map condition– the graph’s complement must be a disjoint union of topological discs (faces). Labels– The vertices have distinct names We choose a function assigning to each vertex one of its incident edges

Edges cannot intersect No Yes 1 1 2

Faces must be discs Yes 1

Faces must be discs Yes 1 1 No

Dehn twist These are the same map 1 1

How can log Z n know about maps???

GUE covariances Gaussian Unitary Ensemble: dP n ( M ) = 1 2 Tr M 2 dM e − 1 Z n E [ M ij M kl ] = δ i = l δ j = k

Wick’s lemma f 1 , . . . , f 2 m are linear functionals on R n × n . � E [ f 1 . . . f 2 m ] = E [ f w (1) f w (2) ] . . . E [ f w (2 m − 1) f w (2 m ) ] w ∈ Wick pairings of 1 , . . . , 2 m An example of a Wick pairing of 1 , . . . , 8 is {{ 1 , 6 } , { 2 , 5 } , { 3 , 4 } , { 7 , 8 }} .

Matrix Integrals and combinatorics Here is a very brief hand wave at the connection between matrix integrals in map combinatorics.   p n �� Tr M 4 � p � � � = E E M i q , j q M j q , k q M k q , l q M l q , i q   q =1 i q , j q , k q , l q =1 n � � Product of quadratic � � = expectations given by w and index variables w ∈ Wick pairings i 1 , j 1 , k 1 ,... , j p , k p , l p =1 of 1,. . . ,4p � n Faces = 4-valent fatgraphs on p vertices

A Wick pairing and the corresponding map The Wick pairing ( i 1 1 , j 1 3 ) , ( i 1 3 , k 2 3 ) , ( k 1 3 , k 3 3 ) , ( i 2 3 , i 3 3 ) , ( j 2 3 , j 3 3 ) corresponds to a fatgraph and a map. 1 1 i 1 1 j 1 3 1 3 i 1 k 1 3 3 k k 2 3 3 3 3 2 3 3 i j j i 2 3 2 3 3 3 3 3

A Wick pairing and the corresponding map The Wick pairing ( i 1 1 , j 1 3 ) , ( i 1 3 , k 2 3 ) , ( k 1 3 , k 3 3 ) , ( i 2 3 , i 3 3 ) , ( j 2 3 , j 3 3 ) corresponds to a fatgraph and a map. 1 1 i 1 1 j 1 3 i 1 1 1 1 1 3 i 1 k 1 3 3 i 1 1 3 3 k k 2 3 3 3 3 2 i 2 3 3 3 i j j i 2 3 2 3 3 3 3 3 3 3 2 3 3 i 3 3