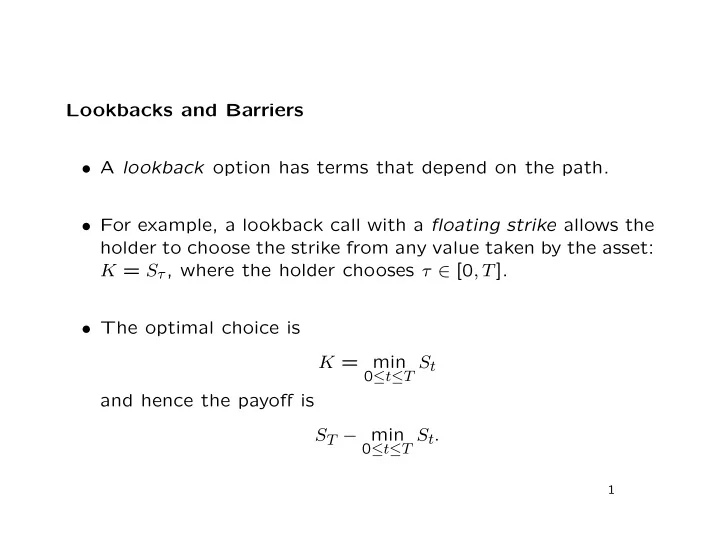
Lookbacks and Barriers A lookback option has terms that depend on - PowerPoint PPT Presentation

Lookbacks and Barriers • A lookback option has terms that depend on the path. • For example, a lookback call with a floating strike allows the holder to choose the strike from any value taken by the asset: K = S τ , where the holder chooses τ ∈ [0 , T ]. • The optimal choice is K = min 0 ≤ t ≤ T S t and hence the payoff is S T − min 0 ≤ t ≤ T S t . 1

• A lookback call with a fixed strike K allows the holder to choose the time at which the option is exercised: the payout is ( S τ − K ) + , where the holder chooses τ ∈ [0 , T ]. • The optimal choice is τ = arg max S t and hence the payoff is � � max 0 ≤ t ≤ T S t − K . + 2

• The price of a lookback option is, as always, the expected value of the discounted payoff under the risk-neutral distri- bution. – This requires the joint distribution of S T and either min 0 ≤ t ≤ T S t or max 0 ≤ t ≤ T S t ; – use the reflection principle . 3

• A barrier option is an option (European or American) with the additional condition that it is worthless if the path crosses ( knock-out ) or fails to cross ( knock-in ) a barrier level. • Knock-in: – up-and-in; – down-and-in. • Knock-out: – up-and-out; – down-and-out. 4

• For a European barrier option, the payoff is a function of S T and either min 0 ≤ t ≤ T S t or max 0 ≤ t ≤ T S t . • Calculating the expected value similarly requires their joint distribution. • More complex barrier options may have barriers that are ef- fective in only the early part of the option’s life, or in only the later part. • One exchange-traded barrier put had a knock-out upper bar- rier and automatic exercise at a lower barrier. 5

Asian options • Payoff depends on the average price. • E.g. Asian call with fixed strike K : payoff is � T � � 1 C T = 0 S t dt − K T + • C T is F T -measurable, so the value at time t is again e − r ( T − t ) C T � E Q � � � F t � • But note that this is not a function of only S t . 6

• Asian call with floating strike: payoff is � T � � S T − 1 C T = 0 S t dt T + • Again, the value at time t is � E Q � e − r ( T − t ) C T � � F t � and again this is not a function of only S t . 7