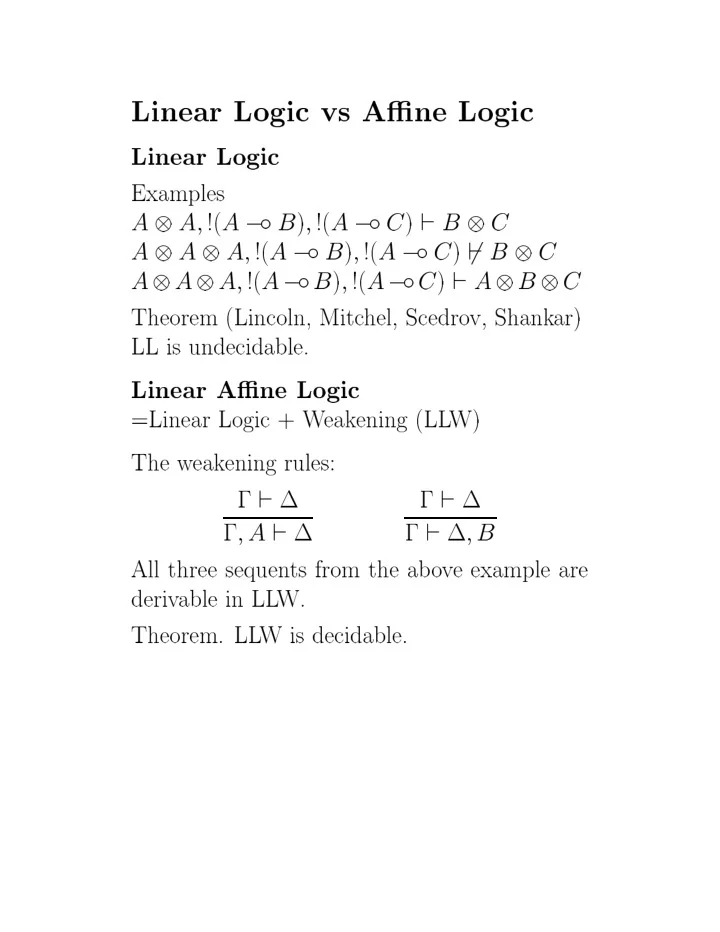
Linear Logi vs Ane Logi Linear Logi Examples A A; !( A - PDF document

Linear Logi vs AÆne Logi Linear Logi Examples A � A; !( A � Æ B ) ; !( A � Æ C ) ` B � C A � A � A; !( A � Æ B ) ; !( A � Æ C ) 6` B � C A � A � A; !( A � Æ B ) ; !( A � Æ C ) ` A � B � C Theorem (Lin oln, Mit hel, S edro v, Shank ar) LL is unde idable. Linear AÆne Logi =Linear Logi + W eak ening (LL W) The w eak ening rules: � ` � � ` � � ; ` � � ` � ; A B All three sequen ts from the ab o v e example are deriv able in LL W. Theorem. LL W is de idable.

P etri Nets Def. A P etri net is a pair ( P ) where ; T is a �nite set of pla es P P P T � ( ! � ! ) is a �nite set of transa tions Def. A marking of a P etri net is a v e tor M 2 P ! Def. A transa tion = ( u; ) is �reable at if t v M 8 p : � P :M u p p Def. A transa tion = ( u; ) �res form to t v M 0 if M 0 = � + M M u v 0 Def. A state is rea hable from if there is M M 0 a sequen e = = su h M M ; M ; : : : ; M M 0 1 n that is obtained from after the �ring M M i +1 i of some transa tion. Theorem (Ma y er 1981, Kosara ju 1982). The rea habilit y problem is de idable.

Horn fragmen t of LL is a tensor pro du t of literals A simple pr o du t (e.g. � � ). p p q A Horn impli ation is an impli ation of the form: A � Æ B , where A and B are simple pro d- u ts. A Horn se quent is a sequen t of the form !� ` W ; Z where and are simple pro du ts and � is a W Z set of Horn impli ations.

En o ding P etri nets in the Horn frag- men t Ea h pla e orresp onds to a literal. V e tors (markings) orresp onds to simple pro d- u ts. T ransa tion orresp onds to Horn impli ations. P etri net R orresp onds to the set of Horn im- pli ations � R 0 Theorem. is rea hable from in a P etri M M 0 net i� the sequen t !� ` is deriv able R M ; M R in LL. 0 Theorem. The sequen t !� ` is deriv- M ; M R 00 0 able in LL W i� there is M � M , su h that 00 M is rea hable from M in a P etri net R .

Normal fragmen t � -Horn is an impli ation of the A impli ation form: � Æ ( B � ), where A , and are A C B C simple pro du ts. A simple disjun tion is a disjun tion of the form: B C , where B and C are simple pro d- . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . u ts. is a sequen t of the form A normal se quent !� ` ? � ; W ; where is a simple pro du t, � is a m ultiset W of Horn impli ations, � -Horn impli ations and simple disjun tions, and � is a m ultiset of sim- ple pro du ts.

Example = � bl d � paper � Æ L hal k a k boar pr esentation 1 = ides � pr � paper � Æ L sl oj e tor pr esentation 2 = � L bl a k boar d pr oj e tor 3 paper � sl ides � hal k ; ! L ; ! L ; ! L ` pr esentation 1 2 3 0 = L bl a k boar d pr oj e tor . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3 . . . . . . . . . . . . . . . . . . . . . . . . . . . 0 � � � ! L ! L ! L ` paper paper sl ides hal k ; ; ; 1 2 3 pr esentation; pr esentation 0 � � � ! L ! L ! L ` paper paper sl ides hal k ; ; ; 1 2 3 ? pr esentation

Let � = ( W !� ` ?�). ; Game A � ~ 1. Initially , all v e tors from � are written on the bla kb oard. 2. W e ma y write new v e tors with natur al o- or dinates b y the follo wing rules: ~ (a) If � Æ 2 � and a v e tor + has X Y a Y b een already written, then w e ma y write ~ + . a X ~ (b) If X � Æ ( Y � Y ) 2 � and v e tors a + Y 1 2 1 ~ and + ha v e b een already written, a Y 2 ~ then w e ma y write a + X . ~ ( ) If Y Y 2 � and v e tors a + Y and . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1 . . . . . . . 2 1 1 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . ~ a + Y ha v e b een already written, then 2 2 w e ma y write a + a . 1 2 ~ 3. The aim of the game is to obtain . W Game B � 4. If a v e tor has b een written and � a a then w e ma y write .

Theorem. (Computational in terpretation) 1. The normal sequen t � is deriv able in LL i� it is p ossible to rea h the aim in the game A � 2. The normal sequen t � is deriv able in LL W i� it is p ossible to rea h the aim in the game B � Pro of. It is easy to pro v e it b y indu tion on deriv ation and on the n um b er of steps that w e needed to a hiev e the aim in the games.

Redu tion to the normal fragmen t Theorem. F or an y sequen t � one an e�e tiv ely onstru t a normal sequen t � su h that ` � ( ) ` �; LLW LLW ` � ( ) ` � : LL LL Lemma. Let � ` � b e a sequen t. Let x b e an atom in the sequen t. Let A b e an arbitrary form ula. 0 0 Let � = �[ x := A ℄ and � = �[ x := A ℄. 0 0 Then � ` � is deriv able i� !( x � Æ A ) ; !( A � Æ x ) ; � ` � is deriv able.

The de idabilit y of LL W Theorem. The problem whether w e an rea h the aim in the game is de idable. B � Lemma. An y set of pairwise in omparable v e - n tors from is �nite. ! Def. Let � b e a set of normal sequen ts. Let n A � . W e sa y that A is �- losed when ! 1. If � Æ 2 � then X Y ~ ~ n 8 a 2 + 2 A ) + 2 A : ! a Y a X 2. If � Æ ( Y � ) 2 � then X Y 1 2 ~ ~ ~ n 8 a 2 a + 2 A ; a + 2 A ) a + 2 A : ! Y Y X 1 2 3. If Y Y 2 � then . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1 . . . . . . . 2 . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . ~ ~ n 8 a ; a 2 ! a + Y 2 A ; a + Y 2 A ) a + a 2 A : 1 2 1 1 2 2 1 2 W e sa y that A is - losed when w 8 a 2 A 8 � 2 A : a

The set of all rea hable v e tors in the game B � is �- losed and - losed. w Lemma. It is p ossible to rea h the aim in the game if and only if the follo wing holds: B � n F or an y A � ! if A is �- losed and ~ ~ w - losed and � � A , then W 2 A . Lemma. If A is losed under the w eak ening then for some �nite B A = K ; [ z z 2B where = f x j � g . K x z z Lemma. The prop ert y of the set A = [ K z 2B z to b e �- lose is de idable. Corollary . The set of deriv able normal sequen ts is o en umerable.