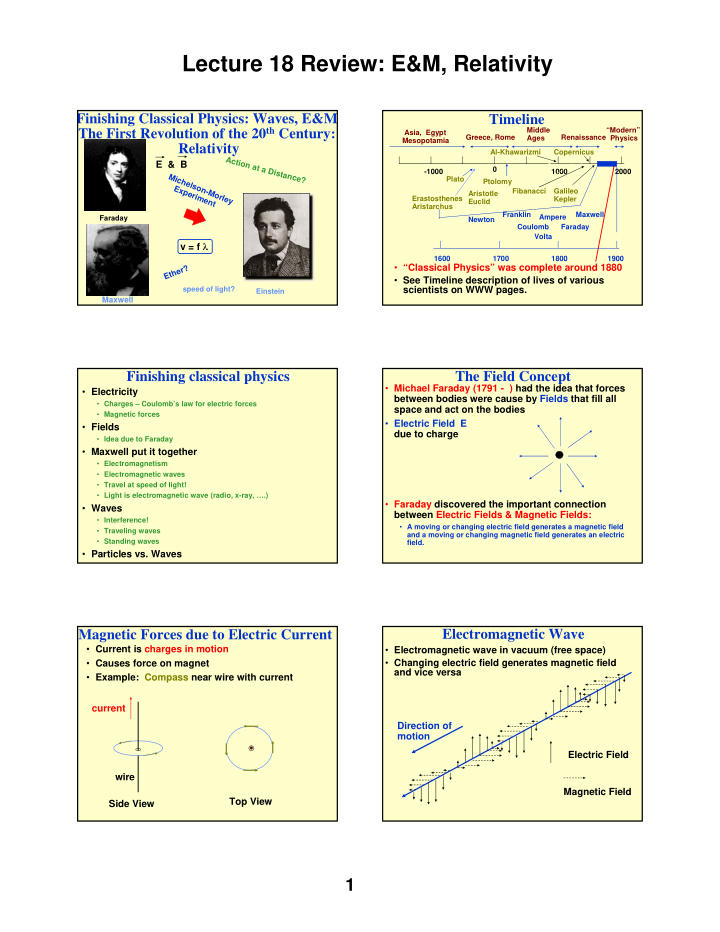
Lecture 18 Review: E&M, Relativity Finishing Classical Physics: - PDF document

Lecture 18 Review: E&M, Relativity Finishing Classical Physics: Waves, E&M Timeline The First Revolution of the 20 th Century: Middle “Modern” Asia, Egypt Greece, Rome Ages Renaissance Physics Mesopotamia Relativity Al-Khawarizmi Copernicus A c t i E & B o n a t a 0 D i -1000 1000 2000 s t a n Michelson-Morley c e ? Plato Ptolomy Experiment Fibanacci Galileo Aristotle Erastosthenes Kepler Euclid Aristarchus Franklin Maxwell Ampere Faraday Newton Coulomb Faraday Volta v = f λ 1600 1700 1800 1900 • “Classical Physics” was complete around 1880 Ether? • See Timeline description of lives of various scientists on WWW pages. speed of light? Einstein Maxwell Finishing classical physics The Field Concept • Michael Faraday (1791 - ) had the idea that forces • Electricity between bodies were cause by Fields that fill all • Charges – Coulomb’s law for electric forces space and act on the bodies • Magnetic forces • Electric Field E • Fields due to charge • Idea due to Faraday • Maxwell put it together • Electromagnetism • Electromagnetic waves • Travel at speed of light! • Light is electromagnetic wave (radio, x-ray, ….) • Faraday discovered the important connection • Waves between Electric Fields & Magnetic Fields: • Interference! • A moving or changing electric field generates a magnetic field • Traveling waves and a moving or changing magnetic field generates an electric • Standing waves field. • Particles vs. Waves Magnetic Forces due to Electric Current Electromagnetic Wave • Current is charges in motion • Electromagnetic wave in vacuum (free space) • Changing electric field generates magnetic field • Causes force on magnet and vice versa • Example: Compass near wire with current current Direction of motion Electric Field wire Magnetic Field Top View Side View 1

Lecture 18 Review: E&M, Relativity Conditions for Interference of Waves Waves • If any type of wave is emitted from two sources “in phase”, i.e, the highs and lows are emitted • Important example: Periodic waves simultaneously • Repeated identical waves: • Constructive interference occurs λ = wavelength = distance it λ if D 1 - D 2 = n λ takes for pattern to repeat • Destructive interference occurs D 1 if D 1 - D 2 = (n + 1/2) λ f = frequency = number of times a given point reaches D 2 maximum each second f = 1/T, T = period = time between maxima λ λ v = λ/ T v = velocity of wave v = f λ Amplitude = max to min variation The range of electromagnetic waves Another view of interference • All waves have velocity given by v = f λ Light is a wave! • Electromagnetic waves have velocity v = c in vacuum • Therefore c = f λ or f = c/ λ or λ = c/ f λ (meters) 10 -12 10 6 1 10 -6 Micro Gamma rays radio TV, FM IR UV X rays waves 10 6 10 15 10 24 F (hertz = cycles/sec) Visible light Standing Waves Summary of Classical Physics • Physics as it stood near the end of the 19th Century • Waves with boundary conditions.. e.g. hold both ends of a string fixed as in a guitar. • Fundamental concepts: • velocity of any wave produced (by plucking the string) is • Time flows the same everywhere for all observers determined by the medium.. in this case the type of the string. • Space is described by 3 dimensions (Euclidean Geometry) • For a fixed length of string, only waves with certain • Mass is never created nor destroyed (conserved) wavelengths can be standing waves... namely those • Charge (plus and minus) total is conserved wavelengths which have zeroes at the ends of the string. • Energy changes form but is conserved • Therefore only certain frequencies will be heard.. namely those which correspond to the definite wavelengths via f = v / λ . • Momentum is conserved • Fundamental Objects and Laws: • Particles have mass and move according to Newton’s laws L = λ / 2 fundamental: lowest frequency L • Force originates in interactions between particles of matter • baseballs, rockets, ….. • Waves are moving patterns in a medium - e.g. light is described by Maxwell’s laws L = λ first harmonic: higher frequency • Sound, Light, ….. • Waves have interference -- Particles do not 2

Lecture 18 Review: E&M, Relativity Summary of Classical Physics Michelson-Morley experiment Start the Revolutions of Modern Physics • Case School of Applied Science (Now Case Western Reserve) in Cleveland (1887) Newton’s Laws of motion • Speed of light the same in all directions Laws for forces, e.g., gravity independent of the motion of the earth! Motion through the Ether? Maxwell’s Laws of E & M Classical Physics in 1880’s mirror Is Physics finished? half-silvered Only details left? mirror mirror light Michelson-Morley Experiment Albert Einstein (1879-1955) Special Relativity I • Postulate: • Born German, went to university in Switzerland, became naturalized Swiss citizen. The speed of light is the same to all observers • 1902: Job at patent office in Bern Does physics on the side. • You can never catch light! • 1905: 5 Five seminal papers • molecular dimensions • Leads to change in defintion of length and time • Brownian motion • Photoelectric effect (Nobel prize) • Relativity • An object moving with respect to an observer • E = mc 2 appears to be shorter to that observer • 1909: Zurich prof. 1913: Berlin chair in Physics • A clock moving with respect to an observer appears to be running slow to that observer 1916: General relativity The Wedding of Time and Space Special Relativity • Postulate: The speed of light is the same universal constant for all observers • Summary in Form of Equations T improper = γ T proper L parallel (moving) = L parallel (rest) / γ L perpendicular (moving) = L perpendicular (rest) m (moving) = γ m (rest) E = m c 2 Moving clocks appear to run slow. 1 γ = Moving objects appear to shrink along line of > 1 sqrt(1 - v 2 /c 2 ) motion and appear distorted. Order of events can differ for different observers. 3

Lecture 18 Review: E&M, Relativity General Relativity Einstein’s “Happiest Idea” Equivalence Principle • Equivalence Principle: The effect of gravity is exactly the same as a=g acceleration! • Consequence: ≡ a=g In an accelerating reference frame clocks run at different rates depending on position The same applies to gravitational field Clocks near the surface of the earth run slower than ones far from the surface General Relativity leads to Does Gravity Also Affect Clocks? “Curved Space-time” • We have just seen that the equivalence principle predicts that light bends in a gravitational field. • “Inertial motion” is not along “straight lines” but What are the consequences of the equivalence along the “shortest path” principle for time? Example of motion on surface • The observer at the bottom observes that the of earth: shortest path is clock at the top appears to run faster than his clock curved geodesic C D clock( at the bottom) • Reason: Time is defined using the speed of light. Triangle with sum of angles > 180 degrees In the time it takes the pulses to travel to the L Newton described free fall accelerated bottom clock, the rocket has increased its velocity a motion as caused by “Force of Gravity” β = v/c = aL/c 2 by an amount: v = at = aL/c a=g Einstein’s generality has no “force of clock gravity! Accelerated free fall is inertial f = f 0 / (1 - β ) motion in curved space-time! Impact of Revolution of Relativity Twin “Paradox” • Enormous impact upon society and intellectual Rocket Twin is Younger! thought in 20 th Century • Dominated by Einstein’s ideas • The key is: The Rocket Twin accelerated while the Earth Twin didn’t! The acceleration distinguishes • Speed of light c is a fundamental constant of nature the two twins and prevents us from applying the • Postulates, “Gedanken Experiments” principle of relativity. • Equivalence Principle • The Calculation: Identify 3 Events: • Total revision of our concepts of space and time • space and time unified to form space-time • Mass and Energy unified - E = mc 2 1 v v • Enormous practical consequence • Matter, Space-Time, Gravity unified 2 • Essential to understand the universe - more later v 3 • For everyday problems, Newton’s laws are still completely adequate! • The Rocket Twin measures proper time for both time intervals: 1-2 and 2-3. Therefore the Rocket Twin measures the smallest time interval from 1-3! 4