= kC A C B . dt dt Elementary reaction is one place where - PowerPoint PPT Presentation

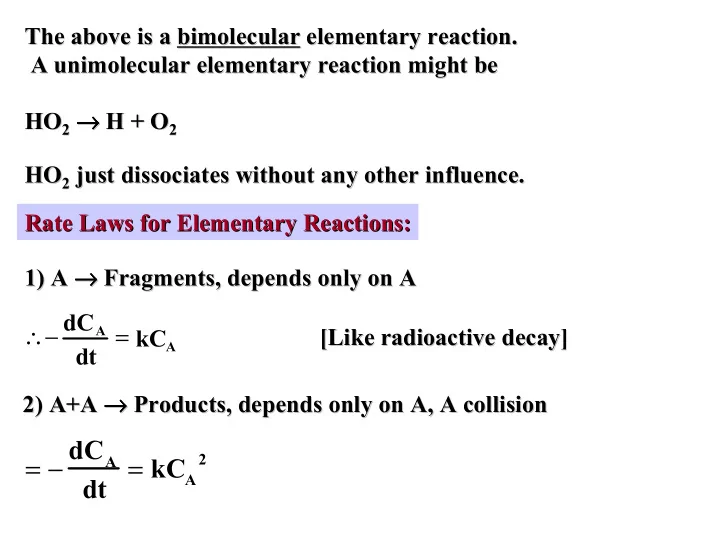
The above is a bimolecular bimolecular elementary reaction. elementary reaction. The above is a A unimolecular unimolecular elementary reaction might be elementary reaction might be A → H + O → 2 → → → → → → HO 2 H + O 2 HO 2 HO 2 just dissociates without any other influence. HO 2 just dissociates without any other influence. Rate Laws for Elementary Reactions: Rate Laws for Elementary Reactions: 1) A → → → → → Fragments, depends only on A → → → Fragments, depends only on A 1) A ∴− dC A = kC A [Like radioactive decay] [Like radioactive decay] dt 2) A+A → → → → → → Products, depends only on A, A collision → → Products, depends only on A, A collision 2) A+A = − dC A dt = kC A 2

→ → Products. A + B → → → → → → Products. A + B − dC A = − dC B = kC A C B . dt dt Elementary reaction is one place where stoichiometry stoichiometry and and Elementary reaction is one place where rate are are related. However never know when you have an related. However never know when you have an rate elementary reaction. Must guess and then verify with experiment. nt. elementary reaction. Must guess and then verify with experime Elementary reactions are hypothetical constructs! Elementary reactions are hypothetical constructs!

Unimolecular Decompositions Decompositions Unimolecular An example of An example of Mechanisms, , Steady Steady State State Approximation Approximation, , Mechanisms and Elementary Elementary Reactions Reactions and → → Fragments A → → → → → → Fragments A Observed Experimentally to Observed Experimentally to be first order in pyrazine pyrazine. . be first order in pyrazine pyrazine

− dA dt = k [ A ] or C → → → → C + B with B → → → → or C - - B C + B with − d [ C − B ] = k [ C − B ] dt Lindemann [Lord [Lord Cherwell Cherwell] suggested the following mechanism: ] suggested the following mechanism: Lindemann Collisions in general produce molecules with higher than average Collisions in general produce molecules with higher than average energy. Consider a box full of A and let those molecules which energy. Consider a box full of A and let those molecules which have an energy E* greater than or equal to that necessary for have an energy E* greater than or equal to that necessary for decomposition be A*. decomposition be A*. Assume, however, that after A* is produced by a collision it hangs gs Assume, however, that after A* is produced by a collision it han around for some time before decomposing. This time lag between around for some time before decomposing. This time lag between activation and reaction may be thought of as the time necessary to to activation and reaction may be thought of as the time necessary transfer energy among the internal (vibrational vibrational) coordinates. ) coordinates. transfer energy among the internal (

* exists for a reasonable time it could suffer a collision and dr If A * exists for a reasonable time it could suffer a collision and drop op If A down to a lower energy where it cannot decompose. down to a lower energy where it cannot decompose. Collision between Collision between k 1 k 1 A(E 1 ) + A(E 2 ) A(E 1 ) + A(E 2 ) A*(E*) + A(E + A(E 3 ) A*(E*) 3 ) A(E 1 ) and A(E 2 ) A(E 1 ) and A(E 2 ) creates “activated” creates “activated” Collision A*(E*) A*(E*) E 1 , E 2 , E 3 < E E min ; E E* ≥ * ≥ E E min = energy necessary for decomposition E 1 , E 2 , E 3 < min ; min = energy necessary for decomposition k − 1  →   Competition between Competition between A * (E * ) + A(E 4 ) A(E 5 ) + A(E 6 ) reaction of A*(E*) A*(E*) to to reaction of     Collional cooling form products and form products and    k 2  step collisional cooling of cooling of collisional     A*(E*) to to A*(E*) ↓ ↓ ↓ ↓ Reaction step produce unreactive unreactive produce Products A(E 5 ) and A(E 6 ) A(E 5 ) and A(E 6 ) Again E 4 , E 5 , E 6 < E E min (k 1 , k - , and k 2 are kinetic rate constants) Again E 4 , E 5 , E 6 < (k 1 , k 1 , and k 2 are kinetic rate constants) min -1

k 2 is decomposition step assumed irreversible. k 2 is decomposition step assumed irreversible. Mechanism Elementary Steps: Mechanism Elementary Steps: ∗ + A A + A  →   A k 1 Step 1 Step 1 ∗ + A k − 1  →   A + A Step 2 Step 2 A ∗ k 2  →   P Step 3 Step 3 A dP = k 2 [ A ∗ ] Step 1 Step 2 Step 3 Step 1 Step 2 Step 3 dt d [ A ∗ ] = k 1 [ A ] 2 − k − 1 [ A ∗ ][ A ] − k 2 [ A ∗ ] dt t know what [A * * ] is, however the number of [A ] is, however the number of [A * * ] must be small Don’ ’t know what [A ] must be small Don or the reaction would go to completion very quickly. A* is a or the reaction would go to completion very quickly. A* is a “bottleneck bottleneck” ” for the reaction since product is only formed via A*. for the reaction since product is only formed via A*. “

* ] → → for [A * ] → → → → → → Therefore we assume a steady state steady state for [A This is a most most Therefore we assume a This is a important “ “trick trick” ” or approximation: or approximation: important The Steady State Steady State The d[A * * ]/ [A * * ][A] [A * * ] ]/dt dt = 0 = k = 0 = k 1 [A] 2 2 - -k k - ][A]- -k k 2 ] d[A 1 [A] 1 [A 2 [A -1 Approximation Approximation * at the same rate.) (Assumes are making and destroying A * at the same rate.) (Assumes are making and destroying A → → → → Solve for [A * ] → → → → * ] = k [A * ] = k 1 [A] 2 2 / ( k / ( k 2 +k - [A] ) } Key Point: [A 1 [A] 2 +k 1 [A] ) } Key Point: -1 Steady state approach allows us to solve for concentration Steady state approach allows us to solve for concentration species [A * * ] in terms of of unknown unknown species [A ] in terms of known known [A] concentration. [A] concentration. of Fundamental result of Fundamental result of Rate = dP ∗ ] = k 2 k 1 [ A ] 2 dt = k 2 [ A Lindemann “ “Unimolecular Unimolecular Lindemann k 2 + k − 1 [ A ] Reaction Mechanism” Reaction Mechanism” Note, multistep mechanism leads to complex rate expression!

Two Limiting Cases Two Limiting Cases Rate = dP ∗ ] = k 2 k 1 [ A ] 2 dt = k 2 [ A k 2 + k − 1 [ A ] I) k - [A] << k 2 I) k 1 [A] << k -1 2 * is much This says the rate of decomposition of A * is much faster faster This says the rate of decomposition of A than the rate of deactivation. than the rate of deactivation. Using k 2 >>k - [A] and the expression for dP dP/ /dt dt: : Using k 2 >>k 1 [A] and the expression for -1 Take k -1 [A] to be 0 compared to k 2 Rate = dP/dt = k 2 [A*] = k 2 k 1 [A] 2 /(k 2 +k -1 [A]) 2 } dt ≅ ≅ ≅ k ≅ ≅ ≅ } } 2nd order in [A] } } } ≅ ≅ [A] 2 } } Thus, dP dP/ /dt k 1 2nd order in [A] Thus, 1 [A] At this point, it looks like Mr Mr. . Lindemann Lindemann will have to hand will have to hand At this point, it looks like in his Theorists’ ’ Club ID card since his scheme seems to Club ID card since his scheme seems to in his Theorists predict a second order kinetic dependence on [A] 2 ! predict a second order kinetic dependence on [A] 2 !

“Physical Interpretation Physical Interpretation” ” of this particular limit: of this particular limit: “ Mechanism Elementary Steps: Mechanism Elementary Steps: ∗ + A A + A  →   A k 1 Step 1 d[A*]/dt = k 1 [A] 2 Step 1 ∗ + A k − 1 Slow enough to be ignored!  →   A + A Step 2 Step 2 A ∗ k 2 So fast that every A* formed  →   P Step 3 Step 3 A Reacts immediately! Reaction rate here is just the rate at which [A*] is formed since e Reaction rate here is just the rate at which [A*] is formed sinc every A* formed falls apart to product P immediately. The rate of f every A* formed falls apart to product P immediately. The rate o formation of A* is obtained from the first step: d[A*]/dt dt = k 1 [A] 2 formation of A* is obtained from the first step: d[A*]/ So the reaction becomes a simple binary collison collison model, second model, second So the reaction becomes a simple binary order process in this limit. order process in this limit.