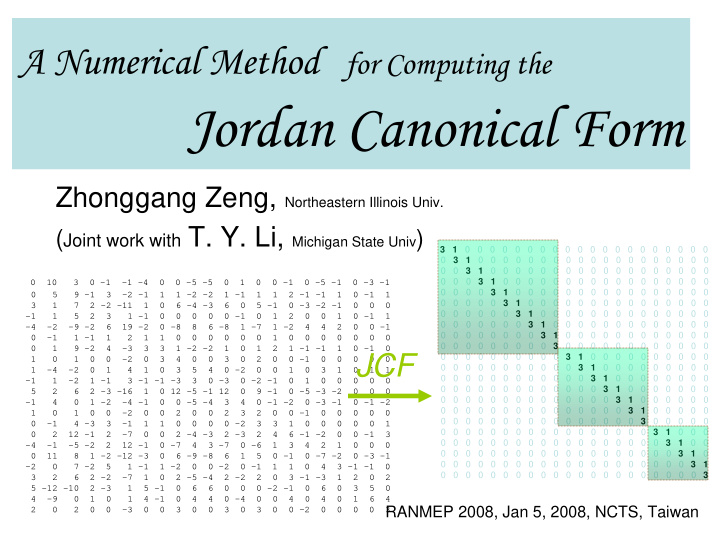
jordan canonical form
play

Jordan Canonical Form Zhonggang Zeng, Northeastern Illinois Univ. ( - PowerPoint PPT Presentation

Apr 30, 2023 •174 likes •606 views

A Numerical Method for Computing the Jordan Canonical Form Zhonggang Zeng, Northeastern Illinois Univ. ( Joint work with T. Y. Li, Michigan State Univ ) 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1

  1. A Numerical Method for Computing the Jordan Canonical Form Zhonggang Zeng, Northeastern Illinois Univ. ( Joint work with T. Y. Li, Michigan State Univ ) 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 10 3 0 -1 -1 -4 0 0 -5 -5 0 1 0 0 -1 0 -5 -1 0 -3 -1 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 5 9 -1 3 -2 -1 1 1 -2 -2 1 -1 1 1 2 -1 -1 1 0 -1 1 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 7 2 -2 -11 1 0 6 -4 -3 6 0 5 -1 0 -3 -2 -1 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 -1 1 5 2 3 1 -1 0 0 0 0 0 -1 0 1 2 0 0 1 0 -1 1 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 -4 -2 -9 -2 6 19 -2 0 -8 8 6 -8 1 -7 1 -2 4 4 2 0 0 -1 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 -1 1 -1 1 2 1 1 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 0 0 0 0 0 0 0 0 0 0 0 0 0 1 9 -2 4 -3 3 3 1 -2 -2 1 0 1 2 1 -1 -1 1 0 -1 0 JCF 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 1 0 1 0 0 -2 0 3 4 0 0 3 0 2 0 0 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 1 -4 -2 0 1 4 1 0 3 5 4 0 -2 0 0 1 0 3 1 0 1 1 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 -1 1 -2 1 -1 3 -1 -1 -3 3 0 -3 0 -2 -1 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 5 2 6 2 -3 -16 1 0 12 -5 -1 12 0 9 -1 0 -5 -3 -2 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 -1 4 0 1 -2 -4 -1 0 0 -5 -4 3 4 0 -1 -2 0 -3 -1 0 -1 -2 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 1 0 1 0 0 -2 0 0 2 0 0 2 3 2 0 0 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 0 0 0 0 0 0 -1 4 -3 3 -1 1 1 0 0 0 0 -2 3 3 1 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 2 12 -1 2 -7 0 0 2 -4 -3 2 -3 2 4 6 -1 -2 0 0 -1 3 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 -4 -1 -5 -2 2 12 -1 0 -7 4 3 -7 0 -6 1 3 4 2 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 11 8 1 -2 -12 -3 0 6 -9 -8 6 1 5 0 -1 0 -7 -2 0 -3 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 -2 0 7 -2 5 1 -1 1 -2 0 0 -2 0 -1 1 1 0 4 3 -1 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 3 2 6 2 -2 -7 1 0 2 -5 -4 2 -2 2 0 3 -1 -3 1 2 0 2 5 -12 -10 2 -3 1 5 -1 0 6 6 0 0 0 -2 -1 0 6 0 3 5 0 4 -9 0 1 0 1 4 -1 0 4 4 0 -4 0 0 4 0 4 0 1 6 4 RANMEP 2008, Jan 5, 2008, NCTS, Taiwan 2 0 2 0 0 -3 0 0 3 0 0 3 0 3 0 0 -2 0 0 0 0 3

  2. Theorem: Every matrix corresponds to a Jordan Canonical Form − = 1 A X J X ⎡ ⎤ J λ ⎡ 1 ⎤ 1 ⎢ ⎥ k ⎢ ⎥ λ J O ⎢ ⎥ ⎢ ⎥ = 2 = k J J ⎢ ⎥ ⎢ ⎥ k 1 O O ⎢ ⎥ ⎢ ⎥ λ ⎣ ⎦ ⎣ ⎦ J k l However, it is extremely difficult to compute JCF with numerical computation 1

  3. Problem : Jordan Canonical Form (JCF) 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 10 3 0 -1 -1 -4 0 0 -5 -5 0 1 0 0 -1 0 -5 -1 0 -3 -1 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 5 9 -1 3 -2 -1 1 1 -2 -2 1 -1 1 1 2 -1 -1 1 0 -1 1 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 7 2 -2 -11 1 0 6 -4 -3 6 0 5 -1 0 -3 -2 -1 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 0 -1 1 5 2 3 1 -1 0 0 0 0 0 -1 0 1 2 0 0 1 0 -1 1 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 0 -4 -2 -9 -2 6 19 -2 0 -8 8 6 -8 1 -7 1 -2 4 4 2 0 0 -1 0 0 0 0 0 0 0 0 0 3 0 0 0 0 0 0 0 0 0 0 0 0 JCF 0 -1 1 -1 1 2 1 1 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 0 0 1 9 -2 4 -3 3 3 1 -2 -2 1 0 1 2 1 -1 -1 1 0 -1 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 0 1 0 1 0 0 -2 0 3 4 0 0 3 0 2 0 0 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 0 1 -4 -2 0 1 4 1 0 3 5 4 0 -2 0 0 1 0 3 1 0 1 1 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 0 -1 1 -2 1 -1 3 -1 -1 -3 3 0 -3 0 -2 -1 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 0 5 2 6 2 -3 -16 1 0 12 -5 -1 12 0 9 -1 0 -5 -3 -2 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 0 -1 4 0 1 -2 -4 -1 0 0 -5 -4 3 4 0 -1 -2 0 -3 -1 0 -1 -2 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 0 0 0 0 0 1 0 1 0 0 -2 0 0 2 0 0 2 3 2 0 0 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 0 -1 4 -3 3 -1 1 1 0 0 0 0 -2 3 3 1 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 0 0 2 12 -1 2 -7 0 0 2 -4 -3 2 -3 2 4 6 -1 -2 0 0 -1 3 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 -4 -1 -5 -2 2 12 -1 0 -7 4 3 -7 0 -6 1 3 4 2 1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 1 0 11 8 1 -2 -12 -3 0 6 -9 -8 6 1 5 0 -1 0 -7 -2 0 -3 -1 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 3 -2 0 7 -2 5 1 -1 1 -2 0 0 -2 0 -1 1 1 0 4 3 -1 -1 0 3 2 6 2 -2 -7 1 0 2 -5 -4 2 -2 2 0 3 -1 -3 1 2 0 2 5 -12 -10 2 -3 1 5 -1 0 6 6 0 0 0 -2 -1 0 6 0 3 5 0 4 -9 0 1 0 1 4 -1 0 4 4 0 -4 0 0 4 0 4 0 1 6 4 2 0 2 0 0 -3 0 0 3 0 0 3 0 3 0 0 -2 0 0 0 0 3 { } λ = ( ) 3 A − λ + 15 E ( 10 ) A 2

  4. Computing JCF is an ill-posed problem λ λ 1 1 λ λ 1 1 λ λ λ λ A = X X -1 1 1 A λ λ λ λ 1 1 λ λ λ 3 λ λ λ 4 λ 2 → ~ = + ε Under arbitrary perturbation A A A E λ 5 λ λ 1 λ 1 λ 8 λ 6 λ 2 ~ = ~ ~ − λ 3 λ 7 1 λ 4 A X X λ 5 λ 6 λ 7 λ 8 Can we recover the lost eigenvalue and JCF of A using perturbed matrix à ? 3

  5. Background and motivation • The new JCF algorithm is motivated by our recent works on solving ill-posed problems in numerical polynomial algebra (approximate GCD, factorization, multiplicity structure, polynomial elimination, etc.) • Numerical polynomial algebra and numerical algebraic geometry are fertile application fields of numerical linear algebra • JCF computing has direct application in numerical irreducible factorization of multivariate polynomials. The preliminary implementation of the JCF algorithm is part of ApaLab (http://www.neiu.edu/~zzeng) 4

  6. The pioneer work (Kublanovskaya-Ruhe): 1. Compute eigenvalues of A with Francis QR algorithm 2. Identify an eigenvalue cluster, and use the mean λ as an approximation to a multiple eigenvalues 3. Use a sequence of SVD and unitary similarity transformations on A - λ I to obtain a local staircase form 4. Repeat on other multiple eigenvalues. Reference: Kublanovskaya (1968) Ruhe (1970) Golub & Wilkinson (1976) Kågström & Ruhe (1980) Beelen & Van Dooren (1988) The main difficulty: accuracy of the multiple eigenvalue 5

  7. >> J = find(abs(e-1)<0.2); >> sum(e(J))/20 ans = 1.00000000000000 >> K = find(abs(e-2)<0.2); >> sum(e(K))/15 ans = 2.00000000000000 If the cluster is properly identified, the center is usually be very good approximation to the multiple eigenvalue 6

  8. 7 The cluster mean can still be sensitive:

  9. Can you solve ( x - 1.0 ) 100 = 0 x 100 - 100 x 99 + 4950 x 98 - 161700 x 97 + 3921225 x 96 - ... - 100 x + 1 = 0 8

Download Presentation
Download Policy: The content available on the website is offered to you 'AS IS' for your personal information and use only. It cannot be commercialized, licensed, or distributed on other websites without prior consent from the author. To download a presentation, simply click this link. If you encounter any difficulties during the download process, it's possible that the publisher has removed the file from their server.

Recommend

- Character set - Character escape conventions - Canonical form - Line editing conventions

- Character set - Character escape conventions - Canonical form - Line editing conventions

CHARACTER STREAM PROCESSING - Character set - Character escape conventions - Canonical form - Line editing conventions CHARACTER SET CONSIDERATIONS minimum: graphic range equal to a typical office typewriter support: throughout system -programs

476 views • 9 slides
Discrete time systems Aim lecture: Show how Jordan canonical forms can be useful to study some

Discrete time systems Aim lecture: Show how Jordan canonical forms can be useful to study some

Discrete time systems Aim lecture: Show how Jordan canonical forms can be useful to study some discrete time systems. Recall from first year the following important example of a discrete system. Example of first order discrete time system Let v

134 views • 9 slides
YANG Open Issues IETF 73 Martin Bjrklund mbj@tail-f.com 2 Canonical form of data types 1(3)

YANG Open Issues IETF 73 Martin Bjrklund mbj@tail-f.com 2 Canonical form of data types 1(3)

YANG Open Issues IETF 73 Martin Bjrklund mbj@tail-f.com 2 Canonical form of data types 1(3) Some data types accept multiple lexical representations of the same values. For example, the positive integer 42 can be represented as 42

852 views • 16 slides
The asymptotics of the holomorphic torsion form Martin Puchol Institut Camille Jordan

The asymptotics of the holomorphic torsion form Martin Puchol Institut Camille Jordan

Introduction Definition Statement of the result Tplitz operators Asymptotic heat kernel The asymptotics of the holomorphic torsion form Martin Puchol Institut Camille Jordan Universit Lyon 1 Index Theory and Singular Structures

218 views • 21 slides
Integral Feminine Jordan Mayyada Abu Jaber The Five Ecosystems of Jordan Source: Jordan's

Integral Feminine Jordan Mayyada Abu Jaber The Five Ecosystems of Jordan Source: Jordan's

Integral Feminine Jordan Mayyada Abu Jaber The Five Ecosystems of Jordan Source: Jordan's Third National Communication Report on Climate Change- 2014 Educated J Jordan The MENA region has achieved gender parity when it comes to

462 views • 18 slides
Digital Design Discussion: Boolean Algebra Boolean Expression Equivalence Boolean Function

Digital Design Discussion: Boolean Algebra Boolean Expression Equivalence Boolean Function

Principles Of Digital Design Discussion: Boolean Algebra Boolean Expression Equivalence Boolean Function Complement Boolean Expression to Canonical Form Canonical Form to Standard Form Boolean Expression Equivalence Method: Boolean

219 views • 5 slides
Jordan Jordan is a land rich in history. Since the dawn of civilization, Jordan has played an

Jordan Jordan is a land rich in history. Since the dawn of civilization, Jordan has played an

Jordan Jordan is a land rich in history. Since the dawn of civilization, Jordan has played an important role in trade between east and west because of its geographic location at the crossroads of Asia, Africa and Europe. It has been home to some

1.39k views • 94 slides
Canonical Typology Danny Hieber Hieber, Daniel W. 2011. Canonical Typology. Talk given to the

Canonical Typology Danny Hieber Hieber, Daniel W. 2011. Canonical Typology. Talk given to the

Canonical Typology Danny Hieber Hieber, Daniel W. 2011. Canonical Typology. Talk given to the Content Development 1 Department, Rosetta Stone, Harrisonburg, VA, Sept 2011. Outline 1. Overview of Typology 2. Overview of Canonical Typology 3.

647 views • 31 slides
MIR-Canon: Improving Code Diff Through Canonical Transformation Puyan Lotfi Apple Inc. 1

MIR-Canon: Improving Code Diff Through Canonical Transformation Puyan Lotfi Apple Inc. 1

MIR-Canon: Improving Code Diff Through Canonical Transformation Puyan Lotfi Apple Inc. 1 What is MIR? MIR (Machine IR) is the newer IR form for MachineInstrs. 2 What is MIR? bb.0: liveins: $w0, $x1, $x2 %1:gpr64 = COPY $x1

1.27k views • 72 slides
View Volumes Canonical View Volumes Why Canonical View Volumes? University of British Columbia

View Volumes Canonical View Volumes Why Canonical View Volumes? University of British Columbia

View Volumes Canonical View Volumes Why Canonical View Volumes? University of British Columbia CPSC 314 Computer Graphics Jan-Apr 2016 standardized viewing volume representation specifies field-of-view, used for clipping permits

52 views • 3 slides
Around canonical heights in arithmetic dynamics Shu Kawaguchi Arithmetic 2015 - Silvermania

Around canonical heights in arithmetic dynamics Shu Kawaguchi Arithmetic 2015 - Silvermania

Around canonical heights in arithmetic dynamics Shu Kawaguchi Arithmetic 2015 - Silvermania August 14, 2015 1 / 39 Plan of the talk (2005 Email? 2008 AIM) 1 Canonical heights for polarized dynamical systems 2 Canonical heights on affine space

1.23k views • 46 slides
University of Jordan University of Jordan Developmental dysplasia of the hip (DDH) . A

University of Jordan University of Jordan Developmental dysplasia of the hip (DDH) . A

Associated risk factors in children who had late presentation of DDH Freih Odeh Abu Hassan F.R.C.S (Eng.), F.R.C.S (Tr. &amp; Orth.) Professor of Orthopedics and Pediatrics Orthopedics Surgeon University of Jordan University of Jordan

251 views • 24 slides
K E D b . D a L a t a B a s e Jordan Vincent XML processing using GPGPU Jordan

K E D b . D a L a t a B a s e Jordan Vincent XML processing using GPGPU Jordan

XML processing using GPGPU Research proposal Jordan Vincent University of Tsukuba February 2, 2011 K E D b . D a L a t a B a s e Jordan Vincent XML processing using GPGPU Jordan Vincent Academic achievements Engineering degree

528 views • 18 slides
A canonical martingale coupling Workshop on Optimal Transportation and Appplications Nicolas

A canonical martingale coupling Workshop on Optimal Transportation and Appplications Nicolas

A canonical martingale coupling A canonical martingale coupling Workshop on Optimal Transportation and Appplications Nicolas JUILLET Universit de Strasbourg Pisa, November 2012 Nicolas JUILLET A canonical martingale coupling A canonical

244 views • 12 slides
BCNucleation-Aggregation Workshop Grand canonical molecular dynamics simulation Grand canonical

BCNucleation-Aggregation Workshop Grand canonical molecular dynamics simulation Grand canonical

D EPARTMENT OF M ECHANICAL E NGINEERING BCNucleation-Aggregation Workshop Grand canonical molecular dynamics simulation Grand canonical molecular dynamics simulation of homogeneous nucleation Universitat de Barcelona, Departament de Fsica

513 views • 18 slides
Introducing... Benjamin Mako Hill GULEV: Ubuntu Canonical Ltd. Ubuntu A GNU/Linux Operating

Introducing... Benjamin Mako Hill GULEV: Ubuntu Canonical Ltd. Ubuntu A GNU/Linux Operating

Introducing... Benjamin Mako Hill GULEV: Ubuntu Canonical Ltd. Ubuntu A GNU/Linux Operating System: Past, Present and Future Benjamin Mako Hill mako@canonical.com Ubuntu http://www.ubuntulinux.org Canonical Ltd. http://www.canonical.com

536 views • 22 slides
Scheduling pipelined applications: models, algorithms and complexity Anne Benoit GRAAL team,

Scheduling pipelined applications: models, algorithms and complexity Anne Benoit GRAAL team,

Introduction Models Complexity results Conclusion Scheduling pipelined applications: models, algorithms and complexity Anne Benoit GRAAL team, LIP, Ecole Normale Sup erieure de Lyon, France ASTEC meeting in Les Plantiers, France June

1.42k views • 129 slides
Low Frequency Waves in HF Heating of Mid-latitude Ionosphere Surja Sharma, Bengt Eliasson*, Xi

Low Frequency Waves in HF Heating of Mid-latitude Ionosphere Surja Sharma, Bengt Eliasson*, Xi

Low Frequency Waves in HF Heating of Mid-latitude Ionosphere Surja Sharma, Bengt Eliasson*, Xi Shao, Gennady Milikh and Dennis Papadopoulos University of Maryland, College Park *University of Strathclyde, Glasgow, UK IES 2015 Alexandria, VA

221 views • 21 slides
Heat full statistics: heavy tails and fluctuations control Annalisa Panati, CPT, Universit de

Heat full statistics: heavy tails and fluctuations control Annalisa Panati, CPT, Universit de

Plan Full statistics and quantum fluctuation relations Heat fluctuations: classical VS quantum Mathematical settings and results Heat full statistics: heavy tails and fluctuations control Annalisa Panati, CPT, Universit de Toulon joint

467 views • 28 slides
$TITLE: M9-2.GMS: Two Country Large-Group Monopolistic Competition $ontext same data is used as

$TITLE: M9-2.GMS: Two Country Large-Group Monopolistic Competition $ontext same data is used as

C:\jim\COURSES\8858\code-bk 2012\M9-2.gms Tuesday, March 20, 2012 1:10:38 PM Page 1 $TITLE: M9-2.GMS: Two Country Large-Group Monopolistic Competition $ontext same data is used as used in M9-1, calibrated to monopolistic comp YI YJ XMI

651 views • 11 slides
SDP and eigenvalue bounds for the graph partition problem Renata Sotirov and Edwin van Dam

SDP and eigenvalue bounds for the graph partition problem Renata Sotirov and Edwin van Dam

SDP and GPP SotirovR SDP and eigenvalue bounds for the graph partition problem Renata Sotirov and Edwin van Dam Tilburg University, The Netherlands SDP and GPP Outline . . . SotirovR the graph partition problem SDP and GPP Outline . . .

1.31k views • 107 slides
Iden%fiability of Subsampled/Mixed- Frequency Structural VAR models Alex Tank University of

Iden%fiability of Subsampled/Mixed- Frequency Structural VAR models Alex Tank University of

Iden%fiability of Subsampled/Mixed- Frequency Structural VAR models Alex Tank University of Washington Joint work with Emily Fox and Ali Shojaie 1 %me 2 %me 3 x 31 x 41 x 11 x 21 x 71 x 51 x 61 x 22 x 32 x 72 x 12 x 42 x 52 x 62 %me 4

318 views • 31 slides
Overview to Exchange-Traded Funds 800.983.0903 06.30. 2006 For Advisor Use Only Risks

Overview to Exchange-Traded Funds 800.983.0903 06.30. 2006 For Advisor Use Only Risks

Overview to Exchange-Traded Funds 800.983.0903 06.30. 2006 For Advisor Use Only Risks Market Risk: Fund Shares are subject to market fluctuations caused by such factors as economic and political developments, changes in interest rates

1.38k views • 94 slides
!&quot;#&quot;$%&amp;'()**+'(,$-- .&quot;#&quot;/)012/(&amp;'()**+'0 !&quot;#$%&amp;'(

!&quot;#&quot;$%&amp;'()**+'(,$-- .&quot;#&quot;/)012/(&amp;'()**+'0 !&quot;#$%&amp;'(

!&quot;&quot;#$%%&amp;''()*(+*,'&amp;+('-. !&quot;#&quot;$%&amp;'()**+'(,$-- .&quot;#&quot;/)012/(&amp;'()**+'0 !&quot;#$%&amp;'( )*+,%-./'$&quot; -/0)*(+*,'&amp;+('-. !&quot;#$%&amp;'())*+,#)'-%$#').+/01&amp;2+&quot;#$)34

726 views • 57 slides

More recommend