in
play

In theoretic Fgm ( X invariant k GW 1) us GW . = Ijf , M ) - PowerPoint PPT Presentation

Dec 01, 2023 •358 likes •537 views

X.ge#ofQu4sche-s Virtual surfaced on . Introduction O . surface X Ici smooth CE Hak tf ( X ,Z ) , 2) projective e , . In theoretic Fgm ( X invariant k GW 1) us GW . = Ijf , M ) invariant refined ( M ) MTH ( v ) VW

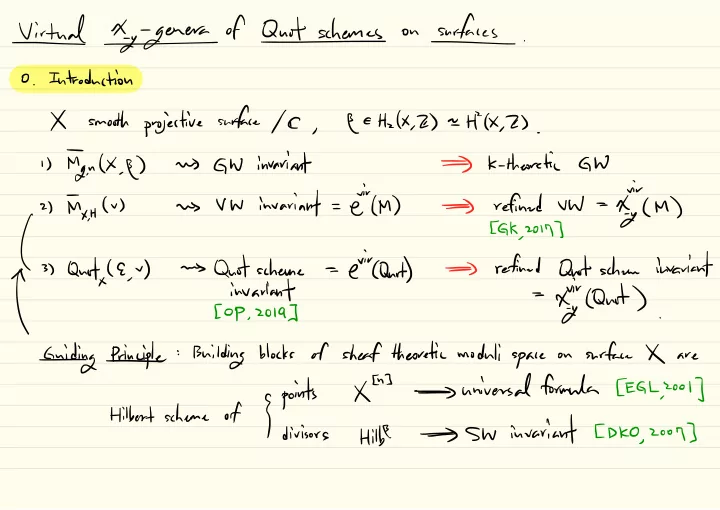
  1. X.ge#ofQu4sche-s Virtual surfaced on . Introduction O . surface X Ici smooth CE Hak tf ( X ,Z ) , 2) projective e , . In theoretic Fgm ( X invariant ⇒ k GW 1) us GW . = Ijf , M ) invariant refined ( M ) MTH ( v ) VW VW ⇒ 2) us = ref Qxjyt EGK , 2017 ] → Quintus - Eiland ! wait ) Quihuis !;ye , ⇒ - \ 2019 ] [ op , Principle deign ⇐ theoretic surface blocks of sheaf moduli X Building : space are on ED universal formula EEG 42001 ] X points → of scheme Hilbert g invariant 2007 ] EDKO divisors Hill → SW ,

  2. virtual invariant Quit 1 of scheme . - f - f E RKCQ ) c. CQ ) } → of 'Ia→ o . ] n ) - - - Quirk CET e. , / , is : - - XCX , Q ) n - - . Q ) ] Howls Tan = t { v. dim land ) difference Nn = = ' Q ) Obs EH ( s . = , = EATS Ots ' Q ) Hom ( Q , s④k×Y = o , To :L [ anti , stand " O - term ⇒ ⇒ Pot 7- - . z - + . . Crd ( TIE ) evil Quit ) S EFG Z , 20103 E in ⇒ [ - tf cyst Ari e Zap y - rigour ) x cant = : , re go 't , gland )

  3. ( Quit ) of [ 01320193 Work 2 S Conjecture on . . 2¥ :{ q ) Def " en ) Iz , E E ( Quark . . J Q KED . E . HEY ⇒ explicit formula . . :÷÷÷::÷ : ' min → z : :* ¥wf with pg① ⇒ E ¥141 ) ( t of general type minimal surface simply → X conn , N ) ( lkx . see o [ 'T ' ' ' .ee#re-- E' ' ⇒ . - N ( Kx ) ITI l Sw - - . I of rational surface X E 3) blow 2¥ f- N - I → up - , , . ( III ) " .ec#--q : in . ⇒ 2x . = , . me

  4. subring ( OP ) e QCE ) . X QUID ⇒ Z E elf ) simply connected mm related coefficients i. are e. , . with pg Think ) X surface ⇒ Q lot ) meet ) E + any , ? . I ' " . a yxq , ecq e key : Refined invariant Quit scheme ( under ¥ invariant SW I 1) . " ' " min genotype e -2 H swlkx ) X ⇒ Ps > o - - , . ) ( certain 8 under condition universal formula 2) Multiplicative UN AJ e.g . , Blow formula rational ? 3) blow Is term up up FEI 2x e. a 2g , HE ? ⇐ LEI . , ,

  5. jw - Witten of ko ] E ' ' See .bz invariants } / . . out ahem . theory they CE H' H2 ) X smooth pug surface . ( virtual localization ( Mochizuki ) , . calculation ) =p } t =/ c. ( Gass ) some divisor Hills effective sit DEX : . . HYO , > CD ) ) H' ( ODD ) ) Obs Tan = = , vde=RK - term of ⇒ pot I a . ( he ) he consider Oiiiibxxl D) ftp.wq * s h " = g . I ' QCD ) Hills Pic Dts AJ sending → : . " ) AH A Ix ( hkn-LHilh.IT ' e H* l pic ) C x. 2) Sw ( e ) Define = re : . , ' , then ( X. 2) If AH vde Z = o - - . 4 U dyEHHB.TK SWIM =

  6. Math result Correct S A 4 assumption . . if swing Det ( L ) is We e say ti ) [ vdq=o ] . ten [ te with ti to ⇒ Sw et - . - . new ) . 7- many examples ( all t in top ] cases surface length IAI N%lB7 o ⇒ ⇒ f N Sw X ed f- o : any , . . vdq -0=0 - : effective f decomposition f- to Only Ot : . - . , relatively minimal a :X surface elliott C length N → su e : fibers ( the o ) . - supported ' EF q - on " " - . mutt of k×n rate F . Zariski Lemma with it length N X surface II e Sw : pg > any . ] Ko ED I file length N ⑤ length N SW Sw → f : : .

  7. ( L ) Mainthm N Assume length e Sw : . W universal UN Umi 7- seise QLY.eu " KED ) E ' s.tn ie . - . , . , , refined Quit The generating series invariants of scheme is equivariant z÷÷ian⇒=E÷÷÷÷¥ .su#uii.x..niiis:.x..uwiii: run ' f nuttily . " 's Tx " 's . formula contribution similar invariants for ref Rink monopole to VW 7- : . :÷÷÷÷÷÷÷:÷÷÷i÷:⇒÷÷ :÷ ÷ : known !

  8. ( Sketch of of proof Quoted understood In general , geometry of Qut N scheme higher is poorly . a QWEN.eu ) no EN with E' E' distinct Let weights ⇒ w war . . " ) , . ( similar IP as a mahthnqswkyt x Qnrkce inn ) ( Quote ' U , ein Dx = . . . , t fu , fit . f- . . loans ! pits t HN hit n = . . each into fixed factors of Induced obstruction theory . { virtual of 2017 ] class " [ GSY , Eh 55 , [ Quoted ? is nested scheme Question what Hilbert : . Quoted .cn?--XtmkHilbe-- " X 7- identification here m=n+ 2 , 1968 ) LF . # ) " ' " e ( coke ) 7 Eantxcesensj " I XEHH [ X' Propels n - rank m ② Special of pf , 2019 ] [ ① GT compare : case . ,

  9. of localization virtual 1999 ] [ GP By , , surfs : ) 's * f . canteen wineskin x . * J 2 - C sth ) - 2 swigs Sw - . - . tf q= ft . .×XTmN ) thx n hit ×EnP× . . - - . ' . f . vdq sit " ] o - - . f Hills safe ; ) ⇒ zi÷÷i"÷÷÷÷ " " ' ( sth ) xi⇒ " ⇒ " n . . # ] E 2001 EGL , universal formula multiplicative 7- . THE

  10. ( to each ) of the Main -1hm EINE 5 Applications case . . . surface ThmA_ X 9=0 any . , . ( " on z where in - - - Yat ) 4- E) 4 Ytitj ) IN IT ( I l HY ) ti 4) If ) UNL t = e yyuqyN - woo ⇒ . = . . , . run polynomial ' EPN - IN rots of in . .tn Here , of t y : - . are of . . . . . . . . P , " ! I = . e.g - y ly 'VE ) pncqt , Putty ) , y * = + yzqr P . utiytyyq I - . - - . ' ) Italy -136-1458/+36 y4t9y5tyb ) E ( Hay -19 y 't ( P ft I y . = - , ' ft ' E 't ( ztaytay t y y - . it taking Top ] y I By recovers - - , .

  11. relatively minimal elliptic surface the fibers supported Thmts :X C a → f : on ( . . , ziiiii " t.ei.i.iii.is " " . invariants [ DKO ] SW above computed by By are , (22-21×10×3) . Dd I Shoki ) c = E- dFt§ajFj o Eajcmj d > o St . , F 's of fibers multiple where multiplicity ng are .

  12. minimal surface of general type X Time with pg > o . . OELEN ) ( have GN.e.GE QYVE lkx ( For ' we f- any .com#e . , z : " . E " " :* : uhm " - . x .¥mI÷i¥÷⇐ .eu#.i;Eiix.I a , ( Set . - l - N s - ' " ) HI that [ Ck Note swlkx ] ) by 2013 = , . lift technical S taking Cop ] By assumptions y recover I we - - , I EH helkx 'D ( simply conn ,

  13. X surface N Thnx length f SW : any . . . up formula ) ( Blow ÷ : " " " " " " " ( " " . - ( E. Bine ) Ii ! where " got , econ - . , Qlyllf -2 Blye As ) = e . EN ] JE - l - N IJI - 445-4-554 e. 2 131µm , I 131µm = - . - - y 'VE ) . C , ( I of ) I - . Blye , by is to compute Greg it For N any given easy , , . I 2=0 .

  14. I :i A o ) ⇒ [ [ c- Qcyslq ) if surface with is Thin X µ of ) > pg min IEE bfmh . surface Then Let X with ( proof ) be apply b/c Ps > > o pg o . . surface X , ee Qty ) I of ) since Bbv assume we may : . , . ) Thin IAI abelian is kod SW class ⇒ ⇒ K3 only f- o - o o : or - . ( I kod time ⇒ ⇒ o - . of Than II : minimal surface f- Kx is only SW class ⇒ lad ⇒ ° z = general type with pg . > o TEH what if ? Question happen : surface Then connected E) EQCE ) [ Jop ] X 2 simply 2020 , . . - more general descendents In fact , they study .

  15. Reduced invariant surfaces 6 of k3 . . X surface Quit Usual scheme trivial Kc k3 invariants are . ⇒ surjective e:ptgf EI Is H' tox ) E EHIQQ ) Exits a .Q ) Obs cosette = . I I Ext 't 0,970-3=0 Hom I Q ,Q④kx ) Hock ← ) i : . : with consider reduced obstruction We theory : . kerf Obs E) → = . N L ) X surface k3 . , x.gl xjdcaukcd.e.us ) ) ⇒ = unheard where I ) in S E is class ntlg IP → m f = - curve . thin the Euler characteristic level Above in [ op ) of Rmd D was proven on ' FLEET ) . . ' S ht [ KY ] smooth to CENT is be computed 2000 2) by are proven , . F - -

  16. with Together Thu formula t KY with Core privation . I ! tix ! canteens ) my is I , .eu = , of coefficient fed in ftp.fy EFI - Ey ) a - Elysa - Etty ) h 'll 4- Easy ) . EY a ) a Etf ) - - - . ↳ " tie - Yoshioka Kawai I rat 't tradable . particular = in is In , , .

  17. Further 7 questions . Lw " invariant stable invariants Qut scheme 4.) 3.) → ambling invariant with ⇒ X rationality gnestfn ? / variant of - primitive Reda surface with k3 N higher ( non ( . - - me - trivial reduced invariant of Define non . Quit ,yp( formula v ) Relative they S degeneration . - ( -

Recommend

More recommend