i
play

i 1 / 61 Introduction and Context 2 / 61 3 / 61 3 / 61 3 / 61 - PowerPoint PPT Presentation

Jun 20, 2023 •198 likes •2k views

Representing and Computing with Types in Dynamically Typed Languages Extending Dynamic Language Expressivity to Accommodate Rationally Typed Sequences Jim Edward Newton Thesis defense for the title: Doctorat de lUniversit e Sorbonne

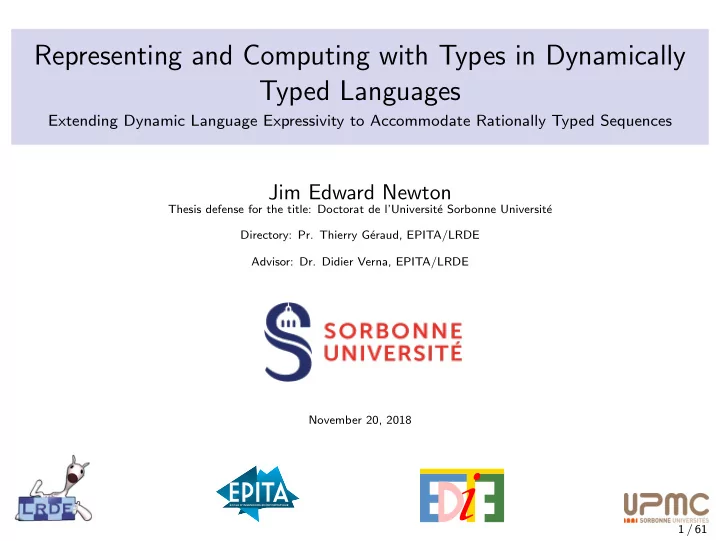
  1. How does a DFA work as a type predicate? number 2 number ( a 1 1.0 b "a" symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  2. How does a DFA work as a type predicate? number number 2 1 1.0 b "a" (a symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  3. How does a DFA work as a type predicate? number 2 number 1.0 b "a" symbol (a 1 symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  4. How does a DFA work as a type predicate? number number 2 b "a" (a 1 1.0 symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  5. How does a DFA work as a type predicate? number number 2 "a" symbol (a 1 1.0 b symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  6. How does a DFA work as a type predicate? number 2 number (a 1 1.0 b "a" symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  7. How does a DFA work as a type predicate? number 2 number (a 1 1.0 b "a" symbol symbol 0 1 "the" symbol "an" c 2 22 222 d 2/3) string 3 string 15 / 61

  8. How does a DFA work as a type predicate? number number 2 (a 1 1.0 b "a" symbol symbol 0 1 c 2 "an" "the" symbol 22 222 d 2/3) string 3 string 15 / 61

  9. How does a DFA work as a type predicate? number number 2 (a 1 1.0 b "a" symbol symbol 0 1 2 "an" "the" c symbol 22 222 d 2/3) string 3 string 15 / 61

  10. How does a DFA work as a type predicate? number 2 number (a 1 1.0 b "a" symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  11. How does a DFA work as a type predicate? number number 2 (a 1 1.0 b "a" symbol "an" "the" c 2 symbol 0 1 222 d 2/3) symbol 22 string 3 string 15 / 61

  12. How does a DFA work as a type predicate? number 2 number (a 1 1.0 b "a" symbol symbol "an" "the" c 2 0 1 symbol d 2/3) 22 222 string 3 string 15 / 61

  13. How does a DFA work as a type predicate? number number 2 (a 1 1.0 b "a" symbol symbol "an" "the" c 2 0 1 symbol 2/3 ) 22 222 d string 3 string 15 / 61

  14. How does a DFA work as a type predicate? Yes, it’s a match! number number 2 (a 1 1.0 b "a" symbol symbol 0 1 "an" "the" c 2 symbol 22 222 d 2/3) string 3 string 15 / 61

  15. Code generated from ( symbol · ( number + ∨ string + )) + number ( tagbody 0 number 2 ( u n l e s s seq ( r e t u r n n i l )) ( typecase ( pop seq ) ( symbol ( go 1)) symbol symbol 0 1 ( t ( r e t u r n n i l ) ) ) symbol 1 ( u n l e s s seq ( r e t u r n n i l )) string 3 ( typecase ( pop seq ) ( number ( go 2)) ( s t r i n g ( go 3)) string ( t ( r e t u r n n i l ) ) ) 3 2 ( u n l e s s seq ( r e t u r n t )) ( u n l e s s seq ( r e t u r n t )) ( typecase ( pop seq ) ( typecase ( pop seq ) ( s t r i n g ( go 3)) ( number ( go 2)) ( symbol ( go 1)) ( symbol ( go 1)) ( t ( r e t u r n n i l ) ) ) ) ) ( t ( r e t u r n n i l ) ) ) 16 / 61

  16. Lambda-lists characterized by RTEs A lambda-list in Common Lisp has a fixed part (defun foo (a b) ...) 17 / 61

  17. Lambda-lists characterized by RTEs A lambda-list in Common Lisp has a fixed part, an optional part (defun foo (a b &optional c) ...) 17 / 61

  18. Lambda-lists characterized by RTEs A lambda-list in Common Lisp has a fixed part, an optional part, and a repeating part. (defun foo (a b &optional c &key x y) ...) 17 / 61

  19. Lambda-lists characterized by RTEs A lambda-list in Common Lisp has a fixed part, an optional part, and a repeating part part. Any of the variables may be restricted by type declarations. (defun foo (a b &optional c &key x y) (declare (type integer a x) (type string b c y)) ...) 17 / 61

  20. Lambda-lists characterized by RTEs A lambda-list in Common Lisp has a fixed part, an optional part, and a repeating part part. Any of the variables may be restricted by type declarations. (defun foo (a b &optional c &key x y) (declare (type integer a x) (type string b c y)) ...) The set of valid argument lists for a function may be characterized by an RTE. 17 / 61

  21. destructuring-bind is a different syntax for calling an anonymous function. ( destructuring − bind ( a (b c ) &key ( x t ) ( y ”” ) z ) DATA ( d e c l a r e ( type fixnum a b c z ) ( type symbol x ) ( type s t r i n g y )) . . . body . . . ) 18 / 61

  22. destructuring-bind is a different syntax for calling an anonymous function. ( destructuring − bind ( a (b c ) &key ( x t ) ( y ”” ) z ) DATA ( d e c l a r e ( type fixnum a b c z ) ( type symbol x ) ( type s t r i n g y )) . . . body . . . ) For example: DATA = (2 (3 4) :y "a" :x ’b) ; YES 18 / 61

  23. destructuring-bind is a different syntax for calling an anonymous function. ( destructuring − bind ( a (b c ) &key ( x t ) ( y ”” ) z ) DATA ( d e c l a r e ( type fixnum a b c z ) ( type symbol x ) ( type s t r i n g y )) . . . body . . . ) For example: DATA = (2 (3 4) :y "a" :x ’b) ; YES DATA = (2 (3 4) :y "a" :x ’b :x 42 :y "hello" :y nil) ; YES 18 / 61

  24. destructuring-bind is a different syntax for calling an anonymous function. ( destructuring − bind ( a (b c ) &key ( x t ) ( y ”” ) z ) DATA ( d e c l a r e ( type fixnum a b c z ) ( type symbol x ) ( type s t r i n g y )) . . . body . . . ) For example: DATA = (2 (3 4) :y "a" :x ’b) ; YES DATA = (2 (3 4) :y "a" :x ’b :x 42 :y "hello" :y nil) ; YES DATA = (2 (3 4) :y "a" :x 42 :x ’b) ; NO An invalid argument list will signal an error at run-time. 18 / 61

  25. QUESTION: Can we select an appropriate lambda-list matching DATA , avoiding a run-time error? We propose destructuring-case . ( d e s t r u c t u r i n g − c a s e DATA ; ; Case − 1 (( a b &o p t i o n a l ( c ”” )) ( d e c l a r e ( type i n t e g e r a ) ( type s t r i n g b c )) . . . body . . . ) ; ; Case − 2 (( a ( b c ) &key ( x t ) ( y ”” ) z ) ( d e c l a r e ( type fixnum a b c ) ( type symbol x ) ( type s t r i n g y ) ( type l i s t z )) . . . body . . . ) ) 19 / 61

  26. QUESTION: Can we select an appropriate lambda-list matching DATA , avoiding a run-time error? We propose destructuring-case . ( d e s t r u c t u r i n g − c a s e DATA ; ; Case − 1 (( a b &o p t i o n a l ( c ”” )) ( d e c l a r e ( type i n t e g e r a ) ( type s t r i n g b c )) ◮ integer · string · string ? . . . body . . . ) ; ; Case − 2 (( a ( b c ) &key ( x t ) ( y ”” ) z ) ( d e c l a r e ( type fixnum a b c ) ( type symbol x ) ( type s t r i n g y ) ( type l i s t z )) . . . body . . . ) ) 19 / 61

  27. QUESTION: Can we select an appropriate lambda-list matching DATA , avoiding a run-time error? We propose destructuring-case . ( d e s t r u c t u r i n g − c a s e DATA ; ; Case − 1 ◮ integer · string · string ? (( a b &o p t i o n a l ( c ”” )) ( d e c l a r e ( type i n t e g e r a ) ( type s t r i n g b c )) integer string 0 1 2 string . . . body . . . ) 3 ; ; Case − 2 (( a ( b c ) &key ( x t ) ( y ”” ) z ) ( d e c l a r e ( type fixnum a b c ) ( type symbol x ) ( type s t r i n g y ) ( type l i s t z )) . . . body . . . ) ) 19 / 61

  28. QUESTION: Can we select an appropriate lambda-list matching DATA , avoiding a run-time error? We propose destructuring-case . ( d e s t r u c t u r i n g − c a s e DATA ; ; Case − 1 ◮ integer · string · string ? (( a b &o p t i o n a l ( c ”” )) ( d e c l a r e ( type i n t e g e r a ) ( type s t r i n g b c )) integer string 0 1 2 string . . . body . . . ) 3 ; ; Case − 2 ◮ What is the rational (( a ( b c ) &key ( x t ) ( y ”” ) z ) ( d e c l a r e ( type fixnum a b c ) type expression? ( type symbol x ) ( type s t r i n g y ) ( type l i s t z )) . . . body . . . ) ) 19 / 61

  29. QUESTION: Can we select an appropriate lambda-list matching DATA , avoiding a run-time error? We propose destructuring-case . ( d e s t r u c t u r i n g − c a s e DATA ; ; Case − 1 ◮ integer · string · string ? (( a b &o p t i o n a l ( c ”” )) ( d e c l a r e ( type i n t e g e r a ) ( type s t r i n g b c )) integer string 0 1 2 string . . . body . . . ) 3 ; ; Case − 2 ◮ What is the rational (( a ( b c ) &key ( x t ) ( y ”” ) z ) ( d e c l a r e ( type fixnum a b c ) type expression? ( type symbol x ) ◮ What is the DFA? ( type s t r i n g y ) ( type l i s t z )) . . . body . . . ) ) 19 / 61

  30. RTE auto-generated from destructuring lambda-list ( : cat ( : cat fixnum ( : and l i s t ( r t e ( : cat fixnum fixnum ) ) ) ) ( : and ( : ∗ ( : cat ( : or ( e q l : x ) ( e q l : y ) ( e q l : z )) t )) ( : cat ( : ∗ ( : cat ( not ( e q l : x )) t )) ( : ? ( : cat ( e q l : x ) symbol ( : ∗ t ) ) ) ) ( : cat ( : ∗ ( : cat ( not ( e q l : y )) t )) ( : ? ( : cat ( e q l : y ) s t r i n g ( : ∗ t ) ) ) ) ( : cat ( : ∗ ( : cat ( not ( e q l : z )) t )) ( : ? ( : cat ( e q l : z ) l i s t ( : ∗ t ) ) ) ) ) ) 20 / 61

  31. DFA corresponding to auto-generated RTE 5 T7 T3 6 T4 9 T8 T5 4 T5 T6 T9 8 T10 7 T7 12 T10 3 T6 27 T3 T3 T7 11 T7 26 T11 T4 T8 T8 T4 15 10 24 25 T4 13 T12 T1 T2 0 1 2 T5 T5 T7 14 16 28 T10 T10 T6 T6 19 T7 21 22 T13 T3 T8 T3 20 T4 23 17 T7 T5 18 T 1 = fixnum T 2 = (and list (rte (:cat fixnum fixnum))) T 3 = (eql :x) T 4 = (eql :y) T 5 = (eql :z) T 6 = symbol T 7 = t T 8 = string T 9 = (member :x :y) T 10 = list T 11 = (member :x :y :z) T 12 = (member :x :z) T 13 = (member :y :z) 21 / 61

  32. DFA corresponding to auto-generated RTE 5 T7 T3 6 T4 9 T8 T5 4 T5 T6 T9 8 T10 7 T7 12 T10 3 T6 27 T3 T3 T7 11 T7 26 T8 T11 T8 T4 T4 15 10 24 25 13 T4 T12 T1 T2 0 1 2 T5 T5 T7 14 16 28 T10 T10 T6 T6 19 T7 21 22 T13 T3 T8 T3 T4 20 23 17 T7 T5 18 Multiple transitions from states give rise to serialization problem. 21 / 61

  33. Rational Type Expressions (RTEs) with overlapping types ( number · integer ) ∨ ( integer · number ) We have non-deterministic (NFA). integer ⊂ number integer 1 integer number 2 number 0 integer number 3 22 / 61

  34. Rational Type Expressions (RTEs) with overlapping types ( number · integer ) ∨ ( integer · number ) We want deterministic (DFA). (and number (not integer)) 1 integer 2 number 0 integer 3 22 / 61

  35. Maximal Disjoint Type Decomposition 23 / 61

  36. MDTD: decompose a set of types into disjoint types ◮ Given A i as possibly overlapping regions, A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 24 / 61

  37. MDTD: decompose a set of types into disjoint types ◮ Given A i as possibly overlapping regions, ◮ Calculate X i as disjoint regions. A 1 A 3 A 2 X 13 X 2 X 3 X 11 X 1 X 12 X 10 A 4 A 6 X 6 X 4 X 9 A 5 A 7 X 7 X 5 A 8 X 8 24 / 61

  38. MDTD problem: Baseline algorithm Are there any disjoint sets? A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  39. MDTD problem: Baseline algorithm Yes, A 7 intersects no other set. A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  40. MDTD problem: Baseline algorithm So collect it into D : D = { A 7 } A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  41. MDTD problem: Baseline algorithm Select any intersecting pair of sets. A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  42. MDTD problem: Baseline algorithm E.g. , A 2 . Does A 2 intersect anything? A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  43. MDTD problem: Baseline algorithm Yes. A 2 intersects A 4 . A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  44. MDTD problem: Baseline algorithm So calculate the standard partition of A 2 and A 4 A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  45. MDTD problem: Baseline algorithm The standard partition is { A 2 ∩ A 4 , ... } A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  46. MDTD problem: Baseline algorithm The standard partition is { A 2 ∩ A 4 , A 4 ∩ A 2 , ... } A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  47. MDTD problem: Baseline algorithm The standard partition is { A 2 ∩ A 4 , A 4 ∩ A 2 , A 2 ∩ A 4 } A 1 A 3 A 2 A 4 A 6 A 5 A 7 A 8 25 / 61

  48. MDTD problem: Baseline algorithm So remove { A 2 , A 4 } and add { A 2 ∩ A 4 , A 4 ∩ A 2 , A 2 ∩ A 4 } . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  49. MDTD problem: Baseline algorithm Now, restart. Anything disjoint from everything else? No. A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  50. MDTD problem: Baseline algorithm So select any intersecting pair. A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  51. MDTD problem: Baseline algorithm E.g. , A 4 ∩ A 2 . Does it intersect anything? A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  52. MDTD problem: Baseline algorithm Yes, it intersects A 5 . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  53. MDTD problem: Baseline algorithm So calculate the standard partition of A 5 and A 4 ∩ A 2 . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  54. MDTD problem: Baseline algorithm The standard partition is { A 5 , ... } . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  55. MDTD problem: Baseline algorithm The standard partition is { ..., A 4 ∩ A 2 ∩ A 5 } . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  56. MDTD problem: Baseline algorithm The standard partition is { A 5 , A 4 ∩ A 2 ∩ A 5 } . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 A 6 A 5 A 7 A 8 25 / 61

  57. MDTD problem: Baseline algorithm So remove { A 5 , A 4 ∩ A 2 } and add { A 5 , A 4 ∩ A 2 ∩ A 5 } . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 ∩ A 5 A 6 A 5 A 7 A 8 25 / 61

  58. MDTD problem: Baseline algorithm So remove { A 5 , A 4 ∩ A 2 } and add { A 5 , A 4 ∩ A 2 ∩ A 5 } . A 5 is in both sets. We can optimize, because A 5 ⊂ A 4 ∩ A 2 . A 1 A 3 A 2 ∩ A 4 A 2 ∩ A 4 A 4 ∩ A 2 ∩ A 5 A 6 A 5 A 7 A 8 25 / 61

  59. MDTD problem: Baseline algorithm Continue the procedure until collecting all the pairwise disjoint sets. X 13 X 2 X 3 X 11 X 1 X 12 X 10 X 6 X 4 X 9 X 7 X 5 X 8 25 / 61

  60. MDTD problem: Baseline algorithm Calculating all the colored regions as subsets of original overlapping sets. X 13 X 2 X 3 X 11 X 1 X 12 X 10 X 6 X 4 X 9 X 7 X 5 X 8 26 / 61

  61. X5 =? =? =? =? X3 X4 X6 X11 List with set semantics ◮ Insertion into list with set semantics has linear complexity. ◮ Type equivalence check is X i ⊂ X j ∧ X j ⊂ X i ? ◮ And prevents us from using a hash table to implement sets. ◮ This equivalence function is SLOW! 27 / 61

  62. MDTD result: type specifiers are explosive in size X2 : ( and ( and ( and A2 ( not ( and ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ) ) ) ( not ( and ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ( not ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ) ) ) ) ( not ( and ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ( not ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ) ) ) ) X3 : ( and ( and ( and A1 ( not A2 )) A3) ( not A4 ) ) X10 : ( and ( and ( and A2 ( not ( and ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ) ) ) ( not ( and ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ( not ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ) ) ) ) ( and ( and A4 ( not ( and ( and A1 ( not A2 ) ) A3 ) ) ) ( not ( and A3 ( not ( and A1 ( not A2 ) ) ) ) ) ) ) 28 / 61

  63. Problems with baseline algorithm ◮ Explosive size of type specifiers ◮ O ( n 2 ) search on each iteration ◮ Set semantics for lists of types: ◮ To maintain uniqueness, O ( n ) list-insertion. ◮ Equivalent types may appear in many different forms. ... No canonical form ◮ Slow set-equivalence algorithm. ◮ Many redundant checks ◮ subtypep may return don’t-know 29 / 61

  64. Strategies to Improving MDTD algorithm We can do better. ◮ Optimize current algorithm (caching etc). ◮ Change the algorithm. ◮ Change the data structure representing the sets (CL types). 30 / 61

  65. Graph-based MDTD 1 A 1 A 3 8 6 A 2 4 A 4 A 6 A 5 3 5 A 7 A 8 7 2 Topology graph representing type hierarchy and intersections. We find MDTD by controlled breaking and re-wiring of this graph. 31 / 61

  66. Step 0 Node Boolean Standard expression partition → A 1 ∩ A 6 1 A 1 1 2 A 2 3 A 3 6 8 4 A 4 5 A 5 4 6 A 6 collect into D A 6 8 A 8 3 5 X 7 A 7 2 32 / 61

  67. Step 1 Node Boolean Standard expression partition → A 1 ∩ A 6 ∩ A 5 1 A 1 1 2 A 2 3 A 3 8 4 → A 4 ∩ A 5 A 4 5 A 5 A 5 collect into D 4 8 → A 8 ∩ A 5 A 8 X 6 A 6 5 3 X 7 A 7 2 33 / 61

Recommend

More recommend