i
play

I IaB = Fb = ( IaB ) b = IabB = IAB B = IA = Iab b IaB ~ = ~ - PowerPoint PPT Presentation

Jun 17, 2023 •205 likes •319 views

Lecture 27: Magne&c Force, Torque, Circular Motor and the Hall Effect Magnetic Force: ~ B field exerts a force on the source, q ~ v ~ v ~ Nq l F = q ~ B ~ t = I l l F = I

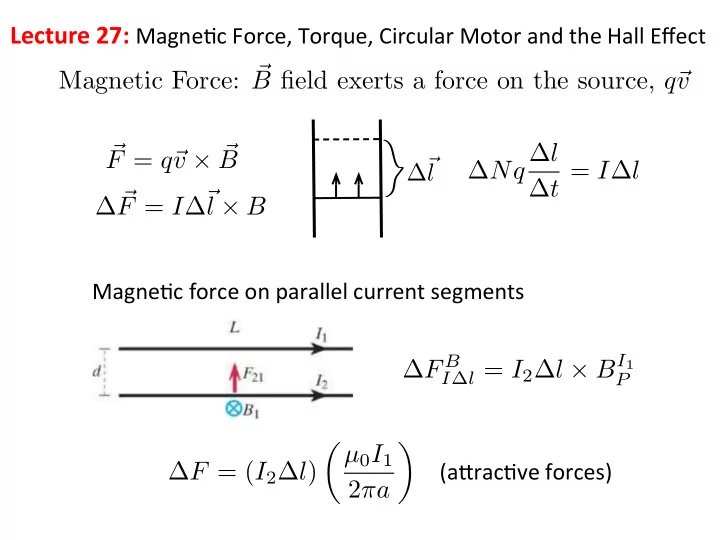
  1. Lecture ¡27: ¡ Magne&c ¡Force, ¡Torque, ¡Circular ¡Motor ¡and ¡the ¡Hall ¡Effect ¡ Magnetic Force: ~ B field exerts a force on the source, q ~ v ~ v × ~ ∆ Nq ∆ l F = q ~ B ∆ ~ ∆ t = I ∆ l l F = I ∆ ~ ∆ ~ l × B Magne&c ¡force ¡on ¡parallel ¡current ¡segments ¡ I ∆ l = I 2 ∆ l × B I 1 ∆ F B P ✓ µ 0 I 1 ◆ (a>rac&ve ¡forces) ¡ ∆ F = ( I 2 ∆ l ) 2 π a

  2. Current ¡loop ¡in ¡a ¡constant ¡B ¡field ¡ F 1 = IaB ⊗ F 2 = F 4 = 0 F 3 = IaB � F ⇤ = F 1 + F 3 = IaB − IaB = 0 Torque ¡about ¡midline ¡00: ¡ I IaB τ = Fb = ( IaB ) b = IabB = IAB B µ = IA = Iab b IaB µ × ~ ⌧ = ~ B (present case: ⌧ = µB ) ~ (view ¡from ¡the ¡baseline ¡loop) ¡

  3. General ¡Case: ¡ µ × ~ ⌧ = ~ ~ B circular loop. ~ B is in the xy plane, ↵ < 90 � ⇣ ⌘ So ~ − sin ↵ ˆ I + cos ↵ ˆ B = B j µ = µ ˆ ~ j � � i j k � � µ × ~ � � ~ ⌧ = ~ B = µB 0 1 0 � � � � − sin ↵ cos ↵ 0 � � = µB sin ↵ ˆ k

  4. Circular ¡Mo&on ¡– ¡Force ¡due ¡to ¡B ¡on ¡qv ¡ Lec26-4 Motion of a charged particle in the plane perpendicular to the magnetic field v 1 P 1 A proton is moving in a clockwise direction in a plane perpendicular to a r uniform magnetic field B pointing out of P 2 the page. Determine the direction of the magnetic force at P 1 and at P 2 . v 2 fig. 26.4 Choice Direction of Force at P 1 Direction of Force at P 2 1 Up Left 2 Down Left 3 Up Right 4 Down Right

  5. Circular ¡Mo+on ¡– ¡Force ¡due ¡to ¡B ¡on ¡qv ¡ v 1 P 1 Given: B = const. , out of the paper r P 2 Find: The direction of the force at v 2 fig. 26.4 P 1 & at P 2 At ¡P 1 : ¡ F cp = mv 2 = qvB qv Period T = 2 π r r r = mv B v qB = 2 π m Down qB At ¡P 2 : ¡ Period is independent ✓ ◆ ω = 2 π T = qB of v, r. m B qv

  6. Lec26-5 Circular motion in the plane perpendicular to a constant magnetic field A proton of charge e and mass m is moving in a plane perpendicular to a uniform magnetic field. Investigate the radius and period of its circular motion. Hint: I F cp = mv 2 = evB r I T = 2 π r v Choice R (radius of the circular motion) T (period of circular motion) 1 mv / eB As v increases, T increases 2 mv / eB T is independent of v 3 eB / mv As v increases, T increases 4 eB / mv T is independent of v

  7. Alternative ∆ V ⇒ E > 0 , E < 0 f ( t ) = ∆ V 2 sin ω t ∆ V t = successive acceleration of charged particle

  8. Hall ¡Effect: ¡Determine ¡the ¡sign ¡of ¡charge ¡carriers ¡ I I ∆ ~ F = ~ I ∆ l × ~ B B B B F If ¡carriers ¡have ¡+ ¡charges: ¡ A G I hall B For case 1: Static polarized charge creates E ↑ ∴ Potential at B is higher. Take a test charge to be pushed by F = qvB to raise it’s potential from A to B . V B − V A = W A → B = qvBl = vBl q q

  9. Lec27-3 Hall Experiment y B v d x B I G z fig. 27.3a Fig. 27.3a shows a portion of a metal strip in a region of uniform magnetic field. A galvanometer is connected to the upper and lower surfaces of the strip. A current I flows to the right (so the electron drift velocity v d is to the left) and B = B ˆ z . Determine the direction of the magnetic force experienced by the drifitng electrons and the direction of the (conventional) Hall current through the galvanometer. Choice Direction of Hall current through the galvanometer F B 1 Up Up 2 Up Down 3 Down Up 4 Down Down

  10. If ¡carriers ¡have ¡– ¡charges: ¡ A G I hall B For case 2: E ↓ V A − V B = vBl Determina&on ¡of ¡v: ¡ I I = | q | i = | q | nAv, v = | q | nA n = # of electrons 2 N A (# of moles) − − − − − − → volume volume 2 valence

Recommend

More recommend