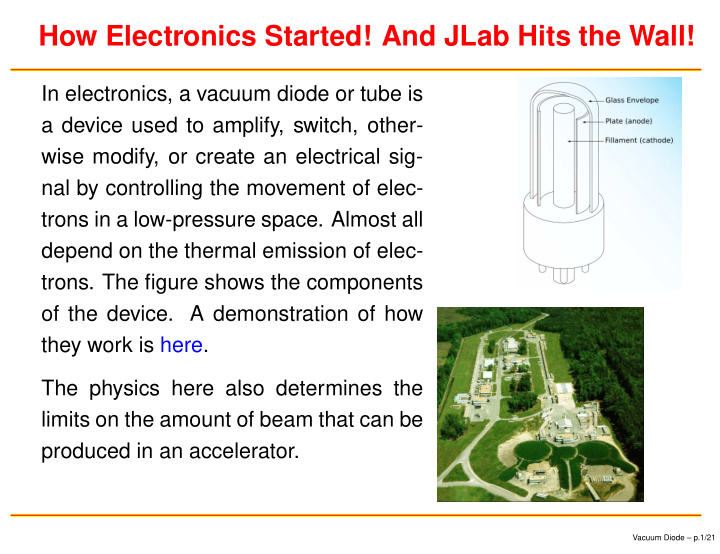



How Electronics Started! And JLab Hits the Wall! In electronics, a vacuum diode or tube is a device used to amplify, switch, other- wise modify, or create an electrical sig- nal by controlling the movement of elec- trons in a low-pressure space. Almost all depend on the thermal emission of elec- trons. The figure shows the components of the device. A demonstration of how they work is here. The physics here also determines the limits on the amount of beam that can be produced in an accelerator. Vacuum Diode – p.1/21
The Child-Langmuir Law In a vacuum diode, electrons are boiled off a hot cathode at zero potential and accelerated across a gap to the anode at a positive potential V 0 . The cloud of moving electrons within the gap (called the space charge) builds up and reduces the field at the cathode surface to zero. From then on a steady current flows between the plates. Suppose two plates are large relative to the separation ( A >> d 2 in the figure) so that edge effects can be ignored and V , ρ , and the electron speed v are functions of only x . d A Electron x Cathode (V=0) Anode(V=V ) 0 Vacuum Diode – p.2/21
The Child-Langmuir Law 1. What is Poisson’s equation for the region between the plates? 2. Assuming the electrons start from rest at the cathode, what is their speed at point x ? 3. In the steady state I , the current, is independent of x . How are ρ and v related? 4. Now generate a differential equation for V by eliminating ρ and v and solve this equation for V as a function of x , V 0 , and d . Make a plot to compare V ( x ) and the potential without the space charge. 5. What are ρ and v as functions of x ? d 6. Show that A Electron I = KV 3 / 2 0 and find K . This the Child-Langmuir law. x Cathode (V=0) Anode(V=V ) 0 Vacuum Diode – p.3/21
Why Should You Care? The Physics 132 Picture positive negative v t ∆ d + I = JA = nqv d A = A ρd V v Area = A d E Ohm’s Law i 10 Voltage (V) d 9 slope = 46 8 ± Ω 8 R = 46.5 Ω meas 7 I = 1 6 R V 5 4 V = IR 3 2 1 0 0 0.02 0.04 0.06 0.08 0.1 0.12 0.14 0.16 0.18 Current (A) 2007-10-16 11:35:02 Vacuum Diode – p.4/21
Why Should You Care, Part Deaux? January 11, 2006 Vacuum Diode – p.5/21
Coulomb’s Law and Superposition Measuring the Electrostatic Force of Charges. Use a torsion pendulum and charged spheres. Vacuum Diode – p.6/21
Coulomb’s Law and Superposition Measuring the Electrostatic Force of Charges. Use a torsion pendulum and charged spheres. Intermediate Lab Results Evidence for Coulomb’s Law. 350 300 n � 1.99 � 0.04 250 Fix charge and vary distance. 200 Θ � deg � 150 100 50 0 0.00 0.05 0.10 0.15 0.20 0.25 r � m � Vacuum Diode – p.6/21
Coulomb’s Law and Superposition Measuring the Electrostatic Force of Charges. Use a torsion pendulum and charged spheres. Intermediate Lab Results Evidence for Coulomb’s Law. 350 300 n � 1.99 � 0.04 250 Fix charge and vary distance. 200 Θ � deg � 150 100 50 0 0.00 0.05 0.10 0.15 0.20 0.25 r � m � Intermediate Lab Results 100 Evidence for Superposition. Quadratic term: 0.5 � 0.3 80 Q � V 60 Fix distance and vary charge on one sphere. Θ � deg � 40 20 0 7 0 1 2 3 4 5 6 V � kV � Vacuum Diode – p.6/21
Electric Field of a Ring A ring of radius r as shown in the figure has a positive charge distribution per unit length with total charge q . Calculate the electric field � E along the axis of the ring at a point lying a distance x from the center of the ring. Get your answer in terms of r , x , q . What happens as x → ∞ ? r Q x q Vacuum Diode – p.7/21
Electric Field of a Ring A ring of radius r as shown in the figure has a positive charge distribution per unit length with total charge q . Calculate the electric field � E along the axis of the ring at a point lying a distance x from the center of the ring. Get your answer in terms of r , x , q . What happens as x → ∞ ? r Q x q Vacuum Diode – p.7/21
Electric Field Lines Point Charges Electric Dipole Positive Charges Vacuum Diode – p.8/21
Properties of Electric Field Lines Field lines start from positive charges (sources) and end at negative ones (sinks). Are symmetrical around point charges. Density of field lines is related to strength of field. Direction of field is tangent to the field line. Field lines never cross! Vacuum Diode – p.9/21
Electric Flux � � Φ E = EA cos θ = � E · � E · � Φ E = EA A Φ E = dA Vacuum Diode – p.10/21
An Example of Electric Flux A nonuniform magnetic field is described by � E = (3 . 0 N/C − m ) x ˆ x + (4 . 0 N/C )ˆ y pierces the Gaussian cube shown in the figure. What is the flux through the cube? Note the orientation of the coordinate system. Vacuum Diode – p.11/21
Gauss’s Law What is the flux from a point charge q at the origin through a sphere centered at the origin of radius r ? Vacuum Diode – p.12/21
Differential Surface and Volume Elements Cartesian Coordinates Cylindrical Coordinates Spherical Coordinates z (x,y,z) φ y x θ z φ θ x y dA = r 2 d cos θdφ dA = dxdy dA = ρdφdz dτ = r 2 d cos θdφdr dτ = dxdydz dτ = ρdφdzdρ Vacuum Diode – p.13/21
Applying Gauss’s Law: Nuclear � E The nucleus of a gold atom has a radius R = 6 . 2 × 10 − 15 m and a positive charge q = Ze where Z = 79 is the atomic number. Assume the gold nucleus is spherical and the charge is uniformly distributed in the volume. What is the electric field for any r ? Vacuum Diode – p.14/21
Applying Gauss’s Law: Nuclear � E The nucleus of a gold atom has a radius R = 6 . 2 × 10 − 15 m and a positive charge q = Ze where Z = 79 is the atomic number. Assume the gold nucleus is spherical and the charge is uniformly distributed in the volume. What is the electric field for any r ? Electric field of a gold nucleus 3.0 2.5 E � 10 21 N � C � 2.0 1.5 1.0 0.5 0.0 0 5 10 15 20 25 r � fm � Vacuum Diode – p.14/21
An Example of Applying ∇ × � E A nonuniform magnetic field is described by � E = (3 . 0 N/C − m ) x ˆ x + (4 . 0 N/C )ˆ y pierces the Gaussian cube shown in the figure. Is this a conservative field? Vacuum Diode – p.15/21
Applying V 1. What is the potential energy of a point charge? 2. What is the potential inside and outside a uniformly charged sphere of total charge q and radius R ? 3. What is the electric field for the previous question? Vacuum Diode – p.16/21
The Child-Langmuir Law In a vacuum diode, electrons are boiled off a hot cathode at potential zero and accelerated across a gap to the anode at a positive potential V 0 . The cloud of moving electrons within the gap (called the space charge) builds up and reduces the field at the cathode surface to zero. From then on a steady current flows between the plates. Suppose two plates are large relative to the separation ( A >> d 2 in the figure) so that edge effects can be ignored and V , ρ , and the electron speed v are functions of only x . d A Electron x Cathode (V=0) Anode(V=V ) 0 Vacuum Diode – p.17/21
The Child-Langmuir Law 1. What is Poisson’s equation for the region between the plates? 2. Assuming the electrons start from rest at the cathode, what is their speed at point x ? 3. In the steady state I , the current, is independent of x . How are ρ and v related? 4. Now generate a differential equation for V by eliminating ρ and v and solve this equation for V as a function of x , V 0 , and d . Make a plot to compare V ( x ) and the potential without the space charge. 5. What are ρ and v as functions of x ? d 6. Show that A Electron I = KV 3 / 2 0 and find K . This the Child-Langmuir law. x Cathode (V=0) Anode(V=V ) 0 Vacuum Diode – p.18/21
Using Mathematica To Solve the DE Vacuum Diode – p.19/21
The Child-Langmuir Law: Results Comparing the Child-Langmuir Law with the no-space-charge solution. 1.0 Blue � ’132’ picture 0.8 Red � Child � Langmuir Current � units of I max � 0.6 0.4 0.2 0.0 0.0 0.2 0.4 0.6 0.8 1.0 V � units of V max � Vacuum Diode – p.20/21
More Child-Langmuir Law Results Comparing the Child-Langmuir Law in vacuum with a copper wire. Wires vs. Vacuum 10 14 Current Density � A � m 2 � Red � copper wire 10 11 Blue � vacuum 10 8 10 5 100 0.0 0.2 0.4 0.6 0.8 1.0 V � megavolts � � J CU = 1 V J CL = 4 ǫ 0 2 e ρ = 1 . 7 × 10 − 8 Ω − m V 3 / 2 d = 0 . 1 m 9 d 2 ρ d m e Vacuum Diode – p.21/21
Recommend
More recommend