givg
play

givg Ya a contradiction vectors This will With - you . Value of - PDF document

Oct 13, 2022 •276 likes •328 views

- 8 16 : 30 18 : 00 LECTURE - QUANTUM CORRELATIONS Sarkar De basis Calcutta University , Rosen ( EPR Paradox ) 1935 Einstein , Podolsk : - In terms of Bohm 's Spin te Representation 101714 1952 : EPR - Gleason 's

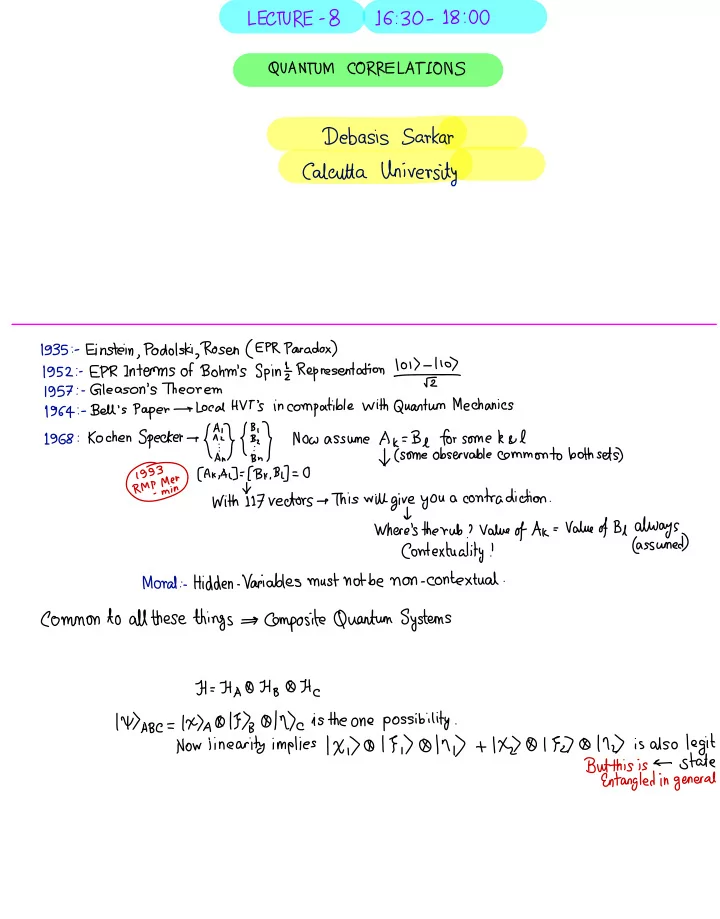
  1. � ⇒ - 8 16 : 30 18 : 00 LECTURE - QUANTUM CORRELATIONS Sarkar De basis Calcutta University , Rosen ( EPR Paradox ) 1935 Einstein , Podolsk : - In terms of Bohm 's Spin te Representation 1017¥14 1952 : EPR - Gleason 's Theorem 1957 : - HVT 's incompatible With Quantum Mechanics 's Paper Local Bell - 1964 : - - { Kochen Stecker ¥3 } { Begin ) Ak= Be for k&l 1968 : Now assume some observable to both sets ) f , ( some common An [ Ak ,AD=[ By ,BD= 0 € ¥% givg Ya a contradiction vectors This will With - you . Value of Be away , Where's the rub ? of Ak value = assumed ) ( ity ! Contextual . contextual . Variables must not be - Hidden non Moral . : common to all these things Gmposite Quantum Systems FKHAOHBQ He 1 × 7/+01923 QK )c is the possibility NDABE one . = IXDQ 19 , ) QKD QHD also Now linearity implies +1 × 27019<7 is legit state Butthis is Entangled in general

  2. � � ⇒ � ENTANGLEMENT = = §w ; QPOPPQPF Then Separable ; If PABE Otherwise Entangled - & F =D ( :-< 1 Wi OEW can be prepared locally Physical Realization � Separable States FOR BIPARTITE PURE STATES from Singular Value Decomposition Theorem one can always ; ; li )aQ IDB that = ?¥n Write this As 1 kayaked other basis ( Schmidt Decomposition ) in ¥ some - = , , t N=1 - Separable Iff But for multipartite states Schmidt Decomposition Can't be done unique - no - Is it Entangled ? Q ) Bipartite Systems : - - How much Entanglement ? If yes a) Entanglement Quantification in terms of Teleportation Protocol � Take Compare states in terms of their Entanglement singlet ( Original Benett Proto d) . . . other initial 100% Exact Teleportation ; But take states inexact teleportation some x ttrms of fidelity wrt target have a 100 ) tbli allowed state D. LOCC like = Suppose we a operation . . . . . Benett Under - al concentration et . . . Xp huffy entangled LOccep0n_oQmf.mcrDent@tm-nCsCsx.D Entangled Schumacher Noiseless Data Compression Theorem Allows the reverse process 'S ( Pa ) pure bipartite state - Easily Entanglement of a Calculable = States ? What about mixed Bipartite Distillable Two Defy 4 Entanglement - states of formation } # for mixed 2) Entanglement

  3. E P ; E ( IYDAB ) EOF HAD inf pure state decompositions Overall = p ⇒ =§P ; lyiapxynitl = nljmannt Distillable Entanglement do o# are hard to But these optimizations A PROPERTIES GOOD MEASURE OF ENTANGLEMENT MUST SATISFY @ Vanishes for Separable State - invariant @ LU Monotone decreasing under 0 LOCC Necessary but desirable @ Additivity , Convexity NOT , Continuity - ( like Six pack abs @ ) EOF is not Result ) ( Recent additive Hastings - Entanglement ( Winter ) One good candidate with all those properties - Squashed t But notoriously hard to calculate ( Winter if ) . NEGATIVITY LOG CONCURRENCE (2 × 2) Easy to Calculate - ÷ $ a¥x to Calculate / EoF= Simple te of Concurrence I NI ~ log = Negativity N ( under Partial Transpose ) Additive but not convex Cplenio ) Mutual Information on Quantum . So Good for Ckegyn Depends Entanglement Squashed . . - - ENTANGLEMENT DETECTION OF - Given PAB is it entangled / separable Prob ? : .

  4. Bdlviolalim -= Entanglement Initially people thought - . But for Werner States plate .lt#)IeifpzHrEntang1edPossibletohavenoBdlViolationevenwithEntamnfpe)ButP707o..7-BellViolation ' k#¥¥O¥iI¥ penetrate detector Ifyouhaveanoperatorwhichistvebutnotcrewdlserveasa t . Banach ) ( e.g. Transpose Operator ) ( Hahn - PPT PABTBI B Faare states Partial on Transpose 20 ieeiaffninoemnredeigenraeue EHAB FA=( } FE Parliairansposeona mutates 't . t Entangled for sure ButdoesPPT ⇒ ? Separable true 2 × 2,2 × 3 - -7 forhigher dimensional states Bound Entangled State False - ( Entangled but not distillable ) Example OFAPPT Entangled State in 3 × 3 systems l°k±'IaH Walks } mnextpmodiauheapsasi " " ' • i ⇒ oe¥± , H ¥610 > Existence of Bound Implies states - Entangled Here But Distillable >0 1. ¥ Bound Entangl EOF ,lqXql ) g=( - . . - ement Entanglement -0 Reduction Criteria Violation Distillable - � Either Separable ) Distillable - '* invariant states -=±+BP+[ states ] where uau Isotropic !¥g± , lv Definite form of rd Entanglement available invariant states -=1+BV[ Werner states ] UQU canbe Bound Criteria hesatisfies Reduction - Entangled

  5. T pABnifyoutind1UDofrank-2and@1paEWfOeDistillab1et7O-0necepyundislillebleHarlialTransposDamilarlyn.cepyundistillabilityTomorrowi-MuHiparliteEntang1ement.N ondassial Entanglement Correlations Beyond

Recommend

More recommend