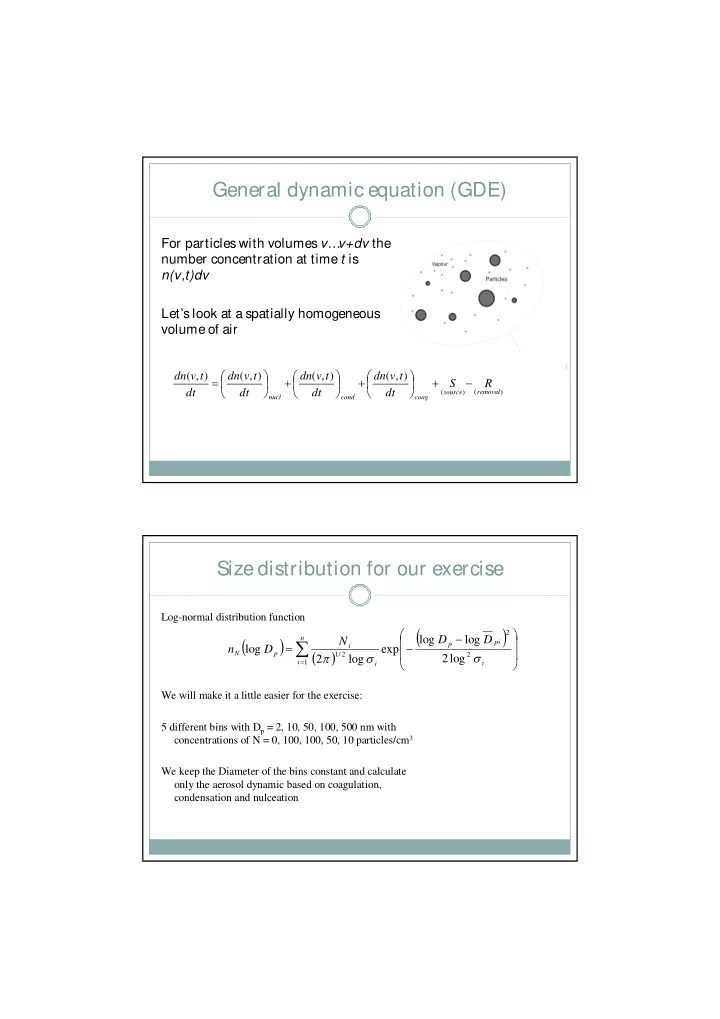

General dynamic equation (GDE) For particles with volumes v … v+dv the number concentration at time t is n(v,t)dv Let’s look at a spatially homogeneous volume of air � � � � � � dn ( v , t ) dn ( v , t ) dn ( v , t ) dn ( v , t ) � � � � � � � � � � � S R � � � � � � dt dt dt dt ( source ) ( removal ) nucl cond coag Size distribution for our exercise Log-normal distribution function � � � � 2 � � � log D log D n � � � N pi � � p i n log D exp � � � � N p � � � 1 / 2 2 2 log 2 log � � � i 1 i i We will make it a little easier for the exercise: 5 different bins with D p = 2, 10, 50, 100, 500 nm with concentrations of N = 0, 100, 100, 50, 10 particles/cm 3 We keep the Diameter of the bins constant and calculate only the aerosol dynamic based on coagulation, condensation and nulceation
Consider particles in volume range v… v+dv Coagulation
Coagulation Coagulation
Coagulation Coagulation in GDE Particles of volume v are produced by collision of two particles whose combined volume is v (we denote them u and v-u ). Particles of volume v are lost by collision with all sized particles. � � v dn 1 � � � � � � � ( u , v u ) n ( u ) n ( v u ) du � � dt 2 coag 0 � � � � ( u , v ) n ( u ) n ( v ) du 0
Coagulation coefficient Coagulation coefficient where k = 1,381 x 10 -23 J K -1 (Boltzmann constant)
Condensation Condensation
Condensation Condensation
Condensation in GDE Particles of volume v can form when smaller particles grow or larger particles shrink to size v . Particles of volume v are lost when condensation grows them to larger sizes or evaporation shrinks them to smaller sizes. dv � Let us denote q ( v ) dt � � � � � � Then n ( v , t ) � � � � q ( v , t ) n ( v , t ) � � � � t v cond Condensation flux Condensation flux of gas phase compound i onto a particle with diameter d p dv � � � � � 2 d D v ( d , )( c c ( d )) p i i p i i eq , i p dt 3 d � � � � � p lim 1 As and lim � � � 8 d d 0 p p dv � for small particles 2 d p dt dv � d for large particles p dt
Kelvin effect dv � � � � � 2 d D v ( d , )( c c ( d )) p i i p i eq , i p dt � � � 2 M � � � Ke exp � � � � RT R � p Exercise Particle diameter growth rates by our organic vapour and sulphric acid: Org_cond = 0.1 * � -pinene * (OH, O 3 , NO 3 ) Particle diameter growth rates by condensation of our organic vapour dD p /dt = 4 * m i * b i * D i * C / D p / r i C is the concentration of our condensing gas in molecules/cm 3 m i are the molecular mass (Org_cond = 136 g/mol, H 2 SO 4 = 98 g/mol) b i is a correction factor for the mass flux: 10 -13 D i are the diffusion coefficients: 0.2 cm 2 /s r i are the liquid density: 1 g/cm 3 After each time step we calculate the growth in comparison with our fixes size bins and assume that this growth fraction of the particles per second in % (multiplied by 100) are moved to the next size bin. If everything is correct in your calculation with gas concentrations of the condensing species in the range up to the power of 6-8 you should move 10 to 50 percent of the particles in one hour to the next bin for the smallest size.
Nucleation in GDE Nucleation forms new particles (typically) at only one size v* and at formation rate J(v,t). In our case we will use the nucleation rate proportional to sulphuric acid with J = A * [H 2 SO 4 ] with A = 10 -8 per second We include this particle in the lowest size bin and assume that all of them will grow to bigger sizes Aerosol removal 1) dry deposition 2) nucleation scavenging (~ in-cloud scavenging) wet deposition 3) impaction scavenging (~ below-cloud scavenging)
Dry deposition Close to the surface deposition flux F = -v d C Deposition velocity v d is most strongly affected by particle size forest and the roughness of the surface crop water Dry deposition velocity 1 � � v v � � d s r r r a b c where r a = aerodynamic resistance (determined by turbulence) r b = quasi-laminar layer resistance (determined by particle properties and surface characteristics) r c = canopy resistance (chemical reactions, perturbation of quasi-laminar layer) v s = particle settling velocity (determined by size) Resistance equations complex, several "simplified" parameterisations for large scale models available
Wet deposition CLOUD WATER Formation of cloud Chemical reactions Dissolution droplets; collisions Chemical reactions PARTICULATE AIR GAS EOUS AIR POLLUTION POLLUTION Collection by rain drops RAIN, S NOW Collection by rain drops Chemical reactions WET DEPOS ITION In-cloud scavenging Nucleation scavenging removes in practice all activated particles larger than a certain cut-off size. Large scale models typically use a cut-off ~100-300 nm dry size in grid boxes where precipitation is formed.
Below-cloud scavenging Scavenging minimum at accumulation mode –smaller particles collected due to Brownian motion – larger particles collected due to their inertia Scavenging rate � � � 2 ( d ) D U ( D ) E ( D , d ) N p drop t drop drop p drop 4 where collision efficiency is � � 3 / 2 � � � � � * 4 1 St S � � � � � � � � � 3 � � E 1 0 . 4 Re Sc 0 . 16 Re Sc 4 ( 1 2 Re ) � � � � � � � * � � Re Sc St S 2 / 3 Summary – Time evolution of atmospheric aerosol particles described with general dynamic equation (GDE) – Although mathematical formulations possible to find for all processes, it is usually impossible to solve all processes simultaneously � time splitting – Factors limiting accurate solution – incomplete information available (esp. emissions) – subgrid scale processes (clouds, emissions,… ) – impossible to describe continuous size distribution in a model (see next lecture!)
Recommend
More recommend