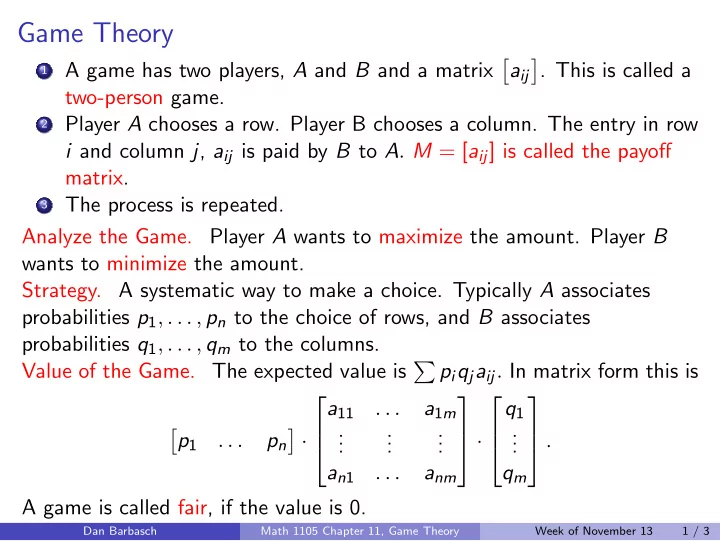
Game Theory 1 A game has two players, A and B and a matrix . - PowerPoint PPT Presentation

Game Theory 1 A game has two players, A and B and a matrix � � . This is called a a ij two-person game. 2 Player A chooses a row. Player B chooses a column. The entry in row i and column j , a ij is paid by B to A . M = [ a ij ] is called the payoff matrix. 3 The process is repeated. Analyze the Game. Player A wants to maximize the amount. Player B wants to minimize the amount. Strategy. A systematic way to make a choice. Typically A associates probabilities p 1 , . . . , p n to the choice of rows, and B associates probabilities q 1 , . . . , q m to the columns. Value of the Game. The expected value is � p i q j a ij . In matrix form this is     a 11 a 1 m q 1 . . . . . . . . . . . � � p 1 p n     . . . . ·  · . . .  .   a n 1 a nm q m . . . A game is called fair, if the value is 0. Dan Barbasch Math 1105 Chapter 11, Game Theory Week of November 13 1 / 3

Notions Zero Sum game No money enters from the outside. Whatever one player loses, the other gains. Fair Game Value is 0. Pure Strategy The player chooses a row/column consistently. The probabilites are 1 for one row/column, 0 for the others. Dominated Strategy A row Dominates another row if every entry in the one row is larger than the corresponding entry in the other row. Similar for columns. In each row, A wants the maximum. In each column B wants the minimum. Strictly Determined Games A Saddle Point is an entry which is largest in its column and smallest in its row. When there is a saddle point, the game is called strictly determined. Dan Barbasch Math 1105 Chapter 11, Game Theory Week of November 13 2 / 3

Examples I � 2 � 1 1 − 1 0 � 0 � 2 2 1 − 3  2 3 1  − 1 4 − 7   3   5 2 0   8 − 4 − 1  3 8 − 4 − 9  − 1 − 2 − 3 0  . 4  − 2 6 − 4 5  − 3 − 2 6  2 0 2 5   5 − 2 − 4 Dan Barbasch Math 1105 Chapter 11, Game Theory Week of November 13 3 / 3