Example: a digital option; for a digital call with strike K , 1 - PowerPoint PPT Presentation

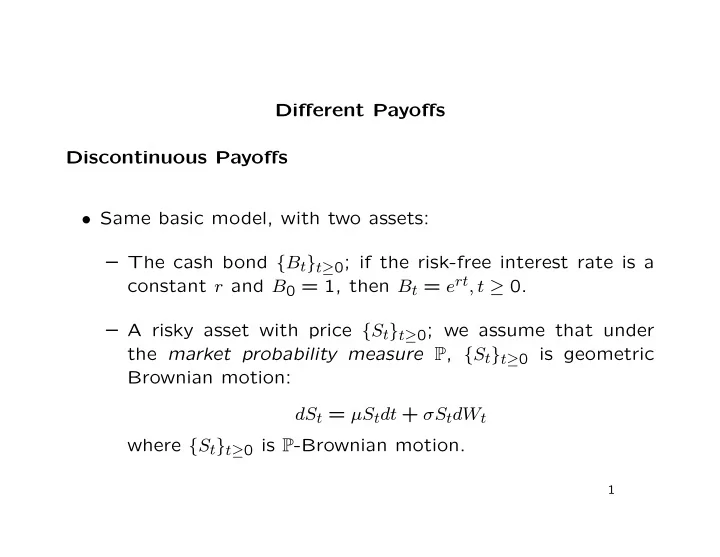
Different Payoffs Discontinuous Payoffs • Same basic model, with two assets: – The cash bond { B t } t ≥ 0 ; if the risk-free interest rate is a constant r and B 0 = 1, then B t = e rt , t ≥ 0. – A risky asset with price { S t } t ≥ 0 ; we assume that under the market probability measure P , { S t } t ≥ 0 is geometric Brownian motion: dS t = µS t dt + σS t dW t where { S t } t ≥ 0 is P -Brownian motion. 1

• Recall: – the value at time t of a European option whose payoff at time T is C T = f ( S T ) is V t = F ( t, S t ), where � V t = F ( t, S t ) = E Q � e − r ( T − t ) f ( S T ) � � F t � � ∞ r − σ 2 √ � �� � �� = e − r ( T − t ) −∞ f x exp ( T − t ) + σy T − t 2 × exp( − y 2 / 2) √ dy 2 π – The replicating portfolio consists of φ t = ∂F � � ∂x ( t, x ) � � x = S t shares and ψ t = e − rt ( V t − φ t S t ) cash bonds. 2

• The Feynman-Kac representation shows that F is continuous and differentiable for t ∈ [0 , T ) and x ∈ R . • But F ( t, x ) → f ( x ) as t → T , which is often not differentiable (calls and puts) and may not be continuous. • So F may behave badly as t → T . 3

• Example: a digital option; for a digital call with strike K ,  1 if S T ≥ K  C T = 0 if S T < K  • Calculus shows that V t = e − r ( T − t ) Φ( d 2 ) , where Φ is the standard normal cumulative distribution func- tion, and r − σ 2 � � � � 1 � S t � d 2 = σ √ T − t log + ( T − t ) 2 K is the same constant that arises in the price of a normal call. 4

• More calculus gives φ t = e − r ( T − t ) × 1 1 × � S t 2 π ( T − t ) σ 2 � 2   r − σ 2 � � � 1 � S t � × exp  − log + ( T − t )  2( T − t ) σ 2 K 2 • This is proportional to a lognormal density function in S t , � ( T − t ) σ 2 . centered approximately at K , with scale • As t approaches T , this is large for S t close to K , and small otherwise, so the hedge may need large adjustments if S t is close to K ; digital options are often booked as spreads to smooth the delta. 5

Multistage Options • Some option definitions involve a maturity T 1 and an inter- mediate time T 0 , 0 < T 0 < T 1 . • Example: forward start call option; payoff at t = T 1 is � � S T 1 − K + , where the strike K = S T 0 . • Work backwards: for T 0 ≤ t ≤ T 1 , it is a standard European call with (known) strike S T 0 , and � �� � V t = e − r ( T 1 − t ) E Q � � S T 1 − S T 0 � F t � + = S t Φ( d 1 ) − S T 0 e − r ( T 1 − t ) Φ( d 2 ) 6

• Note that d 1 and d 2 depend on S t , as well as K = S T 0 , r , σ , and T 1 . • But at t = T 0 , the option is “at the money”, and d 1 and d 2 depend only on r , σ , T 0 , and T 1 . • So Φ( d 1 ) − e − r ( T 1 − T 0 ) Φ( d 2 ) � � V T 0 = S T 0 = c ( r, σ, T 0 , T 1 ) S T 0 . • So for 0 ≤ t < T 0 , V t = c ( r, σ, T 0 , T 1 ) S t . 7

Compound options • Example: “Call on call”; the underlying is a call option on some asset, initiated at time T 0 > 0 and maturing at time T 1 > T 0 , with strike K 1 . • The compound option is the option to buy the underlying call at time T 0 , with a strike of K 0 . • The value of the underlying call, at time T 0 , is given by � � Black-Scholes, as a function of S T 0 : say C S T 0 , T 0 ; K 1 , T 1 . 8

• The value of the compound option at time T 0 is � � � � � � = S T 0 , T 0 ; K 1 , T 1 V S T 0 , T 0 C − K 0 + . • The value of the compound option at a time t < T 0 is the dis- counted expected value of C under the risk-neutral measure: E Q � e − r ( T 0 − t ) V � �� S T 0 , T 0 . • No closed form expression–the integral is computed numeri- cally. 9