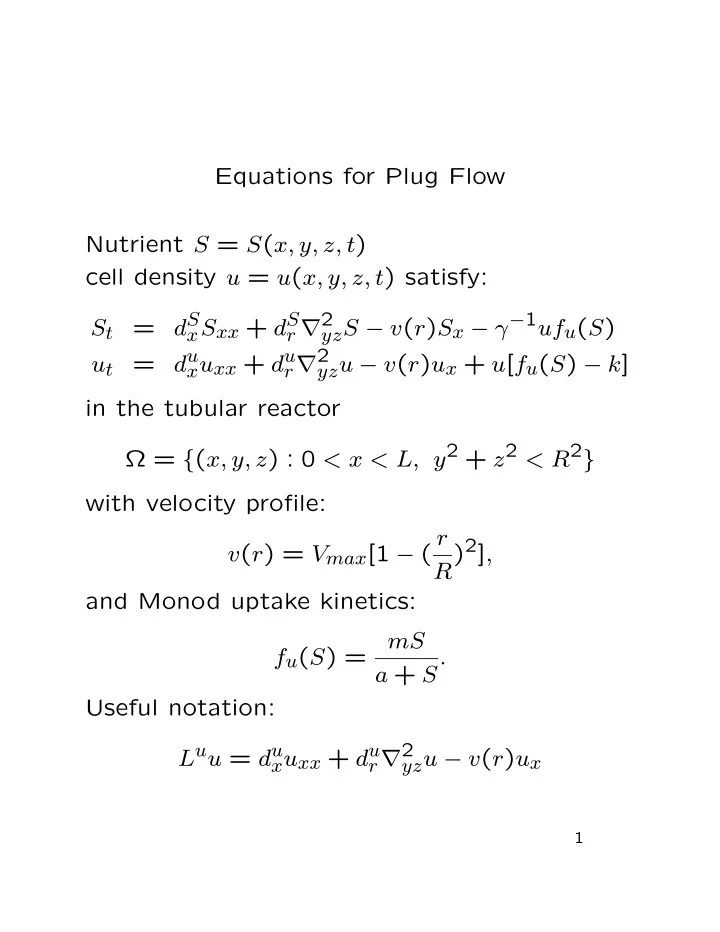
Equations for Plug Flow Nutrient S = S ( x, y, z, t ) cell density u - PDF document

Equations for Plug Flow Nutrient S = S ( x, y, z, t ) cell density u = u ( x, y, z, t ) satisfy: r ∇ 2 yz S − v ( r ) S x − γ − 1 uf u ( S ) d S x S xx + d S S t = r ∇ 2 d u x u xx + d u u t = yz u − v ( r ) u x + u [ f u ( S ) − k ] in the tubular reactor Ω = { ( x, y, z ) : 0 < x < L, y 2 + z 2 < R 2 } with velocity profile: v ( r ) = V max [1 − ( r R ) 2 ] , and Monod uptake kinetics: mS f u ( S ) = a + S. Useful notation: L u u = d u x u xx + d u r ∇ 2 yz u − v ( r ) u x 1

Danckwerts’ Boundary Conditions at x = 0: v ( r ) S 0 − d S = x S x + v ( r ) S − d u 0 = x u x + v ( r ) u, at x = L : d S x S x − v ( r ) S = − v ( r ) S, i.e., S x = 0 = 0 u x See R. Aris, ”Mathematical Modeling, a chem- ical engineers perspective”, Academic Press, 1999. 2

No Wall Growth Single Species in the fluid: L S S − γ − 1 uf u ( S ) = S t L u u + u [ f u ( S ) − k ] u t = at x = 0: v ( r ) S 0 − d S = x S x + v ( r ) S − d u 0 = x u x + v ( r ) u, at x = L : S x = u x = 0 on the wall r = R S r = 0 u r = 0 . 3

Radial Boundary Conditions ( r = R ) wall-attached bacterial fraction w = w ( x, R cos θ, R sin θ, t ) ∈ [0 , w max ] satisfies: w t = w [ f w ( S ) G ( W ) − k w − β ] + αu (1 − W ) , where W = w/w max . radial boundary conditions for S: − d S r S r = γ − 1 wf w ( S ) radial boundary conditions for u: − d u r u r = αu (1 − W ) − wf w ( S )[1 − G ( W )] − βw. 4

With Wall Growth wall-attached bacterial fraction on r = R w = w ( x, R cos θ, R sin θ, t ) ∈ [0 , w max ] satisfies: w t = w [ f w ( S ) G ( W ) − k w − β ] + αu (1 − W ) , where W = w/w max . radial boundary conditions for S: − d S r S r = γ − 1 wf w ( S ) radial boundary conditions for u: − d u r u r = αu (1 − W ) − wf w ( S )[1 − G ( W )] − βw. 5

Summary of Single-Population Model in the fluid: L S S − γ − 1 uf u ( S ) S t = L u u + u [ f u ( S ) − k ] u t = on the wall r = R w t = w [ f w ( S ) G ( W ) − k w − β ] + αu (1 − W ) . at x = 0: v ( r ) S 0 − d S = x S x + v ( r ) S − d u 0 = x u x + v ( r ) u, at x = L : S x = u x = 0 on the wall r = R − d S γ − 1 wf w ( S ) = r S r − d u r u r = αu (1 − W ) − w [ f w ( S )(1 − G ( W )) + β ] . 6

Many-Populations with Wall Growth in the fluid L S S − γ − 1 u i f ui ( S ) � S t = i i L i u i + u i [ f ui ( S ) − k i ] u i = t on the wall r = R γ − 1 − d S w i f wi ( S ) � r S r = i i − d i r u i α i u i (1 − W ) = r − w i [ f wi ( S )(1 − G i ( W )) + β i ] w i w i [ f wi ( S ) G i ( W ) − k wi − β i ] = t + α i u i (1 − W ) . i w i /w max . at x = 0 where W = � v ( r ) S 0 − d S = x S x + v ( r ) S − d i x u i x + v ( r ) u i , 0 = at x = L S x = u i x = 0 . 7

Linear Stability of Washout Steady State S ≡ S 0 , u ≡ 0 , w ≡ 0 . Linear stability analysis: S 0 + ǫ exp( λt )¯ S = S u = ǫ exp( λt )¯ u w = ǫ exp( λt ) ¯ w 0 < | ǫ | << 1, leads to the non-standard eigen- value problem L S ¯ S − γ − 1 ¯ uf u ( S 0 ) λ ¯ = S L u ¯ u [ f u ( S 0 ) − k ] λ ¯ = u + ¯ u w [ f w ( S 0 ) G (0) − k w − β ] + α ¯ λ ¯ w = ¯ u with homogeneous Danckwerts’ b.c. ( x = 0 , L ) and radial b.c. on r = R : S r + γ − 1 ¯ d S wf w ( S 0 ) r ¯ 0 = d u w [ f w ( S 0 )(1 − G (0)) + β ] . 0 = r ¯ u r + α ¯ u − ¯ 8

Principal Eigenvalue There exists a real simple eigen- Theorem: value λ ∗ > f w ( S 0 ) G (0) − k w − β belonging to the interval with endpoints: L f w ( S 0 ) − k w , f u ( S 0 ) − k − λ V max where − λ < 0 is the principal eigenvalue of the (scaled ¯ x = x/L, ¯ r = r/R ) eigenvalue problem: r 2 ) u ¯ r − 1 (¯ λu = θ x u ¯ x − (1 − ¯ x + θ r ¯ ru ¯ r ) ¯ r , x ¯ r 2 ) u, 0 = − θ x u ¯ x + (1 − ¯ ¯ x = 0 0 = u ¯ x , ¯ x = 1 u ¯ = 0 , r = 1 , ¯ r θ x = ( d u x /L 2 )( L/V max ) , θ r = ( d u r /R 2 )( L/V max ). Corresponding to λ ∗ is an eigenvector (¯ S, ¯ u, ¯ w ) satisfying ¯ S < 0, ¯ u > 0 in Ω and ¯ w > 0 in r = R . If λ ∗ < 0 then washout is stable in the linear approximation; if λ ∗ > 0 then it is unstable. 9

Global Stability of Washout Theorem: If both L f u ( S 0 ) − k − λ < 0 , f w ( S 0 ) − k w < 0 , V max then λ ∗ < 0 and � � t →∞ ( lim Ω udV + r = R wdA ) = 0 . Conjecture: The result remains valid if only λ ∗ < 0. 10

Population steady state The equations for a steady state are L S S − γ − 1 uf u ( S ) 0 = L u u + u [ f u ( S ) − k ] , 0 = in Ω 0 = w [ f w ( S ) G ( W ) − k w − β ] + αu (1 − W ) , r = R. Danckwerts’ boundary conditions at x = 0 , L and radial boundary conditions: d S − γ − 1 wf w ( S ) r S r = d u r u r = − αu (1 − W ) + w [ f w ( S )(1 − G ( W )) + β ] . Theorem: Let λ ∗ > 0 and f w ( S 0 ) G (0) − k w − β � = 0. Then there exists a radially symmet- ric steady state solution ( S, u, w ) satisfying (in cylindrical coordinates) 0 < S ( x, r ) ≤ S 0 , u ( x, r ) > 0 , and 0 < w ( x ) ≤ w max . 11

Criterion for Survival λ ∗ > 0 if both f w ( S 0 ) − k w > 0 and L f u ( S 0 ) − k − λ > 0 V max hold, or if f w ( S 0 ) G (0) − k w − β > 0 holds. In case of no wall growth ( α = w = 0), L λ ∗ = f u ( S 0 ) − k − λ V max so middle inequality suffices for survival. 12

Effects of Influx of Antibiotic Concentration A = A ( x, y, z, t ) satisfies: d A x A xx + d A r ∇ 2 A t = yz A − v ( r ) A x d A 0 = r A r , r = R (impenetrable biofilm) v ( r ) A 0 − d A = x A x + v ( r ) A, x = 0 (influx of A) 0 = x = L. A x , As for substrate in absence of bacteria, A ( x, y, z, t ) → A 0 , t → ∞ . If planktonic cell death rate k = k ( A 0 ) , k ′ > 0, then effect on λ ∗ is minimal since: f w ( S 0 ) G (0) − k w − β < λ ∗ where we assume adherent cell death rate k w independent of A . Contrast to case of no wall growth ( α = w = 0) where L λ ∗ = f u ( S 0 ) − k ( A 0 ) − λ. V max 13

A pair of eigenvalue problems L i u + au, λu = Ω λw = bw + αu, r = R 0 = d r u r + αu − cw, r = R (1) 0 = − d x u x + v ( r ) u, x = 0 0 = x = L u x , The corresponding adjoint problem is given by: λu = L i u + au, Ω λw = bw + cu, r = R 0 = d r u r + αu − αw, r = R (2) 0 = d x u x + v ( r ) u, x = L 0 = x = 0 u x , here, a, b, c, α are real constants. 14

In order to see in what sense (2) is adjoint to (1) we make the following observation. Proposition Let u ∈ C 2 (Ω) ∩ C 1 (Ω) satisfy the Danckw- erts’ boundary conditions at x = 0 , L , ˆ u ∈ C 2 (Ω) ∩ C 1 (Ω) satisfy the adjoint Danckwerts’ boundary conditions at x = 0 , L , u, w satisfy the inhomogeneous radial boundary condition h = d r u r + αu − cw, r = R and ˆ u, ˆ w satisfy the homogeneous adjoint radial boundary condition in (2). Then we have � � Ω ( L i u )ˆ udV + r = R ( bw + αu ) ˆ wdA � � = Ω ( L i ˆ u ) udV + r = R h ˆ u + w ( b ˆ w + c ˆ u ) dA If h ≡ 0, then we obtain the adjoint relation of (2) and (1). 15

Principal Eigenvalue Theorem Let α, c > 0. Then there exists a real simple eigenvalue λ ∗ > b of (1) satisfying: b + c < λ ∗ ≤ a − λ i , if b + c < a − λ i b + c = λ ∗ , if b + c = a − λ i a − λ i < λ ∗ < b + c, if b + c > a − λ i Corresponding to eigenvalue λ ∗ is an eigenvec- tor (¯ u, ¯ w ) satisfying ¯ u > 0 in Ω and ¯ w > 0 in r = R . If λ is any other eigenvalue of (1) cor- responding to an eigenvector ( u, w ) ≥ 0, then λ = λ ∗ and ( u, w ) = c (¯ u, ¯ w ) for some c > 0. ¯ u, ¯ w are axially symmetric, i.e., in cylindrical coordinates ( r, θ, x ), ¯ u = ¯ u ( r, x ) , ¯ w = ¯ w ( x ). λ ∗ is also an eigenvalue of (2) corresponding to an eigenvector ( u, w ) = ( ψ, χ ). Moreover, ( ψ, χ ) has the same uniqueness up to scalar multiple, positivity and symmetry properties as does (¯ u, ¯ w ). 16

bacterial growth is limited by supplied substrate Let ( ψ i , χ i ) be the PEV corresponding to the eigenvalue λ i of (2) in the case that a = 0 , b = − β i , α = α i , c = β i , d r = d i r , d x = d i x . Normalize ( ψ i , χ i ) by requiring ψ i , χ i ≤ φ ≤ 1. By PEV Theorem and the fact that b + c = 0, we have λ i < 0. Theorem: A Priori Estimates S ( t, x, y, z ) ≤ S 0 , lim sup t →∞ uniformly in ( x, y, z ) ∈ Ω and � � γ − 1 Ω u i ψ i dV � lim sup t →∞ ( Ω SφdV + [ i i � r = R w i χ i dA ]) + 2 πS 0 � R 0 rv ( r ) dr ≤ min j { λ S , − λ j + k j , − λ j + k wj } 17