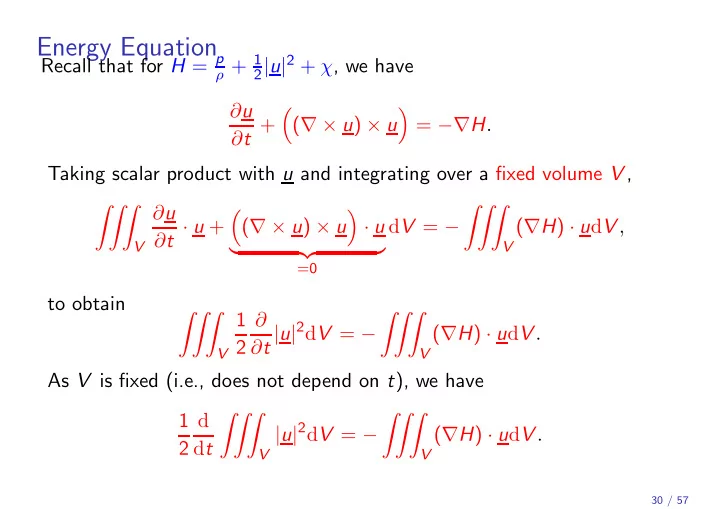
Energy Equation 2 | u | 2 + , we have Recall that for H = p + 1 - PowerPoint PPT Presentation

Energy Equation 2 | u | 2 + χ , we have Recall that for H = p ρ + 1 � � ∂ u ∂ t + ( ∇ × u ) × u = −∇ H . Taking scalar product with u and integrating over a fixed volume V , ��� ��� � � ∂ u ∂ t · u + ( ∇ × u ) × u · u d V = − ( ∇ H ) · u d V , V V � �� � =0 to obtain ��� ��� 1 ∂ ∂ t | u | 2 d V = − ( ∇ H ) · u d V . 2 V V As V is fixed (i.e., does not depend on t ), we have ��� ��� 1 d | u | 2 d V = − ( ∇ H ) · u d V . 2 d t V V 30 / 57

Energy Equation But, ∇ · ( Hu ) = H ∇ · u + u · ∇ H ; and also H ∇ · u = 0 as ∇ · u = 0 due to incompressibility. Thus ��� ��� 1 d | u | 2 d V = − ∇ · ( Hu ) d V , 2 d t V V and using the divergence theorem we obtain ��� �� 1 d | u | 2 d V = Hu · n d S . 2 d t V S Multiplying through by ρ , we deduce the energy equation ��� �� 1 ( p + 1 d 2 ρ u 2 + ρχ ) u · n d S . ρ | u | 2 d V = 2 d t V S 31 / 57

Vorticity We define the vorticity ω ω = ∇ × u . BIG IDEA: Vorticity measures the rotation of the flow at each point. Note: Recall the definition of curl: vorticity is the curl of the velocity! A flow is called irrotational if 0 = ω = ∇ × u 32 / 57

Bernoulli Equations (again) Recall the momentum equation (in curl form) ∂ u ∂ t + ( ∇ × u ) × u = −∇ H Suppose that the flow is steady and irrotational hence ∂ u ∂ t = 0 and ω = ∇ × u = 0 giving ∇ H = 0 H is constant throughout the whole flow field if the fluid is in steady and irrotational flow. 33 / 57

Vorticity Equations The momentum equation reads ∂ u ∂ t + ( ∇ × u ) × u = −∇ H � �� � = ω Taking curl, we obtain ∂ω ∂ t + ∇ × ( ω × u ) = ∇ × ∇ H = 0 Using the identity [Q1 Problem Sheet 9] ∇ × ( ω × u ) = ( u · ∇ ) ω − ( ω · ∇ ) u + ω ( ∇ · u ) − u ( ∇ · ω ) Using the identity ∇ × ( ω × u ) = ( u · ∇ ) ω − ( ω · ∇ ) u + ω ( ∇ · u ) − u ( ∇ · ω ) � �� � � �� � =0 incompressible =0 ∇·∇× u =0 we obtain ∂ω d ω ∂ t + ( u · ∇ ) ω = ( ω · ∇ ) u or d t = ( ω · ∇ ) u Vorticity equations 34 / 57

Vorticity Equations Vorticity equations d ω d t = ( ω · ∇ ) u BIG IDEA: Pressure is eliminated! Indeed, the vorticity equation involves u and ω only; these are related by ω = ∇ × u . 35 / 57