EFFECTS OF SURFACE TREATMENTS WITH FLAME PLASMA AND SILANE ON - PDF document

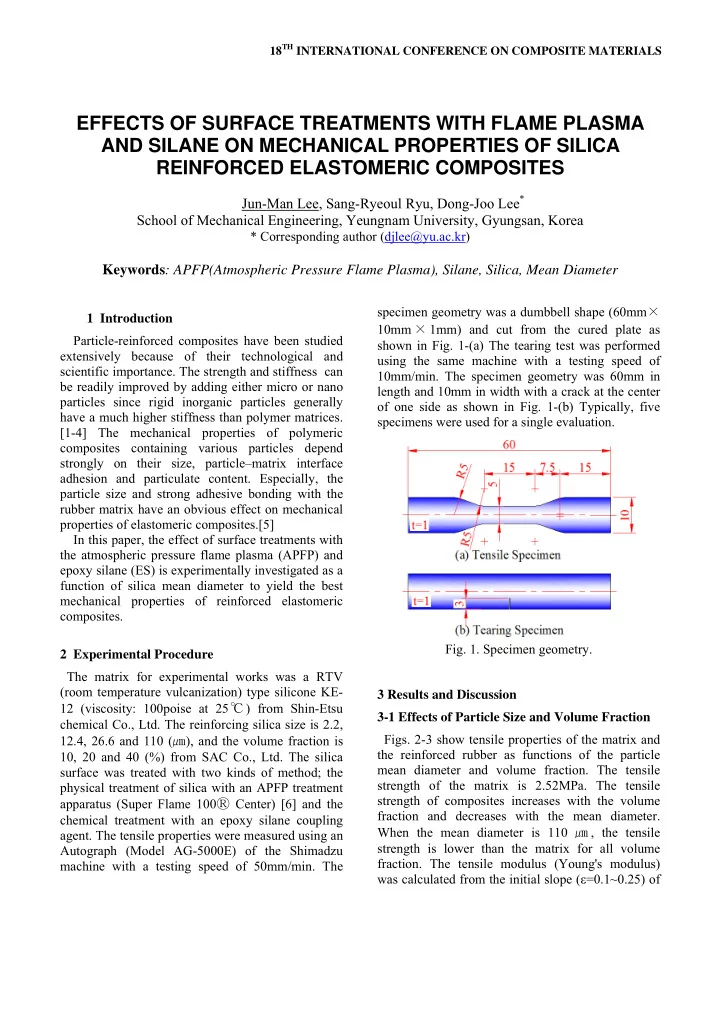
18 TH INTERNATIONAL CONFERENCE ON COMPOSITE MATERIALS EFFECTS OF SURFACE TREATMENTS WITH FLAME PLASMA AND SILANE ON MECHANICAL PROPERTIES OF SILICA REINFORCED ELASTOMERIC COMPOSITES Jun-Man Lee, Sang-Ryeoul Ryu, Dong-Joo Lee * School of Mechanical Engineering, Yeungnam University, Gyungsan, Korea * Corresponding author (djlee@yu.ac.kr) Keywords : APFP(Atmospheric Pressure Flame Plasma), Silane, Silica, Mean Diameter specimen geometry was a dumbbell shape (60mm × 1 Introduction 10mm × 1mm) and cut from the cured plate as Particle-reinforced composites have been studied shown in Fig. 1-(a) The tearing test was performed extensively because of their technological and using the same machine with a testing speed of scientific importance. The strength and stiffness can 10mm/min. The specimen geometry was 60mm in be readily improved by adding either micro or nano length and 10mm in width with a crack at the center particles since rigid inorganic particles generally of one side as shown in Fig. 1-(b) Typically, five have a much higher stiffness than polymer matrices. specimens were used for a single evaluation. [1-4] The mechanical properties of polymeric composites containing various particles depend strongly on their size, particle–matrix interface adhesion and particulate content. Especially, the particle size and strong adhesive bonding with the rubber matrix have an obvious effect on mechanical properties of elastomeric composites.[5] In this paper, the effect of surface treatments with the atmospheric pressure flame plasma (APFP) and epoxy silane (ES) is experimentally investigated as a function of silica mean diameter to yield the best mechanical properties of reinforced elastomeric composites. Fig. 1. Specimen geometry. 2 Experimental Procedure The matrix for experimental works was a RTV (room temperature vulcanization) type silicone KE- 3 Results and Discussion 12 (viscosity: 100poise at 25 ℃ ) from Shin-Etsu 3-1 Effects of Particle Size and Volume Fraction chemical Co., Ltd. The reinforcing silica size is 2.2, Figs. 2-3 show tensile properties of the matrix and 12.4, 26.6 and 110 ( ㎛ ), and the volume fraction is the reinforced rubber as functions of the particle 10, 20 and 40 (%) from SAC Co., Ltd. The silica mean diameter and volume fraction. The tensile surface was treated with two kinds of method; the strength of the matrix is 2.52MPa. The tensile physical treatment of silica with an APFP treatment strength of composites increases with the volume apparatus (Super Flame 100 Ⓡ Center) [6] and the fraction and decreases with the mean diameter. chemical treatment with an epoxy silane coupling When the mean diameter is 110 ㎛ , the tensile agent. The tensile properties were measured using an strength is lower than the matrix for all volume Autograph (Model AG-5000E) of the Shimadzu fraction. The tensile modulus (Young's modulus) machine with a testing speed of 50mm/min. The was calculated from the initial slope ( ε =0.1~0.25) of

the stress-strain curve. The tensile modulus of matrix is 0.88MPa. That of composites increases 10% 14 20% with the volume fraction, and indicates the 40% maximum when the mean diameter is 26.6 ㎛ . For Tear Energy (N-mm) 12 Matrix(6.84) the size larger than 26.6 ㎛ , the tensile modulus 10 decreases slightly. Fig. 4 shows the result of tearing test. The tear energy of elastomeric composites is 8 calculated by integrating the area of the load- displacement curve. The tear energy of the matrix is 6 6.84N-mm, and the composites with silica increase with the volume fraction and mean diameter. 4 However, the cases for 2.2 and 12.4 ㎛ with the 0 20 40 60 80 100 120 volume fraction of 10% show a lower value in Mean Diameter (  m) comparison with the matrix. Fig. 4. Effects of mean diameter and volume fraction on the tear energy of reinforced rubbers. 4.0 10% 20% 3-2 Effects of Surface Treatments Tensile Strength (MPa) 3.6 40% Matrix(2.52) Fig. 5 shows tensile stress-strain curves of the 3.2 matrix and silica (26.6 ㎛ , 40%) reinforced rubbers. 2.8 The rupture strain of composites is greatly reduced, and the stress of those is significantly increased at 2.4 low strain region when it compared to the matrix. Also, the mechanical properties with APFP (‘P’) or 2.0 epoxy silane (‘ES’) treatment tend to increase 1.6 compared to the untreated. Figs. 6-7 show the tensile 0 20 40 60 80 100 120 strength and tensile modulus with different the mean Mean Diameter (  m) diameters at the volume fraction of 40%. The tensile Fig. 2. Effects of mean diameter and volume fraction strength of composites shows the maximum value on the tensile strength of reinforced rubbers. when the mean diameter is 2.2 ㎛ , and that of composites decreases with increasing the mean diameter. Also, the tensile strength of composites 2.4 with APFP and ES treated silica is higher in the 10% 20% Tensile Modulus (MPa) 2.1 order of 5~9% and 10~12%, respectively. The 40% tensile modulus of composite increases with Matrix(0.88) 1.8 increasing the mean diameter and shows the maximum value at 26.6 ㎛ . The tensile modulus of 1.5 silica reinforced composites with APFP and ES 1.2 treated is increased 14~22% and 21~26%, respectively. Fig. 8 shows the result of the tear 0.9 energy as a function of the mean diameter. The tear energy of composites increases with increasing 0.6 0 20 40 60 80 100 120 mean diameter compared with the matrix, because of Mean Diameter (  m) the crack bias of around particles and the increasing Fig. 3. Effects of mean diameter and volume fraction energy loss in the cracked region. The tearing energy on the tensile modulus of reinforced rubbers. of silica reinforced composites with APFP and ES treated is increased 11~13% and 12~15%, respectively.

PAPER TITLE The mechanical properties of silica reinforced composites with the surface treatment greatly 2.7 improved. Especially, the tensile modulus which is 2.4 Tensile Modulus (MPa) mostly affected by interface adhesion increased significantly. Fig. 9 reveals the rate of the tensile 2.1 modulus increase as functions of mean diameter and 1.8 surface treatments. The rate of that for both APFP 1.5 and ES treatments is increased with increasing mean 'P' Treated diameter. It seems that the case for a large mean 1.2 'ES' Treated Untreated diameter has a good dispersion in the matrix. During Matrix(0.88) 0.9 the APFP treatment, the support plate was shaken automatically for better treatment. The particle with 0.6 0 20 40 60 80 100 120 the large diameter was easy to shake. However, the Mean Diameter (  m) smaller one was difficult to shake uniformly. Fig. 7. Effects of mean diameter and surface Therefore, it is believed that it is necessary to review treatments on the tensile modulus of reinforced the amplitude and frequency of the shaker for the further improvement of mechanical properties. rubbers. 16 3.5 3.0 Tear Energy (N-mm) 14 2.5 Stress ( MPa ) 12 2.0 1.5 10 'P' Treated 1.0 'ES' Treated Matrix 8 Untreated Untreated 0.5 Matrix(6.84) 'P' Treated 6 'ES' Treated 0.0 0 20 40 60 80 100 120 Mean Diameter (  m) 0 1 2 3 Fig. 8. Effects of mean diameter and surface Srtrain treatments on the tear energy of reinforced rubbers. Fig. 5. Stress-strain curves of the matrix and silica reinforced rubbers. 40 4.0 'P' Treated Rate of T.M. Increase (%) 'ES' Treated 'P' Treated Regr 'ES' Treated Tensile Strength (MPa) 30 Untreated 3.5 Matrix(2.52) 20 3.0 10 2.5 0 2.0 0 20 40 60 80 100 120 0 20 40 60 80 100 120 Mean Diameter (  m) Mean Diameter (  m) Fig. 6. Effects of mean diameter and surface Fig. 9. Effects of mean diameter and surface treatments on the tensile strength of reinforced treatments on the rate of tensile modulus increase. rubbers. 3