= edge edge ( (u,v u,v) ) is not in is not in E E f x Y ( , - PDF document

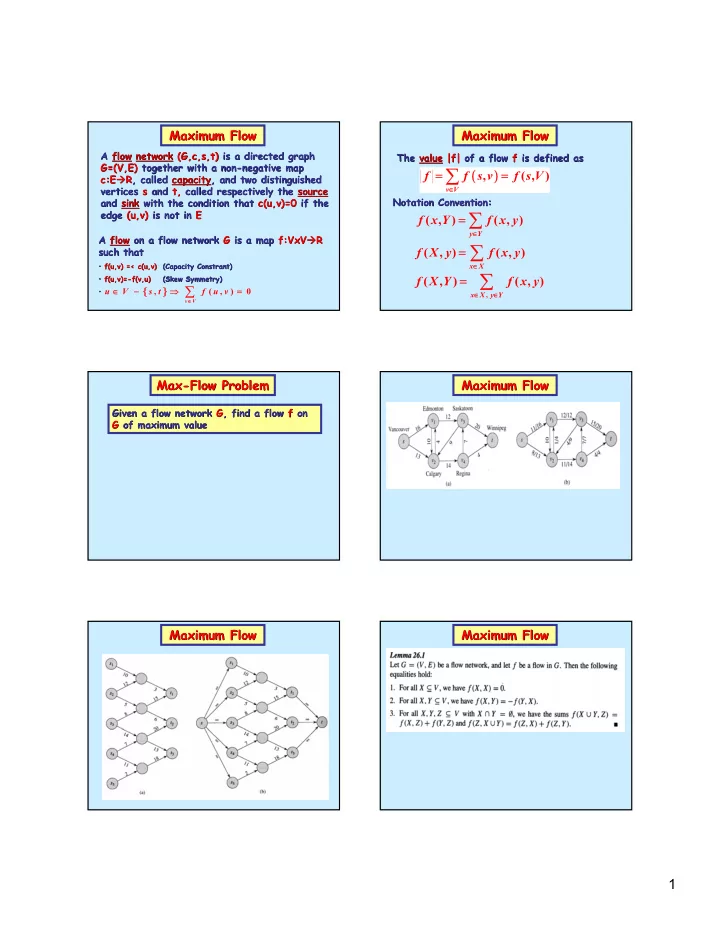
Maximum Flow Maximum Flow Maximum Flow Maximum Flow A A flow flow network network ( (G,c,s,t G,c,s,t) ) is a directed graph is a directed graph The value value |f| |f| of a flow of a flow f f is defined as is defined as The G=(V,E) together with a non G=(V,E) together with a non- -negative map negative map ∑ ( ( ) ) = = = = f f s v , f s V ( , ) c:E c:E � � R R, called , called capacity capacity, and two distinguished , and two distinguished vertices s vertices s and and t, t, called respectively the called respectively the source source ∈ v V Notation Convention: Notation Convention: and sink and sink with the condition that with the condition that c(u,v c(u,v)=0 )=0 if the if the = ∑ edge edge ( (u,v u,v) ) is not in is not in E E f x Y ( , ) f x y ( , ) ∈ y Y A A flow flow on a flow network on a flow network G G is a map is a map f:VxV f:VxV � � R R = ∑ such that such that f X y ( , ) f x y ( , ) • • f(u,v f(u,v) =< ) =< c(u,v c(u,v) ) (Capacity (Capacity Constrant Constrant) ) ∈ x X = ∑ • f(u,v • f(u,v)= )=- -f(v,u f(v,u) ) (Skew Symmetry) (Skew Symmetry) f X Y ( , ) f x y ( , ) ∑ { } ∈ ∈ − − ⇒ = • • u V s t , f ( u v , ) 0 ∈ ∈ ∈ ∈ x X y Y , ∈ v V Max- Max -Flow Problem Flow Problem Maximum Flow Maximum Flow Given a flow network Given a flow network G G, find a flow , find a flow f f on on G G of maximum value of maximum value Maximum Flow Maximum Flow Maximum Flow Maximum Flow 1

Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow 2

Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow Maximum Flow 3

Maximum Flow Maximum Flow 4