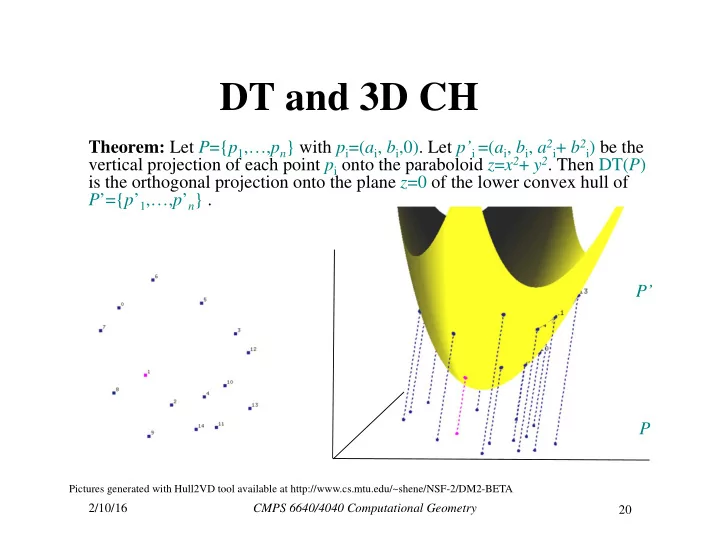
dt and 3d ch
play

DT and 3D CH Theorem: Let P ={ p 1 ,, p n } with p i =( a i , b i - PowerPoint PPT Presentation

Feb 28, 2024 •229 likes •280 views

DT and 3D CH Theorem: Let P ={ p 1 ,, p n } with p i =( a i , b i ,0). Let p i =( a i , b i , a 2 i + b 2 i ) be the vertical projection of each point p i onto the paraboloid z = x 2 + y 2 . Then DT( P ) is the orthogonal projection onto the

  1. DT and 3D CH Theorem: Let P ={ p 1 ,…, p n } with p i =( a i , b i ,0). Let p’ i =( a i , b i , a 2 i + b 2 i ) be the vertical projection of each point p i onto the paraboloid z = x 2 + y 2 . Then DT( P ) is the orthogonal projection onto the plane z =0 of the lower convex hull of P ’={ p ’ 1 ,…, p ’ n } . P’ P Pictures generated with Hull2VD tool available at http://www.cs.mtu.edu/~shene/NSF-2/DM2-BETA 2/10/16 CMPS 6640/4040 Computational Geometry 20

  2. DT and 3D CH Theorem: Let P ={ p 1 ,…, p n } with p i =( a i , b i ,0). Let p’ i =( a i , b i , a 2 i + b 2 i ) be the vertical projection of each point p i onto the paraboloid z = x 2 + y 2 . Then DT( P ) is the orthogonal projection onto the plane z =0 of the lower convex hull of P ’={ p ’ 1 ,…, p ’ n } . Pictures generated with Hull2VD tool available at http://www.cs.mtu.edu/~shene/NSF-2/DM2-BETA 2/10/16 CMPS 6640/4040 Computational Geometry 21

  3. DT and 3D CH Theorem: Let P ={ p 1 ,…, p n } with p i =( a i , b i ,0). Let p’ i =( a i , b i , a 2 i + b 2 i ) be the vertical projection of each point p i onto the paraboloid z = x 2 + y 2 . Then DT( P ) is the orthogonal projection onto the plane z =0 of the lower convex hull of P ’={ p ’ 1 ,…, p ’ n } . Pictures generated with Hull2VD tool available at http://www.cs.mtu.edu/~shene/NSF-2/DM2-BETA 2/10/16 CMPS 6640/4040 Computational Geometry 22

  4. DT and 3D CH Theorem: Let P ={ p 1 ,…, p n } with p i =( a i , b i ,0). Let p’ i =( a i , b i , a 2 i + b 2 i ) be the vertical projection of each point p i onto the paraboloid z = x 2 + y 2 . Then DT( P ) is the orthogonal projection onto the plane z =0 of the lower convex hull of P ’={ p ’ 1 ,…, p ’ n } . p' i, p ’ j, p ’ k form a (triangular) face of LCH( P ’).  The plane through p ’ i, p ’ j, p ’ k leaves all remaining points of P property above it. of unit  paraboloid The circle through p i, p j, p k leaves all remaining points of P in its exterior.  p i, p j, p k form a triangle of DT( P ). Slide adapted from slides by Vera Sacristan. 2/10/16 CMPS 6640/4040 Computational Geometry 23

Recommend

More recommend