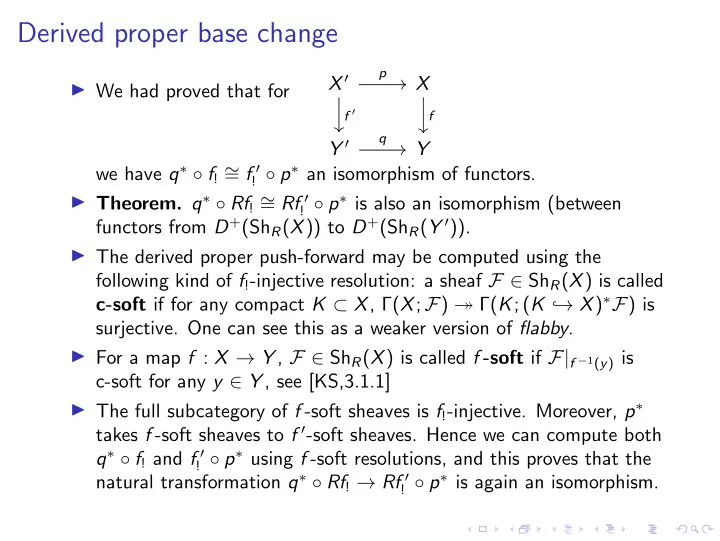

Derived proper base change p X ′ X ◮ We had proved that for f ′ f q Y ′ Y we have q ∗ ◦ f ! ∼ ! ◦ p ∗ an isomorphism of functors. = f ′ ◮ Theorem. q ∗ ◦ Rf ! ∼ ! ◦ p ∗ is also an isomorphism (between = Rf ′ functors from D + (Sh R ( X )) to D + (Sh R ( Y ′ )). ◮ The derived proper push-forward may be computed using the following kind of f ! -injective resolution: a sheaf F ∈ Sh R ( X ) is called c-soft if for any compact K ⊂ X , Γ( X ; F ) ։ Γ( K ; ( K ֒ → X ) ∗ F ) is surjective. One can see this as a weaker version of flabby . ◮ For a map f : X → Y , F ∈ Sh R ( X ) is called f -soft if F| f − 1 ( y ) is c-soft for any y ∈ Y , see [KS,3.1.1] ◮ The full subcategory of f -soft sheaves is f ! -injective. Moreover, p ∗ takes f -soft sheaves to f ′ -soft sheaves. Hence we can compute both q ∗ ◦ f ! and f ′ ! ◦ p ∗ using f -soft resolutions, and this proves that the natural transformation q ∗ ◦ Rf ! → Rf ′ ! ◦ p ∗ is again an isomorphism.
Verdier duality ◮ For convenience, let us now work with sheaves of Z -modules, and write Sh Z ( X ) = Sh( X ). One can work with R -modules with R of finite global dimension. But Cheng-Chiang refuses to. ◮ The wonderful Verdier duality starts with a seemingly innocent abstract concept that Rf ! has a right adjoint for a nice map f : X → Y (e.g. continuous function between finite-dimensional CW-complexes). ◮ In other words, there is a functor f ! : D + (Sh( Y )) → D + (Sh( X )) so that there are natural isomorphisms Hom D + (Sh( Y )) ( Rf ! F , G ) = Hom D + (Sh( X )) ( F , f ! G ) for all F ∈ D + (Sh( X )), G ∈ D + (Sh( Y )).
Poincar´ e duality Hom D + (Sh( Y )) ( Rf ! F , G ) = Hom D + (Sh( X )) ( F , f ! G ) ◮ Suppose we are in a simple case for which X is a manifold and Y is a point so we identify Sh( Y ) = Ab . Let F = Q X [ n ] and G = Q . We have c ( X ; Q )[ n ] , Q ) = Hom D + (Sh( X )) ( Q X , f ! Q ) Hom D + ( Ab ) ( H ∗ ◮ We have Hom D + ( Ab ) ( H ∗ c ( X ; Q )[ n ] , Q ) = Hom( H n c ( X ; Q ) , Q ). ◮ What is Hom D + (Sh( X )) ( Q X [ n ] , f ! Q )? One probably expects Hom D + (Sh( X )) ( Q X , f ! Q ) = H − n ( X ; f ! Q ) (i.e. Rf ∗ ( f ! Q )) no matter what kind of complex of sheaves f ! Q is. Suppose this is true. ◮ Together, this reads Hom( H n c ( X ; Q ) , Q ) = H − n ( X ; f ! Z ). Hmmm ... ◮ If X is orientable, we better have f ! Q = Q X [dim X ] so that H − n ( X ; f ! Q ) = H dim X − n ( X ; Q ) and this is Poincar´ e duality!
Proof of Verdier duality, I Hom D + (Sh( Y )) ( Rf ! F , G ) = Hom D + (Sh( X )) ( F , f ! G ) ◮ So we would like to prove Verdier duality; the existence of the right adjoint f ! . ◮ Our proof follow [KS, § 3.1] and partially a note of Akhil Mathew. ◮ In particular, we want to represent the functor F �→ Hom D + (Sh( Y )) ( Rf ! F , G ) for any G ∈ Sh( Y ). ◮ Replacing Rf ! by Rf ∗ makes this impossible, because even f ∗ does not commute with infinite direct sum! ◮ Suppose for the moment that every object in whatever category we work on is injective and projective. Then it looks like f ! G can be the sheaf with f ! G ( X ) = Hom D + (Sh( Y )) ( f ! Z X , G ) and in general f ! G ( U ) = Hom D + (Sh( Y )) (( f | U ) ! Z U , G ). ◮ This doesn’t make much sense. But we can derive all of them.
Proof of Verdier duality, II Suppose for the moment that every object in whatever category we work on is injective and projective. Then f ! G = Hom D + (Sh( Y )) ( f ! Z X , G )? ◮ Recall that a sheaf F ∈ Sh R ( X ) is c-soft if for any compact K ⊂ X , Γ( X ; F ) ։ Γ( K ; ( K ֒ → X ) ∗ F ) is surjective. A sheaf F is called f -soft if F| f − 1 ( y ) is c-soft for all y ∈ Y . ◮ Lemma. Suppose there is an integer n such that R i f ! F = 0 for all j > n , F ∈ Sh( X ). Then for any exact sequence K 0 → K 1 → ... → K n → 0 in Sh( X ) such that K 0 , K 1 , ..., K n − 1 are all f -soft, we have K n is f -soft as well. ◮ Now let us suppose it is true that R i f ! F = 0 for all j > n ; we will prove that if X is an n -dimensional CW-complex, then this is true. ◮ Let 0 → Z X → I 0 → I 1 → I 2 → ... → I n − 1 → I n → ... be a flabby resolution of flat Z -modules. This can be done as follows: I 0 is the direct product of all the stalks of Z X , where each stalk is realized as a skyscraper sheaf at the same point. After that I 1 is the direct product of all the stalks of the cokernel Z X → I 0 . One checks by induction that the sheaf constructed in each step has flat (i.e. torsion-free) stalks.
Proof of Verdier duality, III Let 0 → Z X → I 0 → I 1 → I 2 → ... → I n − 1 → I n → ... be a flabby resolution of flat Z -modules. ◮ Let K n := Im( I n − 1 → I n ) so we have exact sequence 0 → Z X → I 0 → ... → I n − 1 → K n → 0, i.e. a resolution of Z X . ◮ Flabby ⇒ soft ⇒ c-soft ⇒ f -soft. Hence by the previous lemma, K n is also f -soft. ◮ Write K a = I a for a = 0 , 1 , ..., n − 1. ◮ In dealing with Hom D + (Sh( Y )) ( Rf ! F , G ), we would like to compute F via 0 → F → F ⊗ K 0 → ... → F ⊗ K n → 0 This is exact by the flatness. ◮ Here let me remind that tensor product of sheaves is the sheafification of tensor product on sections, and one checks the stalk of tensor product is tensor product of stalks.
Proof of Verdier duality, IV we would like to compute F via 0 → F → F ⊗ K 0 → ... → F ⊗ K n → 0 ◮ We claim that each F ⊗ K a is again f -soft. ◮ We have the resolution ... → P − 1 → P 0 → F → 0 by taking P 0 to be the direct sum of ( U → X ) ! Z U for each section s ∈ F ( U ) (so P 0 → F sends 1 to s ). And P − 1 is the analogous for ker( P 0 → F ), etc. ◮ As K a is flat, we again have ... → P − 1 ⊗ K a → P 0 ⊗ K a → F ⊗ K a → 0. ◮ Each P b ⊗ F can be checked to be f -soft. In particular this is the case for − n + 1 ≤ b ≤ 0. But then the lemma last page again says F ⊗ K a is f -soft. This proves the claim. ◮ Now for G ∈ Sh( Y ), we define f ! G to be the complex of sheaves given as follows: for any open j : U → X we have ( f ! G ) − a ( U ) = Hom Sh( Y ) ( f ! ( j ! j ∗ K a ) , G ) . ◮ Likewise, for any bounded below complex G • , let f ! G • be the complex associated to the double complex ( f ! G b ) − a .
Recommend
More recommend