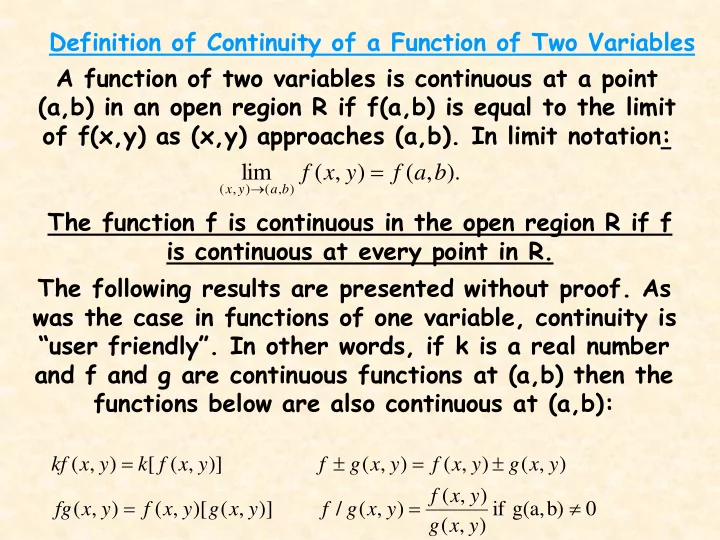
Definition of Continuity of a Function of Two Variables A function - PowerPoint PPT Presentation

Definition of Continuity of a Function of Two Variables A function of two variables is continuous at a point (a,b) in an open region R if f(a,b) is equal to the limit of f(x,y) as (x,y) approaches (a,b). In limit notation:  lim f ( x , y ) f ( a , b ).  ( x , y ) ( a , b ) The function f is continuous in the open region R if f is continuous at every point in R. The following results are presented without proof. As was the case in functions of one variable, continuity is “ user friendly ” . In other words, if k is a real number and f and g are continuous functions at (a,b) then the functions below are also continuous at (a,b):     kf ( x , y ) k [ f ( x , y )] f g ( x , y ) f ( x , y ) g ( x , y ) f ( x , y )    fg ( x , y ) f ( x , y )[ g ( x , y )] f / g ( x , y ) if g(a, b) 0 g ( x , y )

Example 1. Find the limit and discuss the continuity of the function. x lim   2 x y ( x , y ) ( 1 , 2 ) Solution x 1 1 1    lim    2 ( x , y ) ( 1 , 2 ) 2 x y 2 ( 1 ) 2 4 The function will be continuous when 2x+y > 0. Example 2. Find the limit and discuss the continuity x of the function. lim   2 x y ( x , y ) ( 1 , 2 ) Solution x 1 1   lim    2 x y 2 ( 1 ) 2 4 ( x , y ) ( 1 , 2 )  y  The function will be continuous when 2 x 0 . The function will not be defined when y = -2x.

Partial Derivatives of a Function of Several Variables The partial derivative, with respect to x of a function  z f ( , x y ) is:      z z f x ( x y , ) f x y ( , )    x z lim lim x        x x x x 0 x 0 z  f ( x , y ) The partial derivative of the function with respect to x is the ordinary derivative with respect to x calculated on the assumption that y is constant and vice versa.

Example For the following functions, find the partial derivatives:   z z ,   x y   2 sin  i z x y     2 2 ii z x y    y    1   iii z tan   x    y iv z x

Solution   2 sin  i z x y     z z 2 cos x y 2 sin x y   y x     2 2 ii z x y     z 2 x z 2 y   x 2  2 y  2 2 2 x y 2 x y

   y    1   iii z tan   x     2 / z 1 x z y x /    2  y  2 1 ( / ) y x x 1 ( / ) y x    y iv z x     z z  y 1 y x ln x y x   y x

Remark: The partial derivatives of a function of any number of variables are determined similarly as two variables. Example    2 2 3 u x y xtz Find the derivatives of with respect to x, y, z and t. Solution   u u    3 2 y 2 x tz   x y   u u   2 3 3 xtz xz   z t

Example      3 sin z y x y / xz yz 3 z show that If x y Solution        3 2 z y cos x y / 1/ y y cos x y / x          2 3 2 z 3 y sin x y / y cos x y / x y / y   2 cos  x z x y x y / x +       3 2 y z 3 y sin x y / x y cos x y / y     3 3 y sin x y / 3 z

Partial Derivatives of Higher Orders: z  f ( x , y ) The second order partial derivatives of if they exist, are written as:    2 2 z f x y ( , )     z f ( , x y ) f ( , x y )    xx xx x 2 2 x x x 2 ( ,    2 z f x y )     z f ( , x y ) f ( , x y )      xy xy x y x y y x 2 ( ,    2 z f x y )     z f ( , x y ) f ( , x y )      yx yx y x y x x y    2 2 z f x y ( , )     z f ( , x y ) f ( , x y )    yy yy y 2 2 y y y

Example   xy z e x . Find the four 2nd order partial derivatives of Solution   z z    xy xy xe ye 1   y x   2 2 z z   2 xy 2 xy x e y e  2  y 2 x  2 z  2   z xy xy xye e   xy xy xye e   x y   y x

Example  4 2  u  2 x y u z e . if Compute    2 z y x Solution  u   2 2 x y z e  x  2 u   2 2 x y z e  2 x  3 u   2 2 x y 2 yz e   2 y x  4 u   2 x y 4 yze    2 z y x

Theorem : z  f ( x , y ) If the function and its partial derivatives are defined and continuous at any point and in some neighborhood of it, then at this point   2 2 f f      y x x y This is true for a function of any number of variables and any number of successive partial derivatives.

The Chain Rule: Let z be a function of two independent variables u and v ,  z f u v ( , ) and let u and v be continuous functions of the independent variables x , y :     u ( x , y ), v ( x , y ). then:      z z u z v        x u x v x      z z u z v        y u y v y

x u x u u y y z u z x v z x v v v y y

Example  y z     2 3 Given . Find z u 2 v , u xy v , .  x x Solution        z z u z v y      2   2 uy 6 v        x u x v x 2 x 3 6 y   2 2 xy 4 x y  2 2 u y 6 v 2 x

Example       z 2 2 x y 2 z ln( u v ), u e , v x Given . Find .  x Solution      2 u 1 z z u z v      2 x y e 2 x        2 2 x u x v x u v u v 2   2  x y ( ue x )  2 u v e  2 2 u x y 1 2 x   2 2 u v u v