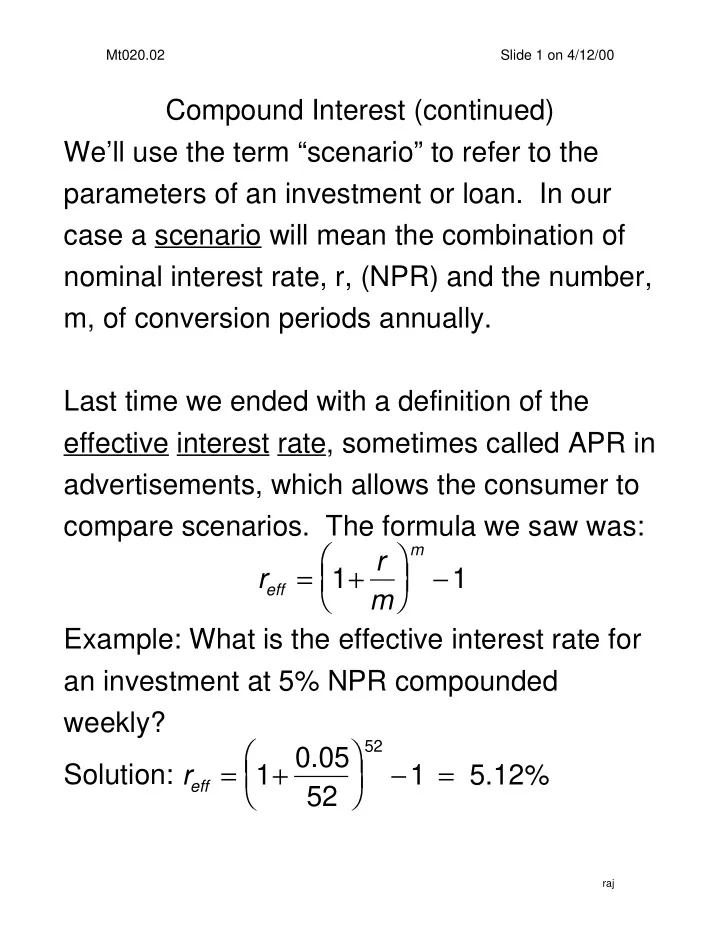

Mt020.02 Slide 1 on 4/12/00 Compound Interest (continued) We’ll use the term “scenario” to refer to the parameters of an investment or loan. In our case a scenario will mean the combination of nominal interest rate, r, (NPR) and the number, m, of conversion periods annually. Last time we ended with a definition of the effective interest rate, sometimes called APR in advertisements, which allows the consumer to compare scenarios. The formula we saw was: m r eff = 1 + r − 1 m Example: What is the effective interest rate for an investment at 5% NPR compounded weekly? 52 Solution: r eff = 1 + 0.05 − 1 = 5.12% 52 raj
Mt020.02 Slide 2 on 4/12/00 Two financial vocabulary words should be introduced here: present value (PV) and future value (FV) of an investment. Simply speaking these two ideas have already been encountered here as, principal, P, and accumulated amount, A, respectively. Analysts view the principal, as the present value and the value of that sum in the future, the accumulated value is the future value. Example: Terry came into some money with an inheritance and wonders how much she should put aside to “grow” into the $15,000 she wants for a car when she turns 18. She’s only 14 now. Her investment will grow at 8% annually with interest compounded monthly. Solution: Terry needs to know the present value of her $15,000 four years into the future under the specified scenario. raj
Mt020.02 Slide 3 on 4/12/00 Present value requires us to “pull back” the $15,000 to the present by using the “expansion factors” that we’ve already developed to “contract” the sum of money. The scenario says our conversion period expansion factor is 1 + 0.08 and we get the present value as: 12 − mt − (12)(4) = 15,000 1 + 0.08 PV = FV 1 + r 12 m = $10,903.81 showing us that Terry will have to save almost $11,000 of her inheritance to reach her goal. If our interest rate remains constant, we’ve observed that by compounding our interest more and more frequently during a year (allowing m to grow bigger and bigger), the scenario becomes increasingly attractive to us. raj
Mt020.02 Slide 4 on 4/12/00 What happens as m approaches infinity? t mt m A = P 1 + r 1 + r = P m m Before we let m get arbitrarily large we make a simple substitution, letting u = m/r. This makes our expression become: And as m gets bigger, so does u (because r is constant). So we get raj
Mt020.02 Slide 5 on 4/12/00 A = P e rt This is what is called continuous compounding of interest, the limiting case of having infinitely many conversion periods per year, compounding interest every second. Example: If Terry had compounded her investment of $10,903.81 continuously at 8% (instead of monthly) she’d have accumulated A = (10,903.81)e (0.08)(4) = (10,903.81)e (0.32) = $15,015.94, an additional $15.94. This continuously compounding formula can be used to “pull back” a future value to the present using PV = (FV) e (-rt) raj
Mt020.02 Slide 6 on 4/12/00 Example: A condominium complex was purchased by a group of investors for $1.4 million and sold 6 years later for $3.6 million. Find the annual rate of return (assuming continuous compounding) on their investment. Solution: (FV) = (PV)e (rt) becomes 3.6 = (1.4)e (6r) and we have to solve this for the nominal interest rate r. To do this we use natural logarithms to neutralize the exponential function: 1.4 = e 6 r ⇒ 3.6 ln 3.6 = 6 r 1.4 solving this for r, using properties of the natural logarithm we find: r = 1 [ ] = 15.74% 6 ln(3.6) − ln(1.4) raj
Recommend
More recommend