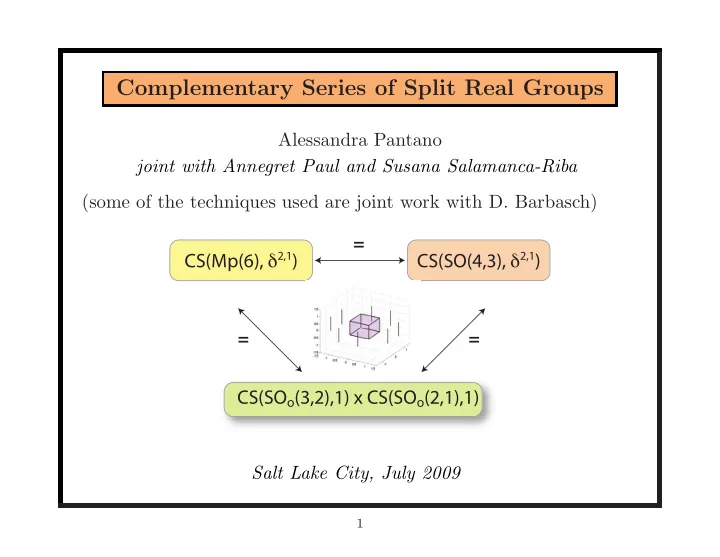
Complementary Series of Split Real Groups Alessandra Pantano joint - PowerPoint PPT Presentation

Complementary Series of Split Real Groups Alessandra Pantano joint with Annegret Paul and Susana Salamanca-Riba (some of the techniques used are joint work with D. Barbasch) = CS(Mp(6), δ 2,1 ) CS(SO(4,3), δ 2,1 ) = = CS(SO o (3,2),1) x CS(SO o (2,1),1) Salt Lake City, July 2009 1

Introduction Discuss the unitarity of minimal principal series Aim of Mp (2 n ) and SO ( n + 1 , n ). Genuine ? Complementary series complementary series of SO(n+1,n) of Mp(2n) ? ? Union of spherical complementary series of certain orthogonal groups 2

PART 1 Genuine Complementary Series of Mp (2 n ) Genuine ? Complementary series complementary series of SO(n+1,n) of Mp(2n) ? ? FIRST Union of spherical complementary series of certain orthogonal groups 3

NOTATION • G := Mp (2 n ) the connected double cover of Sp (2 n, R ) K := � • U ( n ) the maximal compact subgroup of G = { [ g, z ] ∈ U ( n ) × U (1): det( g ) = z 2 } • g 0 = k 0 ⊕ p 0 • a 0 := maximal abelian subspace of p 0 • M := Z K ( a 0 ) • ∆( g 0 , a 0 ) = {± ǫ k ± ǫ l } k,l =1 ...n ∪ {± 2 ǫ k } k =1 ...n type C n • W ≃ S n ⋉ ( Z / 2 Z ) n all permutations and sign changes 4

The group M and its genuine representations M = Z K ( a 0 ) subgroup of K generated by the elements � � m k = diag(1 , . . . , 1 , − 1 k , 1 , . . . , 1) , i , k = 1 . . . n (of order 4) Genuine M -types Irreducible repr.s δ of M s.t. δ ([ I, − 1]) � = +1 . � m 2 k = [ I, − 1] → each generator m k acts by ± i Subsets S ⊂ { 1 . . . n } S keeps track of which generators act by − i   − i if k ∈ S Mp (6) m 1 m 2 m 3 δ S ( m k ) =  − i − i + i otherwise δ { 2 , 3 } + i 5

An action of the Weyl group on genuine M -types W acts on � M ← ( s α · δ )( m ) := δ ( σ − 1 α mσ α ) ∀ m ∈ M, ∀ α ∈ ∆ W δ := { w ∈ W : w · δ ≃ δ } . The stabilizer of δ in W is For all S ⊂ { 1 , . . . , n } , set q = | S | , p = | S c | . • W δ S ≃ W ( C p ) × W ( C q ) ← s 2 ǫ k & s ǫ k ± ǫ l , k, l in S or S C • W · δ S = { δ T : | T | = q, | T c | = p } W -orbits of genuine M -types � pairs ( p, q ): p, q ∈ N , p + q = n   if k ≤ p + i Pick representatives δ p,q := δ { p +1 ,...,n } . δ p,q ( m k ) =  − i if k > p . 6

The group K and its genuine representations K = � Maximal compact subgroup of G : U ( n ) parameterized by highest weight ( a 1 , . . . , a n ) Genuine K -types with a 1 ≥ a 2 ≥ · · · ≥ a n and a j ∈ Z + 1 2 , ∀ j fine K -types highest weight restriction to M Λ p ( C n ) ⊗ det − 1 / 2 ( 1 2 , . . . , 1 , − 1 2 , . . . , − 1 W · δ p,q ) 2 2 � �� � � �� � p q • If we restrict a fine K -type to M , we get one full W -orbit in � M • Each genuine M -type δ is contained in a unique fine K -type µ δ . 7

Genuine Complementary Series of Mp (2 n ) • MA := Levi factor of a minimal parabolic • δ := genuine irreducible representation of M • ν := real character of A • P = MAN := a minimal parabolic making ν weakly dominant. I P ( δ, ν ) := Ind G P ( δ ⊗ ν ⊗ 1) Minimal Principal Series J ( δ, ν ) := composition factor of I P ( δ, ν ) ⊇ µ δ Langlands Quotient δ - Complementary Series CS ( G, δ ) := { ν ∈ a ∗ R | J ( δ, ν ) is unitary } Problem : Find CS ( Mp (2 n ) , δ p,q ) 8

THEOREM 1 Theorem 1: For all ν ∈ a ∗ R , write ν := ( ν p | ν q ). The map: CS ( Mp (2 n ) , δ p,q ) → CS ( SO ( p + 1 , p ) 0 , 1) × CS ( SO ( q + 1 , q ) 0 , 1) ν �→ ( ν p , ν q ) is a well defined injection. (1 denotes the trivial M -type ) Spherical complementary series of real split orthogonal groups are known (Barbasch). Hence this theorem provides explicit necessary conditions for the unitarity of genuine principal series of Mp (2 n ) . 9

Example: CS ( Mp (6) , δ 2 , 1 ) → CS ( SO (3 , 2) 0 , 1) × CS ( SO (2 , 1) 0 , 1) ν 2 ν 1 1/2 3/2 CS ( SO (3 , 2) 0 , 1) CS ( SO (2 , 1) 0 , 1) ♣ . . . . . . ♣ ♣ ♣ r ♣ ♣ ♣ r ♣ ♣ - 3 - 1 1 3 -2 -1 0 1 2 2 2 2 2 ⇒ CS ( Mp (6) , δ 2 , 1 ) embeds into: 10

A reformulation of THEOREM 1 For all p, q ∈ N s.t. p + q = n , set: G δ p,q ≡ SO ( p + 1 , p ) 0 × SO ( q + 1 , q ) 0 and note that W ( G δ p,q ) = W δ p,q . connected real split group whose root system is G δ p,q := dual to the system of good roots for δ p,q . The δ p,q -complementary series of Mp (2 n ) Theorem 1: embeds into the spherical complementary series of G δ p,q . Proof: based on Barbasch’s idea to use calculations on petite K-types to compare unitary parameters for different groups. 11

Comparing unitary parameters for Mp (2 n ) and G δ p,q J ( δ p,q , ν ) unitary for Mp (2 n ) J (1 , ν ) unitary for G δ p,q � � T ( µ, δ p,q , ν ) A ( ψ, 1 , ν ) < − pos. semidefinite pos. semidefinite ∀ µ ∈ � | K ∀ ψ ∈ � W δ p,q ? | � ? | A ( ψ, 1 , ν ) | pos. semidefinite − > W δ p,q relevant ∀ ψ ∈ � 12

A matching of operators Key Proposition : ∀ relevant W δ p,q -type ψ , ∃ a “petite” K -type µ s.t. T ( µ, δ p,q , ν ) = A ( ψ, 1 , ν ) � �� � � �� � operator for G δp,q operator for Mp (2 n ) Sketch of the proof: • T ( µ, δ p,q , ν ) is defined on Hom M ( µ, δ p,q ) • This space carries a representation ψ µ of W δ p,q ← = W ( G δ p,q ) • Attached to ψ µ , ∃ a spherical operator A ( ψ µ , 1 , ν ) for G δ p,q • If µ is petite, T ( µ, δ p,q , ν ) = A ( ψ µ , 1 , ν ) W δ p,q relevant, ∃ µ ∈ � • For all ψ ∈ � K petite such that ψ = ψ µ . � 13

A matching of relevant W δ p,q -types with petite K -types      1 2 , . . . , 1 , − 1 2 , . . . , − 1 , − 3 2 , . . . , − 3 (( p − s ) × ( s )) ⊗ triv  2 2 2 � �� � � �� � � �� � q s p − s      3 2 , . . . , 3 , 1 2 , . . . , 1 , − 1 2 , . . . , − 1 ( p − s, s ) ⊗ triv  2 2 2 � �� � � �� � � �� � s p − 2 s q + s      3 2 , . . . , 3 , 1 2 , . . . , 1 , − 1 2 , . . . , − 1 triv ⊗ (( q − r ) × ( r ))  2 2 2 � �� � � �� � � �� � r p q − r      1 2 , . . . , 1 , − 1 2 , . . . , − 1 , − 3 2 , . . . , − 3 triv ⊗ ( q − r, r )  2 2 2 � �� � � �� � � �� � q − 2 r r p + r 14

J (1 , ν ) unitary for G δ p,q J ( δ p,q , ν ) unitary for Mp (2 n ) � � T ( µ, δ p,q , ν ) A ( ψ, 1 , ν ) pos. semidefinite pos. semidefinite ∀ ψ ∈ � ∀ µ ∈ � W δ p,q K ⇓ � T ( µ, δ p,q , ν ) A ( ψ, 1 , ν ) pos. semidefinite pos. semidefinite == > W δ p,q relevant ∀ ψ ∈ � ∀ µ ∈ � K petite ↑ | W δ p,q relevant, ∃ µ ∈ � ∀ ψ ∈ � K petite s.t. A ( ψ, 1 , ν )= T ( µ, δ p,q , ν ) 15

Non-unitarity certificates Let G δ p,q = SO ( p + 1 , p ) 0 × SO ( q + q, q ) 0 . For all ν = ( ν p | ν q ): J ( δ p,q , ν ) unitary for Mp (2 n ) == > J (1 , ν ) unitary for G δ p,q . The spherical unitary dual of split orthogonal groups is known. So we get non-unitarity certificates for genuine L.Q.s of Mp (2 n ) . If Theorem 1’: • the spherical L.Q. J (1 , ν p ) of SO ( p + 1 , p ) 0 is not unitary , or • the spherical L.Q. J (1 , ν q ) of SO ( q + 1 , q ) 0 is not unitary then the genuine L.Q. J ( δ p,q , ( ν p | ν q )) of Mp (2 n ) is also not unitary . 16

An example of non-unitarity certificate Let ν = ( ν 1 , . . . , ν n ). We may assume: ν 1 ≥ · · · ≥ ν p ≥ 0 and ν p +1 ≥ · · · ≥ ν n ≥ 0 , by W δ p,q -invariance. (Recall W δ p,q = W ( C p ) × W ( C q ).) If any of the following conditions holds: • ν p > 1 / 2 • ν n > 1 / 2 • ν a − ν a +1 > 1 , for some a with 1 ≤ a ≤ p − 1, or • ν a − ν a +1 > 1 , for some a with p + 1 ≤ a ≤ n − 1 then the genuine Langlands quotient J ( δ p,q , ν ) of Mp (2 n ) is not unitary. 17

An application This non-unitarity certificate is a key ingredient in the classification of the ω - regular unitary dual of Mp (2 n ). Definition: A representation of Mp (2 n ) is called ω - regular if its infinitesimal character is at least as regular as the one of the oscillator representation. The only ω - regular complementary series repr.s of Corollary: Mp (2 n ) are the two even oscillator representations: � � �� � � �� n − 1 2 , . . . , 3 2 , 1 n − 1 2 , . . . , 3 2 , 1 J δ 0 ,n , and J δ n, 0 , . 2 2 18

PART 2 Complementary Series of SO ( n + 1 , n ) Genuine ? Complementary series complementary series of SO(n+1,n) of Mp(2n) ⊆ ? Theor. 1 NEXT Union of spherical complementary series of certain orthogonal groups 19

NOTATION • G := SO ( n + 1 , n ) • K := S ( O ( n + 1) × O ( n )) maximal compact dual to previous • ∆( g 0 , a 0 ) = {± ǫ k ± ǫ l } ∪ {± ǫ k } type B n ← case same Weyl group • W ≃ S n ⋉ ( Z / 2 Z ) n ← as before • M := Z K ( a 0 ) = { diag(1 , t n , . . . , t 1 , t 1 , . . . , t n ): t j = ± 1 , ∀ j } 20