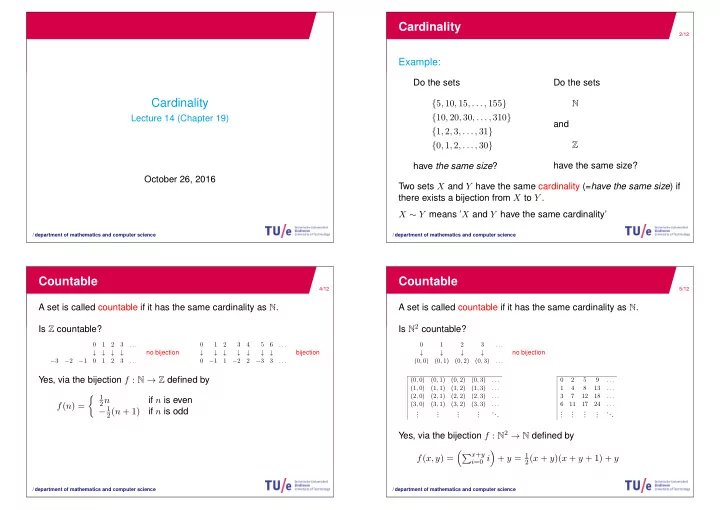
Cardinality 2/12 Example: Do the sets Do the sets Cardinality { - PowerPoint PPT Presentation

Cardinality 2/12 Example: Do the sets Do the sets Cardinality { 5 , 10 , 15 , . . . , 155 } N { 10 , 20 , 30 , . . . , 310 } Lecture 14 (Chapter 19) and { 1 , 2 , 3 , . . . , 31 } { 0 , 1 , 2 , . . . , 30 } Z have the same size? have the same size ? October 26, 2016 Two sets X and Y have the same cardinality (= have the same size ) if there exists a bijection from X to Y . X ∼ Y means ’ X and Y have the same cardinality’ / department of mathematics and computer science / department of mathematics and computer science Countable Countable 4/12 5/12 A set is called countable if it has the same cardinality as N . A set is called countable if it has the same cardinality as N . Is N 2 countable? Is Z countable? 0 1 2 3 . . . 0 1 2 3 4 5 6 . . . 0 1 2 3 . . . ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ ↓ no bijection bijection no bijection − 3 − 2 − 1 0 1 2 3 . . . 0 − 1 1 − 2 2 − 3 3 . . . (0 , 0) (0 , 1) (0 , 2) (0 , 3) . . . Yes, via the bijection f : N → Z defined by (0 , 0) (0 , 1) (0 , 2) (0 , 3) 0 2 5 9 . . . . . . (1 , 0) (1 , 1) (1 , 2) (1 , 3) . . . 1 4 8 13 . . . � 1 (2 , 0) (2 , 1) (2 , 2) (2 , 3) . . . 3 7 12 18 . . . 2 n if n is even f ( n ) = (3 , 0) (3 , 1) (3 , 2) (3 , 3) . . . 6 11 17 24 . . . − 1 2 ( n + 1) if n is odd . . . . . . . . ... ... . . . . . . . . . . . . . . . . Yes, via the bijection f : N 2 → N defined by �� x + y � + y = 1 f ( x, y ) = i =0 i 2 ( x + y )( x + y + 1) + y / department of mathematics and computer science / department of mathematics and computer science

Countable Infinite 0 - 1 -sequences 6/12 9/12 A set is called countable if it has the same cardinality as N . Theorem: Is Q + countable? The set of infinite 0 - 1 -sequences is not countable. Proof: 1 1 1 1 . . . 1 2 3 4 Reductio ad absurdum: we suppose that the set of infinite 2 2 2 2 X X . . . 0 - 1 -sequences is countable, and derive a contradiction. 1 2 3 4 3 3 3 3 X . . . If the set of infinite 0 - 1 -sequences is countable, then there exists an 1 2 3 4 4 4 4 4 enumeration, i.e., a bijection from N to that set: X X . . . 1 2 3 4 . . . . 0 1 2 3 . . . ← N ... . . . . . . . . ↓ ↓ ↓ ↓ ← all 0 - 1 -sequences without repetition ϕ 0 ϕ 1 ϕ 2 ϕ 3 . . . The bijection from N to Q + is similar to the bijection from N to N 2 , except that n d has to be skipped if n and d are not relatively prime. [Proof continues on next slide.] / department of mathematics and computer science / department of mathematics and computer science Infinite 0 - 1 -sequence (continued proof) Uncountable 10/12 11/12 List those sequences ϕ 0 , ϕ 1 , ϕ 2 , ϕ 3 , . . . : An infinite set that is not countable, is called uncountable.  = · · · ϕ 0 a 00 a 01 a 02 a 03   = · · · Examples: { 0 , 1 } N , N N , � 0 , 1 � , R , P ( N ) , . . . ϕ 1 a 10 a 11 a 12 a 13     ϕ 2 = a 20 a 21 a 22 a 23 · · · every a ij is either 0 or 1 ϕ 3 = a 30 a 31 a 32 a 33 · · · Degrees of infinity:    . .  . .  . .  N , Z , Q , N 2 , Z 2 , Q × Z , . . . ℵ 0 : countable Now consider the diagonal sequence R , P ( N ) , { 0 , 1 } N , N N , . . . ℵ 1 : uncountable ψ = a 00 a 11 a 22 a 33 · · · and in it replace every 0 by a 1 and vice versa: ℵ 2 : P ( R ) , P ( P ( N )) , . . . uncountable χ = b 0 b 1 b 2 b 3 · · · met b i � = a ii . Then no ϕ i can be equal to χ , so χ is not in the enumeration. NB: N �∼ R �∼ P ( R ) . But χ is an infinite 0 - 1 -sequence; so it must be in the enumeration. Contradiction! / department of mathematics and computer science / department of mathematics and computer science

Exercises 12/12 Exercise 1 Prove that R + has the same cardinality as R . Exercise 2 Prove that every equivalence class associated with the equivalence relation ≡ 6 is countable. Exercise 3 Prove that the set N × { 0 , 1 } is countable. Exercise 4 Prove that if V and W are countable, then also V × W is countable. Exercise 5 Prove that the set N N of all functions from N to N is uncountable. / department of mathematics and computer science