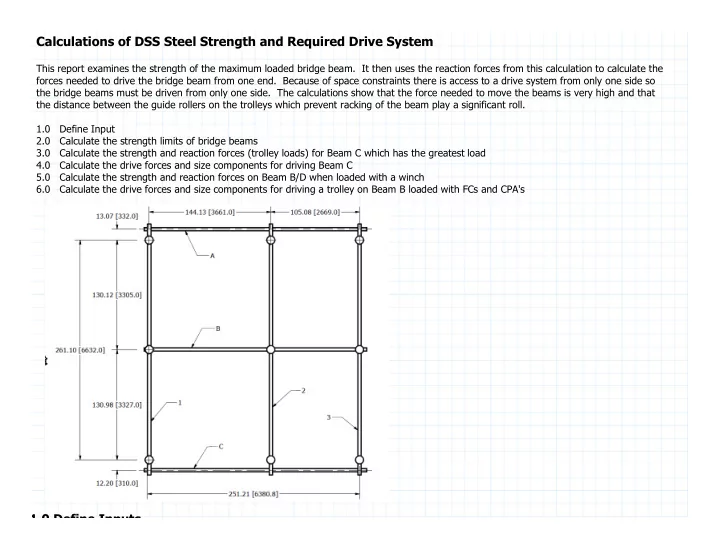
Calculations of DSS Steel Strength and Required Drive System This - PowerPoint PPT Presentation

Calculations of DSS Steel Strength and Required Drive System This report examines the strength of the maximum loaded bridge beam. It then uses the reaction forces from this calculation to calculate the forces needed to drive the bridge beam from one end. Because of space constraints there is access to a drive system from only one side so the bridge beams must be driven from only one side. The calculations show that the force needed to move the beams is very high and that the distance between the guide rollers on the trolleys which prevent racking of the beam play a significant roll. 1.0 Define Input 2.0 Calculate the strength limits of bridge beams 3.0 Calculate the strength and reaction forces (trolley loads) for Beam C which has the greatest load 4.0 Calculate the drive forces and size components for driving Beam C 5.0 Calculate the strength and reaction forces on Beam B/D when loaded with a winch 6.0 Calculate the drive forces and size components for driving a trolley on Beam B loaded with FCs and CPA's 1 0 Define Inputs

1.0 Define Inputs Beams are stainless steel S8x18.4 in 2 ≔ 5.4 in ⋅ A S8 lbf lbf lbf lbf ≔ A S8 .283 ―― ⋅ ⋅ = 1.5 ―― q beam in 3 in in in in 4 ≔ 57.5 in ⋅ Ix in 3 ≔ 14.4 in ⋅ Sx ≔ 3.26 in ⋅ r in in 3 ≔ 16.5 in Z ≔ 6 in T in 7 ≔ ⋅ ― tf in in 16 1 ≔ ⋅ ― tw in in 4 Material - A36 ≔ 36 ksi fy ksi ≔ 50 ksi fu ksi ≔ 29000 ksi E ksi Geometry ≔ 332.0 mm a mm ≔ 3305 mm b mm ≔ 3327 mm c mm ≔ 310 mm d mm ≔ 3661 mm e mm ≔ 2669 mm f mm Length of beam ≔ + = 20.8 ft L bridge e f ft Spacing between CPA supports ≔ 1.1 m spacing cpa 1 t 6 l ti f t ll /l d i t l f

+ f ≔ ―― − 2.5 spacing cpa ⋅ = 16.3 in x1 x1 to x6 are locations of trolley/load points along 2 the length of beam from one end + e f ≔ − 1.5 spacing cpa ⋅ = 59.6 in ―― x2 in 2 + e f ≔ ―― − 0.5 spacing cpa ⋅ = 103 in x3 in 2 + e f ≔ ―― + 0.5 spacing cpa ⋅ = 146.3 in x4 in 2 + e f ≔ ―― + 1.5 spacing cpa ⋅ = 189.6 in x5 in 2 + e f ≔ + 2.5 spacing cpa ⋅ = 232.9 in ―― x6 in 2 Weight of CPA ≔ 160 lbf ⋅ W CPA lbf Weight of FC ≔ 440 lbf ⋅ W FC lbf Weight of bridge beam ≔ ⋅ = 380.8 lbf W beam q beam L bridge lbf 2.0 Calculate Strength Limits of S8x14.5 beam ≔ 1.0 γ M0 ≔ 1.0 γ M1 ≔ 1.25 γ M2 ‾‾‾‾‾‾ 235 ≔ = 0.973 ―― ε fy ――

MPa MPa T Class 1 cross section ― = 24 73 ε ⋅ = 71 33 ε ⋅ = 32.1 tw ⋅ Z fy ≔ = 49500 ⋅ ―― M c lbf lbf ft γ M0 3.0 Calculate Reaction Forces and Stresses in CPA Support beam (C Beam) Worst case is during installation and CPA carried total load of FC ⎛ ⎞ W FC Load from CPA strap onto bridge beam ≔ + 4 ⋅ ―― = 1040 lbf ⎜ ⎟ F CPA W CPA lbf 2 ⎝ ⎠ ≔ 1000 lbf ⋅ ≔ 1000 lbf ≔ 1000 lbf ⋅ ≔ .1 in Ra lbf Rb lbf Rc lbf y7 in ≔ .1 in ≔ .1 in ≔ .1 in ≔ .1 in ≔ .1 in ≔ .1 in y1 in y2 in y3 in y4 in y5 in y6 in 2 L bridge F CPA ( x6 ) ⋅ + + + + + + ⋅ − ⋅ − ⋅ ＝ 0.0 ( ) q beam ――― x1 x2 x3 x4 x5 e Rb L bridge Rc 2 + + ＝ 6 F CPA ⋅ + ⋅ Ra Rb Rc q beam L bridge 3 ⎞ F CPA ⎛ ⎠ e 3 ⎞ ⎛ −⎛ ⎛ x1 ⎞ ⎠ ⎛ x1 ⎞ ⎠⎞ ⎛ x1 ⎞ L bridge ( x1 ) 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ − ⋅ ( − ＝ ――――― ) L bridge ⎠ x1 e L bridge e y1 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅ 3 ⎞ F CPA ⎛ ⎠ e 3 ⎞ ⎛ −⎛ ⎛ x2 ⎞ ⎠ ⎛ x2 ⎞ ⎠⎞ ⎛ x2 ⎞ L bridge ( x2 ) ――――― 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ − ⋅ ( − ) ＝ L bridge ⎠ x2 e L bridge e y2 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅ 3 ⎞ F CPA ⎛ ⎠ e 3 ⎞ ⎛ −⎛ ⎛ x3 ⎞ ⎠ ⎛ x3 ⎞ ⎠⎞ ⎛ x3 ⎞ L bridge ( x3 ) ――――― 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ − ⋅ ( − ＝ ) L bridge ⎠ x3 e L bridge e y3 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅ F CPA ⎠ e 3 ⎞ ⎛ ⎛ ⎠⎞ −⎛ ⎛ x4 ⎞ ⎠ ⎛ x4 ⎞ ⎠⎞ ⎛ x4 ⎞ 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ ＝ ――――― L bridge ⎠ x4 e L bridge y4 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅

F CPA ⎠ e 3 ⎞ ⎛ ⎛ ⎠⎞ −⎛ ⎛ x5 ⎞ ⎠ ⎛ x5 ⎞ ⎠⎞ ⎛ x5 ⎞ ――――― 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ ＝ L bridge ⎠ x5 e L bridge y5 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅ F CPA ⎠ e 3 ⎞ ⎛ ⎛ ⎠⎞ −⎛ ⎛ x6 ⎞ ⎠ ⎛ x6 ⎞ ⎠⎞ ⎛ x6 ⎞ 2 L bridge − ⋅ − ⋅ ⋅ + − ⋅ ＝ ――――― L bridge ⎠ x6 e L bridge y6 ⎝ ⎝ ⎝ ⎝ ⎝ ⎝ ⎠ 6 E Ix L bridge ⋅ ⋅ ⋅ − q beam e ⋅ e 3 ⎞ ⎛ 3 2 L bridge e 2 ⋅ − ⋅ ⋅ + ＝ ――― L bridge y7 ⎝ ⎠ 24 E Ix ⋅ ⋅ − Rb e 2 f 2 ⋅ ⋅ + + + + + + ＝ ――――― y1 y2 y3 y4 y5 y6 y7 3 E Ix L bridge ⋅ ⋅ ⋅ ⎡ ⎤ 1582.9 lbf lbf ⎢ ⎥ 4097.2 lbf lbf ⎢ ⎥ 940.8 lbf lbf ⎢ ⎥ 0 ft ft ⎢ ⎥ ⎢ ⎥ 0 ft ft Find ( Ra Rb Rc y1 y2 y3 y4 y5 y6 y7 ) So Sol ≔ Find , , , , , , , , , = ( ) ⎢ ⎥ 0 ft ft ⎢ ⎥ 0 ft ft ⎢ ⎥ 0 ft ft ⎢ ⎥ 0 ft ⎢ ⎥ ft ⎢ ⎥ 0 ft ⎣ ⎦ ft 6 F CPA ⋅ + ⋅ = 6620.8 lbf q beam L bridge lbf Sol ( (0) Sol ( (3) Ra,Rb,Rc are reaction forces from Beam B to transverse beam -- ≔ So = 1582.9 lbf ≔ So = −0.0364 in ) ) Ra trolley lbf y1 in these are the loads on each trolley supporting Beam B. Y1 to Y6 are Sol ( (4) ≔ So = −0.1242 in ) y2 in the deformations caused by each load at trolley Rb Sol ( (1) Sol ( (5) ≔ So = 4097.2 lbf ≔ So = −0.1826 in ) ) Rb trolley lbf y3 in Sol ( (6) ≔ So = −0.1902 in ) y4 in Sol ( (2) Sol ( (7) ≔ So = 940.8 lbf ≔ So = −0.1355 in Rc trolley ) lbf y5 ) in Sol ( (8) ≔ So = −0.0403 in ) y6 in Sol ( (9) + + = 6620.8 lbf ≔ So = −0.0447 in ) Ra trolley Rb trolley Rc trolley lbf y7 in + + + + + + = −0.7539 in y1 y2 y3 y4 y5 y6 y7 in

− Rb trolley e 2 f 2 ⋅ ⋅ ―――――― = −0.7539 in in 3 E Ix L bridge ⋅ ⋅ ⋅ Calculate moments along beam ‖ M ( ( x ) ≔ if ≤ ) x x1 ‖ ‖ q beam x 2 ‖ ⋅ ‖ ⋅ − ――― ‖ Ra trolley x ‖ 2 ‖ ‖ ‖ if < ≤ x1 x x2 ‖ ‖ q beam x 2 ⋅ ‖ ‖ F CPA ( x1 ) ⋅ − ⋅ ( − − ) ――― Ra trolley x x ‖ ‖ 2 ‖ ‖ ‖ if < ≤ x2 x x3 ‖ ‖ q beam x 2 ⋅ ‖ ‖ F CPA ( ( x1 ) ( x2 ) )) ⋅ − ⋅ ( − + ( − − ――― ( ) ) Ra trolley x x x ‖ ‖ 2 ‖ ‖ if < ≤ ‖ x3 x e ‖ ‖ q beam x 2 ⋅ ‖ ‖ F CPA ( ( x1 ) ( x2 ) ( x3 ) )) ⋅ − ⋅ ( − + ( − + ( − − ( ) ) ) ――― Ra trolley x x x x ‖ ‖ 2 ‖ ‖ if < ≤ e x x4 ‖ ‖ ‖ q beam x 2 ⋅ ‖ ‖ F CPA ( ( x1 ) ( x2 ) ( x3 ) )) Rb trolley ( e ) ⋅ − ⋅ ( − + ( − + ( − + ⋅ ( − − ――― ( ) ) ) ) Ra trolley x x x x x ‖ ‖ 2 ‖ ‖ if < ≤ x4 x x5 ‖ ‖ ‖ q beam x 2 ⋅ ‖ F CPA ( ( x1 ) ( x2 ) ( x3 ) ( x4 ) )) Rb trolley ( e ) ‖ ⋅ − ⋅ ( − + ( − + ( − + ( − + ⋅ ( − − ――― Ra trolley x ( x ) x ) x ) x ) x ) ‖ 2 ‖ ‖ ‖ if < ≤ x5 x x6 ‖ ‖ q beam x 2 ‖ ⋅ ‖ F CPA ( ( x1 ) ( x2 ) ( x3 ) ( x4 ) ( x5 ) )) Rb trolley ( e ) ⋅ − ⋅ ( − + ( − + ( − + ( − + ( − + ⋅ ( − − ( ) ) ) ) ) ) ――― ‖ Ra trolley x x x x x x x ‖ 2 ‖ ‖ ‖ if < ≤ x6 x L bridge ‖ ‖ q beam x 2 ‖ ⋅ ‖ F CPA ( ( x1 ) ( x2 ) ( x3 ) ( x4 ) ( x5 ) ( x6 ) )) Rb trolley ( e ) ⋅ − ⋅ ( ( − ) + ( − ) + ( − ) + ( − ) + ( − ) + ( − ) + ⋅ ( − ) − ――― Ra trolley x x x x x x x x ‖ ‖ 2 ‖ ‖ ‖