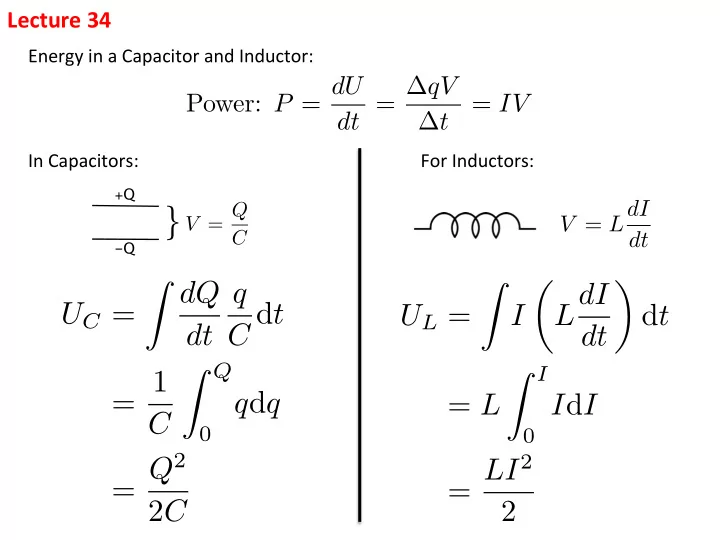
c dt q z dq q z ldi u c c d t u l i d t dt dt z q z i 1 q
play

} C dt -Q Z dQ q Z LdI U C = C d t U L = I d t dt dt Z Q - PowerPoint PPT Presentation

Oct 12, 2022 •253 likes •329 views

Lecture 34 Energy in a Capacitor and Inductor: dt = qV Power: P = dU = IV t In Capacitors: For Inductors: + Q V = LdI V = Q } C dt -Q Z dQ q Z LdI U C = C d t U L = I d

  1. Lecture ¡34 ¡ Energy ¡in ¡a ¡Capacitor ¡and ¡Inductor: ¡ dt = ∆ qV Power: P = dU = IV ∆ t In ¡Capacitors: ¡ For ¡Inductors: ¡ + Q V = LdI V = Q } C dt -Q Z dQ q ✓ ◆ Z LdI U C = C d t U L = I d t dt dt Z Q Z I = 1 q d q = L I d I C 0 0 = Q 2 = LI 2 2 C 2

  2. Mechanical ¡Analogy: ¡Mass ¡Spring ¡System ¡ F = ma = md 2 x dt 2 KE = 1 PE = 1 2 mv 2 2 kx 2 1 1 2 LI 2 2 C q 2 Corresponds ¡to: ¡ LC ¡circuits ¡oscillate ¡much ¡in ¡the ¡same ¡way ¡a ¡mass ¡spring ¡system ¡oscillates ¡

  3. LC ¡Circuit ¡ C L Basic ¡Equa?ons: ¡ LdI dt + q V L + V C = 0 Loop ¡Equa?on: ¡ C = 0 Power ¡Equa?on: ¡ P = I × loop eqn. = IV L + IV C = 0 ✓ ◆ LdI + dq q Conserva?on ¡of ¡ 0 = I Energy: ¡ dt dt C U L + U C = const. U C,max = U C + U L = U L,max

  4. Solving ¡the ¡Loop ¡Equa?on ¡– ¡Analogy ¡to ¡Mass-­‑Spring ¡System ¡ md 2 x dt 2 = − kx Solu?on: ¡ x = A cos ω t dx dt = − A ω sin ω t d 2 x dt 2 = − A ω 2 cos ω t = − ω 2 x Comment: ¡ x = x max cos( ω t + δ ) General ¡Solu?on: ¡ (δ ¡is ¡the ¡ini?al ¡phase ¡angle ¡of ¡the ¡oscilla?on) ¡ OJen ¡set ¡δ ¡= ¡0, ¡where ¡at ¡t ¡= ¡0, ¡the ¡ini?al ¡ ¡ condi?on ¡is ¡chosen ¡to ¡be ¡x ¡= ¡x max ¡

  5. LC ¡Circuit ¡Analogy ¡ q = q max cos ω t I = dq dt = − q max ω sin ω t + - = − I max sin ω t T /4 T /2 3 T /4 T 0 0 0 0 0 Q t - + I t 0 q charge on left plate. at t = 0, q = q max cos ( ω 0) = q max ✓ ω T ◆ ✓ 2 π ◆ I current in coil. At t = T 4 , I = − I max sin = − I max sin = I max 4 4

  6. Check: ¡ U L + U C = U 0 LHS = 1 2 L ( I max sin ω t ) 2 + 1 2 C ( q max cos ω t ) 2 u 0 = 1 2 LI 2 max = 1 2 L ω 2 q 2 max = 1 2 L 1 LC q 2 max u 0 = 1 2 C q 2 max LHS = u 0 sin 2 ω t + u 0 cos 2 ω t = u 0 sin 2 ω t + cos 2 ω t = 1 where ¡

  7. RLC ¡Circuit ¡– ¡Loop ¡Equa?on ¡ − LdI dt − q C − IR = 0 Power Eqn. = I × ( loop eqn ) 2 I 2 + q 2  L � − d − I 2 R = 0 dt 2 C + LI dI dt + q dq dt + I 2 R = 0 C Graph ¡of ¡Q ¡and ¡I ¡of ¡Dampened ¡Oscilla?on: ¡ P L + P C + P R = 0 Each ¡term ¡can ¡be ¡posi?ve ¡ Always ¡ or ¡nega?ve. ¡ posi?ve ¡ ¡ P L ¡+ ¡P C ¡stays ¡nega?ve ¡

Recommend

More recommend