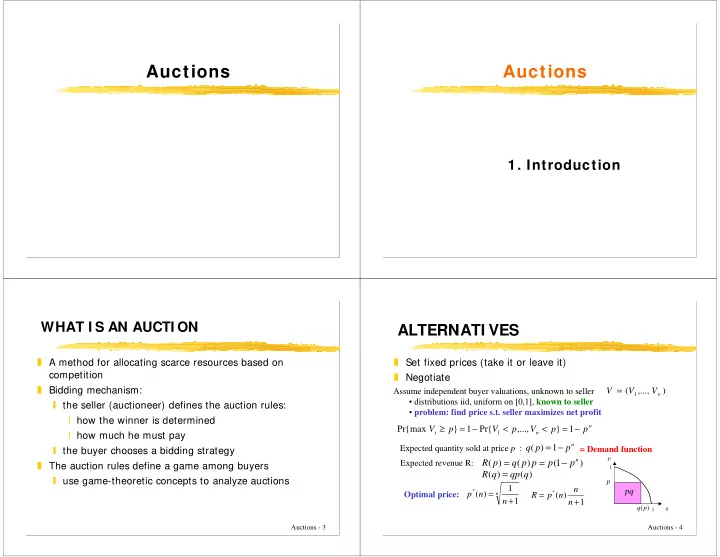

Auctions Auctions 1. Introduction WHAT I S AN AUCTI ON ALTERNATI VES ❚ A method for allocating scarce resources based on ❚ Set fixed prices (take it or leave it) competition ❚ Negotiate V = ❚ Bidding mechanism: ( V ,..., V ) Assume independent buyer valuations, unknown to seller 1 n • distributions iid, uniform on [0,1], known to seller ❙ the seller (auctioneer) defines the auction rules: • problem: find price s.t. seller maximizes net profit ❘ how the winner is determined ≥ = − < < = − n Pr{max V p } 1 Pr{ V p ,..., V p } 1 p ❘ how much he must pay i 1 n = 1 − n ( ) Expected quantity sold at price p : q p p ❙ the buyer chooses a bidding strategy = Demand function = = − p n Expected revenue R: ( ) ( ) ( 1 ) R p q p p p p ❚ The auction rules define a game among buyers 1 = R ( q ) qp ( q ) ❙ use game-theoretic concepts to analyze auctions p 1 n = pq * = ( ) * p n Optimal price: n R p ( n ) + + 1 n 1 n q ( p ) q 1 Auctions - 3 Auctions - 4
Auctions and resource allocation WHY AUCTI ONS ❚ An auction is a market mechanism which ❚ Useful in the context of partial information ❙ when selling a commodity of undetermined value ❙ allocates resources (goods) to customers ❘ value depends on buyer, or actual value to be found ex-ante ❘ generates value for the consumers ❙ when no information about value distribution of buyers ❙ generates revenue for the seller ❚ Simplicity, speed of sale ❘ generates revenue for the producer ❚ Reveal information about buyer’s valuation ❚ Is used where where traditional market ❚ Price determined by the market response mechanisms can not be used ❙ prevent dishonest dealing between seller-buyer value V i Seller buyers revenue Auctions - 5 Auctions - 6 TYPES OF AUCTI ONS Auctions ❚ Single unit ❙ English ❙ Dutch ❙ First price sealed bid ❙ Vickrey (second price sealed bid) ❙ k -th price, all-pay,... ❚ Multi-unit 2. Basic auction types ❙ homogeneous-heterogeneous ❙ individual-combinatorial ❙ sequential-simultaneous ❙ one time-progressive ❙ discriminatory-uniform price Auctions - 8
PERFORMANCE MEASURES BI DDER AND SELLER CHARACTERI STI CS ❚ Valuation ❚ When choosing an auction design, a variety of criteria ❙ private values (each bidder knows his valuation) and measures may be used: ❙ common values (unknown common value for all bidders) ❙ social efficiency (maximize the total value to buyers) ❘ winner’s curse ❙ revenue (seller profit) ❚ Risk assessment ❙ bidder profit ❙ risk neutral ❙ time, complexity ❙ risk averse ❚ Why is it hard? Because of lack of information! ❚ Symmetry ❙ symmetrical (same distribution of valuation) Auction: incentive mechanism incentives 10 ❙ asymmetrical • buyer: maximizes expected profit seller • seller: maximizes optimality measure 20 Auctions - 9 Auctions - 10 ENGLI SH AUCTI ON BI DDER AND SELLER CHARACTERI STI CS (cont.) U = ❚ V Valuation V ❚ ascending bid, open-outcry Buyer i 3 i i ❙ = = + i private values , U V V V x ❚ item is sold if reserve price is met p = i i i V ❙ ∑ common values 2 = + U aV b V V V ❙ ❚ best strategy for bidder i V correlation i i j 2 i 4 ≠ j i (affiliation) V ❙ bid a small amount more than the previous high bid 1 ( h ) U until bidder’s valuation is reached, then stop ❚ Risk assessment ❚ auctioneer has great influence ❙ risk neutral r ❙ risk averse ❚ most emotional and competitive of auctions ❚ highly susceptible to rings = − h V p ❚ Symmetry h ❙ symmetrical V V ❙ asymmetrical 1 2 Auctions - 11 Auctions - 12
DUTCH AUCTI ON FI RST PRI CE SEALED BI D ❚ descending price, open-outcry ❚ first price V ❚ first price ❚ sealed (each bidder is ignorant of 1 b p other bids) ❚ auctioneer usually has no influence 1 V ❚ usually each participant is allowed 2 ❚ not very susceptible to rings b one bid 2 V ❚ two parts 3 price b ❙ bidding period 3 V 1 ❙ resolution phase (determination of the V 2 b winner) 1 V V b n 3 ❚ strategy : shade bids 2 b b V n 3 4 ❙ b to generate positive profit 4 ❙ to avoid winner’s curse (common value) Auctions - 13 Auctions - 14 VI CKREY AUCTI ON BASI C RESULTS ❚ second price, sealed ❚ the Dutch auction is strategically equivalent with the first price sealed bid auction (one-to-one mapping of (the item is awarded to the highest bidder at a V 1 strategies) ⇒ equivalent payoffs price equal to the second highest bid) b 1 ❚ dominant strategy : submit a bid equal to ❚ under private values, the English and the Vickrey p V 2 the true valuation (incentive compatibility) b auction are equivalent but not strategically (unlike in a 2 ❙ less fear of winner’s curse (common value) sealed bid, in an English auction bidders can respond to V 3 b rivals’ bids) 3 ❚ Revenue Equivalence Theorem: Any auction mechanism ′ = ′ ′ = ′ V V b V b b that satisfies (1-3) yields the same expected revenue V 1. Signals iid, continuous distribution, risk-neutral buyers b n n 2. object goes to buyer with highest signal 3. any buyer with lowest-possible signal expects zero profit Auctions - 15 Auctions - 16
The SIPV model Examples ❚ a single indivisible object Assume private value case, values iid, uniform on [0,1] 1 + n 1 ❚ private values r 0 1 = n = ( ) [ ] r E V V max { V ,..., V } + ( r ) ( r ) 1 n 1 ❚ all bidders are indistinguishable (symmetry) ... V V V V ( − ( 1 ) ( 2 ) 1 ) ( n ) n − ❚ valuations are independent and identically distributed 1 n = = * ( ) , English (or Vickrey) auction: b u u p + 1 and continuous random variables n − 1 n n = = ⇒ = * ❚ bidders and seller risk neutral ( ) , b u au p a a Dutch (or sealed bid first-price ) auction: + 1 n n ❚ RE Theorem: all auctions that award the item to the n u u highest bidder are payoff equivalent − 1 × = n Bidder’s expected gain = u n n Seller’s expected gain = p Auctions - 17 Auctions - 18 Examples (cont.) Participation fees ❚ Seller imposes participation fee c The expected revenue equivalence theorem does not imply same price dispersion ❚ Buyer participates only if his expected payoff is − − 1 1 1 n n F = = = = * * ( ) , ( ) , larger than c D 2 b u u b u u p n + E D F 1 n n E ❚ Fiercer competition = > higher seller expected U ( h ) revenue F D ❚ Positive probability of non-participation = > not F 1 1 1 E = p socially optimal 3 2 p h •Risk-averse sellers prefer the Duch auction Auctions - 19 Auctions - 20
Risk sensitive bidders Correlated beliefs ❚ In a first-price auction, risk averse users shade ❚ First price auctions generate lower prices less their bids than second price auctions ❙ higher average prices, more seller revenue, less ❙ small value bidders think that rival bidders are expected bidder profit also small, hence shade more their bids ❚ With risk averse bidders, the seller prefers the ❙ this convinces high valuation bidders to shade Duch to the English auction also more ❚ Revenue equivalence no longer applies Auctions - 21 Auctions - 22 Removing symetry Multi-unit auctions ❚ Different distributions for bidders ❚ k -units are for sale ❚ Revenue equivalence does not apply ❚ Bids are for single units ❚ First price auctions not socially optimal ❚ First price auction: k- highest bids win, each pays its bid ❚ Public authorities should use second price ❚ Second price auction: k- highest bids win, each auctions pays the value of the k + 1 highest bid (the highest > b > Pr{ b } 0 rejected bid) 1 2 a b c d ❚ Revenue equivalence applies Bidder 1 Bidder 2 ❙ not when bids are for several units Auctions - 23 Auctions - 24
Repeated auctions Collusion ❚ Bidders make collusive agreements to get the item ❚ Seller has k units available cheaper ❚ Uses sequential auctioning ❚ Which auctions are more collusive than others? ❚ Declining price anomaly ❙ Potential bidders select their designated winner (with the highest valuation), others commit to follow specific ❚ Example: 3 iid bidders, two items, Vickrey strategy (abstain from bidding) ❙ first round: bid less than usual, use option of ❙ Enforcement problem: incentives to keep the promise ❚ First price auctions: not self-enforcing! second round to get the item + ε , p ❙ winner places bidd = other bidders abstain ❙ second round: reduced competition min ❚ Second price auctions: self-enforcing! ❙ winner places bidd = value, others place zero bids Auctions - 25 Auctions - 26 Optimal auctions Common value auctions ❚ Value of bidder is not fixed before the auction ❚ Seller seeks to maximize expected revenue ❙ True value of item is known ex-ante ❚ Strategy: ❙ Value to bidder i depends on other bidder’s values ❙ use optimal participation fees + standard auctions ❙ examples: sealed box with coins, paintings, oil ❙ construct incentive compatible mechanisms ❚ Complex strategies, no general results ❘ make bidders reveal their valuations ❚ Winners curse: the winner discovers that he over ❘ allocate item to bidder with highest marginal revenue estimated the value of the item ❙ highest bid does not always win ❚ Solution: shade the bid to account for the adverse ❙ not socially efficient selection bias Auctions - 27 Auctions - 28
Recommend
More recommend