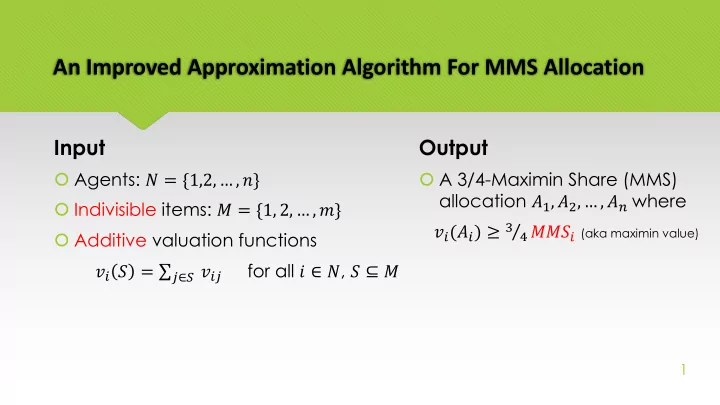
An Improved Approximation Algorithm For MMS Allocation Input Output - PowerPoint PPT Presentation

An Improved Approximation Algorithm For MMS Allocation Input Output š Agents: 𝑂 = {1,2, … , 𝑜} š A 3/4-Maximin Share (MMS) allocation 𝐵 % , 𝐵 & , … , 𝐵 ' where š Indivisible items: 𝑁 = {1, 2, … , 𝑛} ( ) 𝑁𝑁𝑇 ! (aka maximin value) 𝑤 ! (𝐵 ! ) ≥ ⁄ š Additive valuation functions 𝑤 ! 𝑇 = ∑ "∈$ 𝑤 !" for all 𝑗 ∈ 𝑂 , 𝑇 ⊆ 𝑁 1

MMS value / partition / allocation MMS allocation: 🍏 🍍 🍑 🍎 🥦 𝑤 ! (𝐵 ! ) ≥ 𝑁𝑁𝑇 ! Agents\items 👪 3 1 2 5 4 👩 4 4 5 3 2 👪 🍎🥦 🍍 🍍 👩 🍍 🍏 🍏🍑 👪 👩 🍏 🥦 🍑🍎 🍎🥦 🍎🥦 🍍🍑 value 7 8 value 9 9 MMS allocation might MMS value 7 MMS value 9 not exist, but 3/4-MMS 2 allocation always exist Finding MMS value is hard!

Algorithm Big Picture To show the existence of 3/4-MMS allocation: We assume MMS i is known for all 𝑗 ⟹ Scale valuations such that MMS i = 1 for all 𝑗 ⇒ 𝑤 ! 𝑁 ≥ 𝑜 š Step 1: Valid Reductions š Exist 𝑇 ⊆ 𝑁 and 𝑗 ∗ ∈ 𝑂 such that 𝑤 ! ∗ (𝑇) ≥ ( ⁄ % (𝑁) # $ )𝑁𝑁𝑇 ! ∗ %&' (𝑁\S) ≥ 𝑁𝑁𝑇 ! % (𝑁) for all 𝑗 ≠ 𝑗 ∗ š 𝑁𝑁𝑇 ! š Step 2: Generalized Bag Filling 3

Results Strongly Polynomial- time Algorithm for 3/4-MMS allocation n / ) M ( v i Existence of 3/4- MMS allocation More careful analysis MMS values are known Existence of PTAS for (3/4+1/(12n))- (3/4+1/(12n))-MMS PTAS MMS allocation allocation 4 MMS values are known