a
play

A Xz Of fixed points 3 Students HW example: # = For every - PowerPoint PPT Presentation

Aug 04, 2023 •469 likes •528 views

Questions Example: Returning HW (From notes.) I If I flip 20 coins, how many are heads ? Let X 3 = the number of fixed points Intro to Random Variables I If I enter a raffle with 9 other people every Permutation X 3 outcomes day, when will

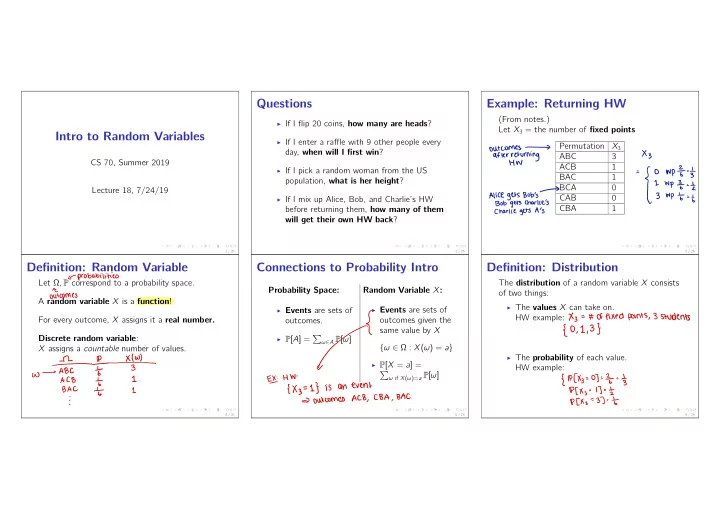
  1. Questions Example: Returning HW (From notes.) I If I flip 20 coins, how many are heads ? Let X 3 = the number of fixed points Intro to Random Variables I If I enter a raffle with 9 other people every Permutation X 3 outcomes → day, when will I first win ? after returning X 3 ABC 3 ' HW CS 70, Summer 2019 ACB 1 I ! :;÷÷ I If I pick a random woman from the US = BAC 1 population, what is her height ? BCA 0 . . Lecture 18, 7/24/19 - Wp 'T nice gets . . . - to CAB 0 I If I mix up Alice, Bob, and Charlie’s HW ' 's Charlie Bob gets CBA 1 before returning them, how many of them A 's gets Charlie will get their own HW back ? 1 / 26 2 / 26 3 / 26 Definition: Random Variable Connections to Probability Intro Definition: Distribution probabilities ← Let Ω , P correspond to a probability space. The distribution of a random variable X consists Probability Space: Random Variable X : a of two things: outcomes A random variable X is a function ! I The values X can take on. I Events are sets of I Events are sets of A Xz Of fixed points 3 Students HW example: # = For every outcome, X assigns it a real number. outcomes given the outcomes. , , 3 } { O I same value by X , Discrete random variable : I P [ A ] = P ! 2 A P [ ! ] { ! 2 Ω : X ( ! ) = a } X assigns a countable number of values. X I w ) I The probability of each value. r p I P [ X = a ] = - - HW example: 3 f- ABC - Z - I → P w ! if X ( ! )= a P [ ! ] O ] { IP C Xz H W EI : I A CB - t - - - 6 {115-1} event is an p [ X , I ] BAC I I 1 = = 6 BAC ACB CBA ; =3 ) outcomes I ⇒ . P [ X 3 , , - - ( 4 / 26 5 / 26 6 / 26

  2. Sanity Check! Working with RVs Functions of RVs Let X be a random variable with the following Same definition for X I What should the probabilities sum to, across distribution: all values X can take on? 1 8 1 wp 0.4 > 8 < 1 wp 0.4 1 X = wp 0.25 > 2 < 1 X = wp 0.25 I Can X take on negative values? Yes ! − 1 > wp 0.35 : 2 2 − 1 > wp 0.35 : 2 Write the distribution of f ( X ) , where f : R ! R . I Can X take on an infinite number of values? What is the probability that X is positive ? 0.4 Wp the - I ] for Raffle win can O ] DEX =L ] : PEX > PEX t = I Countable values? after # first any time aisoa.tv?Yt-f#Ywpo.zsfftz Of days 0.65 0.25 0.4 = t = I Uncountable values? 0.35 w p ) population Height in a → Is continuous RVs arts ! Later , ( 7 / 26 8 / 26 9 / 26 Functions of RVs Bernoulli Random Variable Bernoulli Example: Indicators Same definition for X Models whether one biased coin flip is a head. If X ∼ Bernoulli( p ) , and X = 1 corresponds to an = ) Models a yes/no -type question or event event A in an experiment: 8 1 wp 0.4 " Yes { 0,25 " = ) We say that X is an indicator for A . > < 1 Possible values of X : X = wp 0.25 2 not − 1 " > wp 0.35 : 2 I P [ X = ] = p Each day, if it is sunny in Berkeley with probability - p PEX 03=1 Write the distribution of X 2 . = 0 . 8 and cloudy with probability 0 . 2. . ⇐ Indicator for a sunny day? Parameters : p ÷÷¥ 1¥ : : : day for a sunny S indicator * = Notation: X ∼ Bernoulli( p ) S= { to 0.8 Wp . . . w p 0.2 . 10 / 26 11 / 26 12 / 26

  3. Binomial Random Variable Binomial Example: Weather I Binomial Example: Weather II Models how many heads are in n biased coin flips Each day, it is sunny in Berkeley with probability What is the probability that over a 10 day period, Ftp 0 . 8 and cloudy with probability 0 . 2. there are at least two sunny days? I ] complement = ) Models a sum of independent, identically Weather across days is independent. Pf SE 1 c- IPCSZ 2) - = distributed ( i.i.d ) Bernoulli( p ) RVs. - PCS =D What is the probability that over a 10 day period, O ] PCS 1- , n } - { 0 = - Possible values of X : 1 there are exactly 5 sunny days? , , . - , 0.8 ) . . BINGO days - - i S sunny over 10 # distribution - f 7) pill = . IP fatter - PJ Enos ] use ? n = 10 , p = 0.8 P [ X = i ] = - Binh , p ) S IP [5--0]=(18/10.8)%0.2110--9 ~ PCS ; ' f) . - ( IF ? ] 0.29 - - 17=(1,0×0.8/40.2) Parameters : n , p 10.850.25 9 0.8 10 - PCS = - - w - i - - pgn pi ( ni ) a Notation: X ∼ Bin( n , p ) 1010.8/10.239 " 223=1-0.2 ) PCS + Sat - tsn 5=5 . 8) Iif Si Berto Si is , Let - day i - - . - - . sunny . 13 / 26 14 / 26 15 / 26 Break Geometric Random Variable Probabilities Sum To 1 ? pcx=i Geomlp ) X - Models how many biased coin flips I need until Not obvious that the probabilities sum to 1. - up ] PCH ] ) P my first head. - . 1 ~ - p5pt - p ) X ( 1 − p ) i − 1 p = ptcl Ptu . . = ) Models time until a “success” when . i = 1 performing i.i.d. trials with success probability p What is your real favorite movie, and what movie Each term is the previous × the same multiplier. positive do you pretend is your favorite to sound cultured? Possible values of X : 1) 2,3 { a , ar , ar 2 , ar 3 , . . . } is a geometric sequence . integers , . . . . stgtafigge Fratto win pfi fall " I 1 - , P [ X = i ] = a ar i − 1 = X mens%fe.gs/=H-PXtp7...Ci-p)p--Ci-p)i-lp ftp.ya.FI#..1 1 − r need 0 i = 1 . Parameters : Success probability p Notation: X ∼ Geometric( p ) 16 / 26 17 / 26 18 / 26

  4. Aside: The Formula Geometric Example: Ra ffl e I Geometric Example: Ra ffl e II Dfw -581=1 iorartsfetime . An easy way to recreate the formula? I enter a ra ffl e with 9 other people every day. What is the probability that I win the ra ffl e some Each day, a winner is chosen independently, and time on or before the 8th day?? all Of SUM Let S = a + ar + ar 2 + . . . - pfWZ9 ] ← with equal probability. terms . r distributing Key Idea: - lpftogsersfordays ) =L → What is the probability that I win for the first d → r · S = ar + ar 2 + ar 3 + · · · = S a time on the 5th day? - ' = day C Fol of first WIN =L W - . Solve for S ! p = ÷ r S S a - - - If X ∼ Geometric( p ) , then: Fr s = " I I S S ( fo )4( wt ) r 5 ] a = - PC iwtses ] - p ) Ipfw Ci - = P [ X i ] = - I - r , - sci a = - " " doesn't I r I work If 21 S → a = . , 19 / 26 20 / 26 21 / 26 Poisson Random Variable Probabilities Sum To 1 ? Poisson Example: Typos Models number of rare events over a time period Again, not obvious that the probabilities sum to 1. (Notes.) We make on average 1 typo per page. " €7 of xme = ) Use the “rate” of event per unit time. The number of typos per page is modeled by a 1 λ i i ! e − λ = X / ' Poisson( λ ) random variable. e no innings :S at 2 Possible values of X : , , . . i = 0 Tiki , . . et P [ X = i ] = λ i What is the probability that a single page has i ! e − λ a exactly 5 typos? - X . e I " page e = typos per # T . = = λ = I Parameters : Rate λ Taylor Series for e x : = if , = Toe = to ' s ] e- ' Notation: X ∼ Poisson( λ ) e- BET 1 x i = e x = X i ! i = 0 22 / 26 23 / 26 24 / 26

  5. Poisson Example: Typos Summary We type 200 pages. The pages are all I Random variables assign numbers to independent. What is the probablity that at least outcomes. one page has exactly 5 typos? . ] p [ of F) ? n teas f- ! p [ Pg I Treat X = i as any ordinary event. at z - = " I Ya g es 200 I Bernoulli, Binomial, and Geometric RVs have fazes y ) . [ p [ 1 I - = nice interpretations via biased coin flips . - [ I " ° # I Practice modeling real world events as I = - , previous Bernoulli, Binomial, Geometric, Poisson. I page 25 / 26 26 / 26

Recommend

More recommend