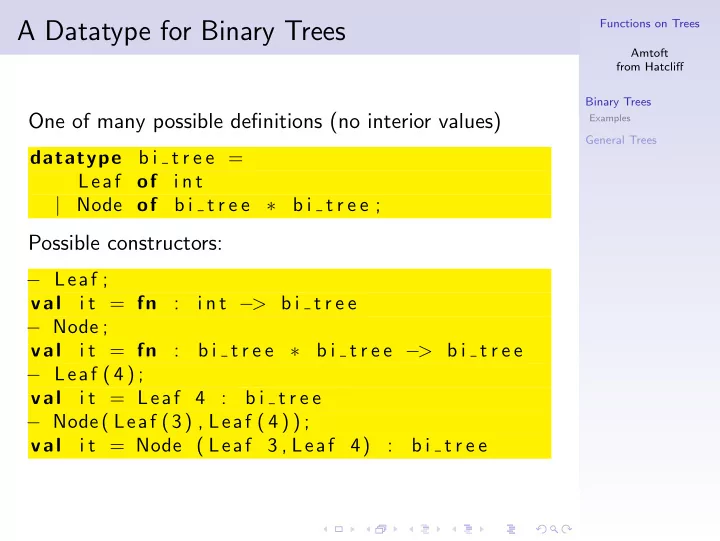
A Datatype for Binary Trees Functions on Trees Amtoft from - PowerPoint PPT Presentation

A Datatype for Binary Trees Functions on Trees Amtoft from Hatcliff Binary Trees One of many possible definitions (no interior values) Examples General Trees datatype b i t r e e = Leaf i n t of | Node of b i t r e e ∗ b i t r e e ; Possible constructors: − Leaf ; val i t = fn : i n t − > b i t r e e − Node ; val i t = fn : b i t r e e ∗ b i t r e e − > b i t r e e − Leaf ( 4 ) ; val i t = Leaf 4 : b i t r e e − Node ( Leaf (3) , Leaf ( 4 ) ) ; val i t = Node ( Leaf 3 , Leaf 4) : b i t r e e

Recursion Template Functions on Trees Amtoft from Hatcliff Binary Trees b i t r e e = datatype Examples Leaf i n t of General Trees | Node of b i t r e e ∗ b i t r e e ; Recall our guiding principle: A recursive function follows the structure of inductively-defined data. Recursion template: t r e e r e c ( Leaf (n )) = . . . . fun | t r e e r e c ( Node ( bt1 , bt2 )) = . . . t r e e r e c ( bt1 ) . . . . . . t r e e r e c ( bt2 ) . . . ;

Sum of Tree Data Functions on Trees Amtoft from Hatcliff Sample tree: Binary Trees Examples t = Node ( Node ( Leaf (3) , Leaf ( 4 ) ) , Leaf ( 1 ) ) ; − val General Trees t = Node ( Node ( Leaf #, Leaf #), Leaf 1) val : b i t r e e Desired behavior − use ” t r e e l i b r a r y . sml” ; . . . tree sum = fn : b i t r e e − > i n t val − tree sum ( t ) ; i t = 8 : i n t val Implementation: tree sum ( Leaf (n )) = n fun | tree sum ( Node ( bt1 , bt2 )) = tree sum ( bt1 ) + tree sum ( bt2 ) ;

Flipping a Tree Functions on Trees Amtoft from Hatcliff t = Node ( Node ( Leaf (3) , Leaf ( 4 ) ) , Leaf ( 1 ) ) ; − val Binary Trees t = Node ( Node ( Leaf #, Leaf #), Leaf 1) val Examples : b i t r e e General Trees Desired behavior − use ” t r e e l i b r a r y . sml” ; . . . t r e e f l i p = fn : b i t r e e − > b i t r e e val − t r e e f l i p ( t ) ; i t = Node val ( Leaf 1 , Node ( Leaf #,Leaf #)) : b i t r e e Implementation: fun t r e e f l i p ( Leaf (n )) = Leaf (n) | t r e e f l i p ( Node ( bt1 , bt2 )) = Node ( t r e e f l i p ( bt2 ) , t r e e f l i p ( bt1 ) ) ;

Fringe of a Tree Functions on Trees Amtoft from Hatcliff Binary Trees t = Node ( Node ( Leaf (3) , Leaf ( 4 ) ) , Leaf ( 1 ) ) ; − val Examples t = Node ( Node ( Leaf #, Leaf #), Leaf 1) val General Trees : b i t r e e Desired behavior − use ” t r e e l i b r a r y . sml” ; . . . t r e e f r i n g e = fn : b i t r e e − > i n t l i s t val − t r e e f r i n g e ( t ) ; i t = [ 3 , 4 , 1 ] : i n t l i s t val Implementation: t r e e f r i n g e ( Leaf (n )) = [ n ] fun | t r e e f r i n g e ( Node ( bt1 , bt2 )) = t r e e f r i n g e ( bt1 ) @ t r e e f r i n g e ( bt2 ) ;

A Datatype for General Trees Functions on Trees Amtoft from Hatcliff Trees with arbitrary branching (still no interior values) Binary Trees ’ a g t r e e = datatype Examples Leaf ’ a of General Trees | Node of ’ a branches ’ a branches = and Empty | Branch of ’ a g t r e e ∗ ’ a branches ; A recursive function still follows the structure of inductively-defined data. Recursion template now involves mutual recursion: g t r e e x ( Leaf (n )) = . . . fun | g t r e e x ( Node ( bs )) = . . . branches x ( bs ) . . . and branches x (Empty ) = . . . | branches x ( Branch ( gt , bs )) = . . . g t r e e x ( gt ) . . . . branches x ( bs ) . . . ;

Sum of Tree Data Functions on Trees Amtoft from Hatcliff Binary Trees Examples ’ a g t r e e = datatype General Trees Leaf ’ a of | Node of ’ a branches ’ a branches = and Empty | Branch of ’ a g t r e e ∗ ’ a branches ; fun gtree sum ( Leaf (n )) = n | gtree sum ( Node ( bs )) = branches sum ( bs ) and branches sum ( Empty ) = 0 | branches sum ( Branch ( gt , bs )) = gtree sum ( gt ) + branches sum ( bs ) ;

Lifting to Higher-Order Functions Functions on Trees Amtoft from Hatcliff ’ a g t r e e = datatype Binary Trees Leaf ’ a of Examples | Node of ’ a branches General Trees ’ a branches = and Empty | Branch of ’ a g t r e e ∗ ’ a branches ; As for lists, we can write a map function gtree map f ( Leaf (n )) = Leaf ( f (n )) fun | gtree map f ( Node ( bs )) = Node ( branches map f bs ) and branches map f (Empty ) = Empty | branches map f ( Branch ( gt , bs )) = Branch ( gtree map f gt , branches map f bs ) ; gtree add1 = gtree map ( fn x = > x + 1 ) ; val What about filter or fold?