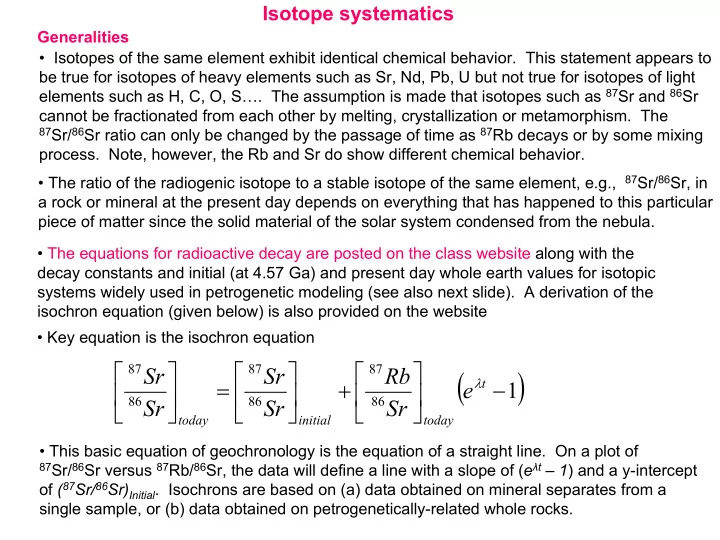

Isotope systematics Generalities • Isotopes of the same element exhibit identical chemical behavior. This statement appears to be true for isotopes of heavy elements such as Sr, Nd, Pb, U but not true for isotopes of light elements such as H, C, O, S…. The assumption is made that isotopes such as 87 Sr and 86 Sr cannot be fractionated from each other by melting, crystallization or metamorphism. The 87 Sr/ 86 Sr ratio can only be changed by the passage of time as 87 Rb decays or by some mixing process. Note, however, the Rb and Sr do show different chemical behavior. • The ratio of the radiogenic isotope to a stable isotope of the same element, e.g., 87 Sr/ 86 Sr, in a rock or mineral at the present day depends on everything that has happened to this particular piece of matter since the solid material of the solar system condensed from the nebula. • The equations for radioactive decay are posted on the class website along with the decay constants and initial (at 4.57 Ga) and present day whole earth values for isotopic systems widely used in petrogenetic modeling (see also next slide). A derivation of the isochron equation (given below) is also provided on the website • Key equation is the isochron equation ( ) 87 87 87 Sr Sr Rb = + λ − t e 1 86 86 86 Sr Sr Sr today initial today • This basic equation of geochronology is the equation of a straight line. On a plot of 87 Sr/ 86 Sr versus 87 Rb/ 86 Sr, the data will define a line with a slope of ( e λ t – 1 ) and a y-intercept of ( 87 Sr/ 86 Sr) Initial . Isochrons are based on (a) data obtained on mineral separates from a single sample, or (b) data obtained on petrogenetically-related whole rocks.
Example of a whole rock isochron determined on Example of internal isochron determined on whole rock samples from the Stillwater Complex mineral separates from a Stillwater gabbro From: DePaolo and Wasserburg (1979) Geochim Cosmochim Acta 43, 999
Some common isotopic systems with long half lives used as tracers in igneous processes Radioactive Decay Constant Ratio measured Whole earth values at decay λ - (yr -1 ) 4.57Ga present 87 Rb → 87 Sr ( β ) 1.42 x 10 -11 87 Sr/ 86 Sr 0.69898 ~0.7047 147 Sm → 143 Nd ( α ) 6.54 x 10 -12 143 Nd/ 144 Nd 0.506631 0.512638 176 Lu → 176 Hf ( β ) 1.94 x 10 -11 176 Hf/ 177 Hf 0.27978 0.28290 238 U → 206 Pb (8 α ) 1.55125 x 10 -10 206 Pb/ 204 Pb 9.307 ~18.70 235 U → 207 Pb (7 α ) 9.8485 x 10 -10 207 Pb/ 204 Pb 10.294 ~15.63 232 Th → 208 Pb (6 α ) 4.95 x 10 -11 208 Pb/ 204 Pb 29.476 ~38.63 187 Re → 187 Os ( β ) 1.666 x 10 -11 187 Os/ 188 Os 0.09526 0.12863 Notes: 1. At present day: 238 U/ 235 U = 137.88 2. Values for whole earth at 4.57 Ga obtained from meteorites. Values for whole earth at 0 Ga are based on meteorite values for Nd isotopes and ε Nd vs. ε Sr correlations. 3. Geochron: 207 Pb/ 204 Pb = 0.617976 206 Pb/ 204 Pb + 4.524501 4. The isotopic ratio measured in a sample today represent the sum total of all the processes that have affected this particular piece of matter since the earth accreted 5. Isotopes of the same (heavy) element (those listed above) cannot be fractionated from each other by natural processes of melting, crystallization or recrystallization. Of course, this is not true for lighter elements such as H, C, O, S 87 87 Sr Sr [ ] [ ] + − 6. Mixing Equation for Sr for two components X Sr ( 1 X ) Sr 1 1 1 2 86 86 Sr Sr 87 Sr (similar equation for other systems) = 1 2 [ ] [ ] 2 86 + − Sr X Sr ( 1 X ) Sr 1 1 1 MIX 7. Hf and Nd isotopes are strongly correlated
What is the geochron? (yr -1 ) λ Ratio measured @ 4.57Ga at present 238 U → 206 Pb 1.55125 x 10 -10 206 Pb/ 204 Pb 9.307 ~18.70 235 U → 207 Pb 9.84850 x 10 -10 207 Pb/ 204 Pb 10.294 ~15.63 Values of 206 Pb/ 204 Pb and 207 Pb/ 204 Pb @ 4.57Ga determined on troilite (FeS) in iron meteorites ( ) 206 206 238 Pb Pb U = + λ − t e 1 238 U/ 204 Pb (µ), 235 U/ 204 Pb (µ/137.88), t =4.57 Ga 204 204 204 Pb Pb Pb today initial today 207 207 235 ( ) Pb Pb U = + λ − t 18 e 1 204 204 204 Pb Pb Pb µ=10 today initial today 16 8 206 Pb/ 204 Pb µ/137.88 207 Pb/ 204 Pb µ 207 Pb/ 204 Pb Geochron 14 6 2 11.3705 2/137.88 11.5734 4 13.434 4/137.88 12.8528 4 6 15.497 6/137.88 14.1322 12 2 8 17.561 8/137.88 15.4117 10 19.625 10/137.88 16.6911 10.294 10 9.307 Geochron represents lead in the earth and 8 meteorites that have evolved in a single-stage 14 20 10 16 18 8 12 from 4.57 to the present day. The geochron 206 Pb/ 204 Pb defines a line in Pb-Pb space with the equation 207 Pb/ 204 Pb = 0.617976 206 Pb/ 204 Pb + 4.524501 Why is there no equivalent geochron for 208 Pb/ 204 Pb?
Isotope evolution diagrams (similar diagrams for Lu-Hf and Re-Os systems) 0.512638 0.7047 Chondrite Uniform Uniform reservoir Reservoir (CHUR) 144 Nd/ 143 Nd evolution line evolution line 87 Sr/ 86 Sr 0.69898 0.506631 0 0 Time (Ga) Time (Ga) 4.57 4.57 How well do we know the values at 4.567Ga? Amitsoq gneiss During melting: Average N. American (Rb/Sr) melt > (Rb/Sr) source shield (~0.718) ( 87 Sr/ 86 Sr) melt = ( 87 Sr/ 86 Sr) source Average crust (~0.712) sea water 0.709 Sr enters Ca-rich minerals, Whole earth (0.7047) especially plagioclase. Rb is Upper mantle essentially excluded from all (~0.7025) depleted 0.69898 minerals except micas. 0 4.57 Time (Ga)
Nd isotopes It is conventional to use the ε notation when discussing Nd isotopic ratios. In this notation, the ratios are compared to those of the chondritic uniform reservoir (CHUR). 143 Nd sample − 144 ε = 4 ( t ) [ 1 ] x 10 143 Nd 144 CHUR e.g., for a zero age MORB, 143 Nd/ 144 Nd ratio = 0.513166 and CHUR at zero age = 0.512638 0 . 513166 ε = − 4 [ 1 ] x 10 = + 10.3 Nd 0 . 512638 As a general rule, during melting and/or crystallization, (Sm/Nd) melt < (Sm/Nd) crystals which means that the residue is depleted in Nd relative to Sm and the melt is enriched in Nd relative to Sm 20 REE in sample/REE in chond Evolution of depleted mantle A = crust 10 a, b, c: model ages c b a CHUR 1 ε Nd 0 CHUR B = mantle Crust derived -10 from mantle at different times -20 La Nd Sm Yb 0 4.6 time (Ga)
Mantle Correlation curve DMM: Depleted Morb Mantle PUM: Primitive Upper Mantle (DMM) 0.7047 As a general rule, the 10 Mantle array lower left quadrant and f Sm/Nd = 0 the upper right quadrant f Rb/Sr = 6 (PUM) contain few data points. 0 ε Nd Sm Upper crust Nd = − basalt f 1 Sm f Sm/Nd = -0.4 -10 Nd f Rb/Sr = 6 CHUR Many, but by no means not -20 all, crustal rocks plot in the Lower crust lower right quadrant with f Sm/Nd = -0.4 older samples plotting further f Rb/Sr = 0 -30 from whole earth values 0 50 100 150 -50 ε Sr Mantle array includes samples believed to be derived directly from the mantle with minimal or zero crustal input. Most such samples have +ve ε Nd and –ve ε Sr . MORB, OIB, IAB, some CAB, and many continental flood basalts fall in this range. When this correlation was first observed by DePaolo and Wasserburg (1976) they postulated two main isotopic reservoirs in the mantle: DMM (Depleted Morb Mantle at ε Nd ~10-12) and PUM (Primitive Upper Mantle with ε Nd around 0). This is now known to be an oversimplification.
Some details of the mantle array defined by MORB (mid Ocean Ridge Basalts) and OIB (Ocean Island basalts) +13 0.7047 ε Nd 0.512638 0
Isotopic ratios as indicators of mixing processes Since there is commonly a large difference in the isotopic compositions of crust and mantle, isotopic ratios can provide information on the extent of mixing between these two reservoirs. Mixing is primarily due to assimilation of crustal material by mantle-derived magmas although many different types of mixing can occur. The equation below is the mixing equation for Sr isotopes. Similar equations exist for the other isotopic systems. 87 87 Sr Sr [ ] [ ] + − X Sr ( 1 X ) Sr 1 1 1 2 86 86 87 Sr Sr Sr = 1 2 [ ] [ ] 86 + − Sr X Sr ( 1 X ) Sr 1 2 1 1 MIX X 1 and X 2 are the wt. fractions of components 1 and 2, Sr 1 and Sr 2 are the abundances of Sr in the two components, ( 87 Sr/ 86 Sr) 1 and ( 87 Sr/ 86 Sr) 2 are the isotopic ratios of the two components On a plot of ε Nd vs. ε Sr , mixing lines are hyperbolas 1 K = (Sr/Nd) 1 /(Sr/Nd) 2 K = 0.1 0.5 K = 1 ε Nd If 1 refers to mantle and 2 refers to crust, 5 assimilation will tend to move the mix K = 10 along a line where K>1 2 ε Sr After: DePaolo and Wasserburg (1979) Geochimica Cosmochimica Acta 43, 615
Recommend
More recommend