8.3 GRAPH REPRESENTATIONS AND GRAPH ISOMORPHISM INCIDENCE TABLE - PDF document

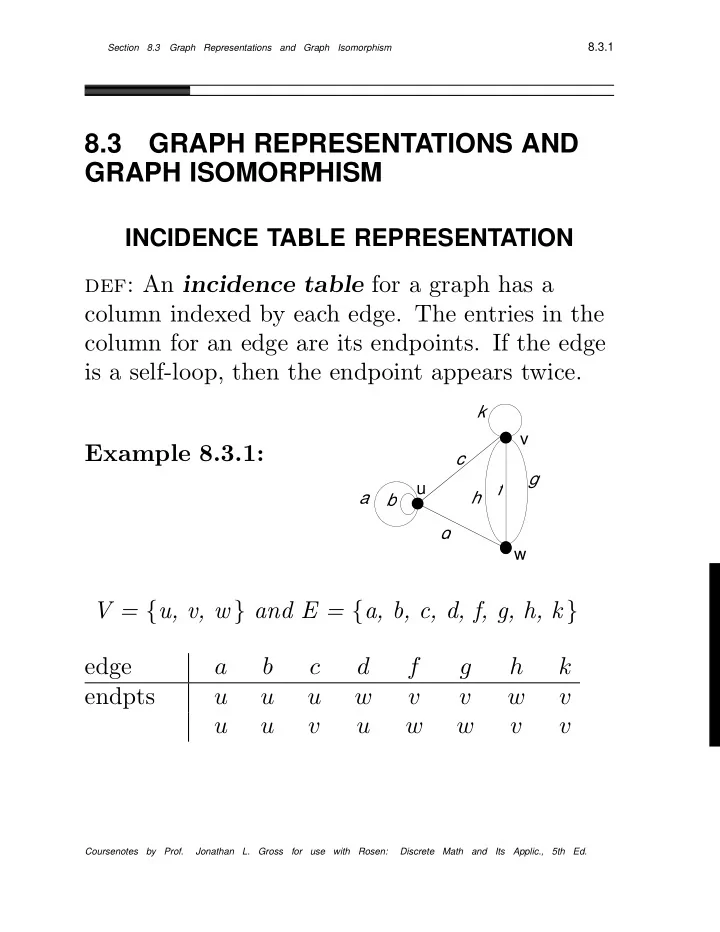
8.3.1 Section 8.3 Graph Representations and Graph Isomorphism 8.3 GRAPH REPRESENTATIONS AND GRAPH ISOMORPHISM INCIDENCE TABLE REPRESENTATION def: An incidence table for a graph has a column indexed by each edge. The entries in the column for an edge are its endpoints. If the edge is a self-loop, then the endpoint appears twice. k v Example 8.3.1: c g u f a h b d w V = { u, v, w } and E = { a, b, c, d, f, g, h, k } edge a b c d f g h k endpts u u u w v v w v u u v u w w v v Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.2 Chapter 8 GRAPH THEORY INCIDENCE MATRIX REPRESENTATION k Example 8.3.1, continued: v c g u f a h b d w a b c d f g h k 2 2 1 1 0 0 0 0 u. 0 0 1 0 1 1 1 2 v. 0 0 0 1 1 1 1 0 w. Incidence matrices waste space on all the zeroes. However, they are sometimes useful in conceptualization. Thm 8.2.1. (Euler’s Thm, revisited) The sum of the degrees of a graph equals 2 | E | . The degrees of a graph are the row Proof: sums of its incidence matrix. Thus, the sum of degrees equals the sum of the row sums. There is a column for each edge, and every column sum equals 2. Thus, 2 | E | equals the sum of the col- umn sums. The sum of the row sums equals the sum of the column sums. ♦ Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.3 Section 8.3 Graph Representations and Graph Isomorphism ADJACENCY LIST REPRESENTATION def: An adjacency list for a vertex v of a graph G is a list containing each vertex w of G once for each edge between v and w . def: An adjacency list representation of a graph is a table of all the adjacency lists. k Example 8.3.1, continued: v c g u. u u v w u f a h b v. u v w w w d w. u v v v w ADJACENCY MATRIX REPRESENTATION u v w 2 1 1 u. 1 1 3 v. 1 3 0 w. Remark : Lots of wasted space. Clumsy for self- loops. Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.4 Chapter 8 GRAPH THEORY GRAPH ISOMORPHISM The Greek root “iso” means “same”. The Greek root “morphism” means “form”. An isosceles triangle has two Example 8.3.2: edges that are the same length. isosceles triangle Two molecules with the same Example 8.3.3: chemical formula are called isomers . H H H H C C C C C C H H H H H H H H C C C C C C C H H C H H H H H H butane isobutane Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.5 Section 8.3 Graph Representations and Graph Isomorphism And now for graphs. How are these the same?? def: The graphs G and H are isomorphic if there exists a one-to-one onto function f : V G → V H such that ∀ u, v ∈ V G , the number of edges between f ( u ) and f ( v ) equals the number of edges between u and v . Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.6 Chapter 8 GRAPH THEORY SIMPLE ISOMORPHISM Prop 8.3.2. Two simple graphs G and H are isomorphic if and only if there if a bijection f : V G → V H such that vertices f ( u ) and f ( v ) are adjacent in H if and only if vertices u and v are adjacent in G . The graph mapping f is an Example 8.3.4: isomorphism. u v f(v) a f(x) f(a) f(b) e d b f(d) f(c) c x w f(u) f(w) f(e) Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.7 Section 8.3 Graph Representations and Graph Isomorphism Clearly, two isomorphic graphs have • the same number of vertices • the same number of edges • the same degree sequence But this is not enough! Two nonisomorphic graphs Example 8.3.5: with the same degree sequence. Two more nonisomorphic Example 8.3.6: graphs with the same degree sequence. Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.8 Chapter 8 GRAPH THEORY GRAPH ISOMORPHISM TESTING Are these graphs isomorphic? Example 8.3.7: 0 2 4 1 3 5 K 3,3 ML 3 Are these graphs isomorphic? Example 8.3.8: Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.9 Section 8.3 Graph Representations and Graph Isomorphism Are these graphs isomorphic? Example 8.3.9: 0 2 4 CL 3 1 3 5 K 3,3 From Final Exam May 1993. Example 8.3.10: 2 1 6 3 6b (10) 5 4 Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.10 Chapter 8 GRAPH THEORY From Dec 1993. Example 8.3.11: From May 1994. Example 8.3.12: Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.

8.3.11 Section 8.3 Graph Representations and Graph Isomorphism From GTAIA Example 8.3.13: A B C D E No two of these graphs are isomorphic. Remark : Prop 8.4.2 (next section) facilitates a brief explanation why. Coursenotes by Prof. Jonathan L. Gross for use with Rosen: Discrete Math and Its Applic., 5th Ed.