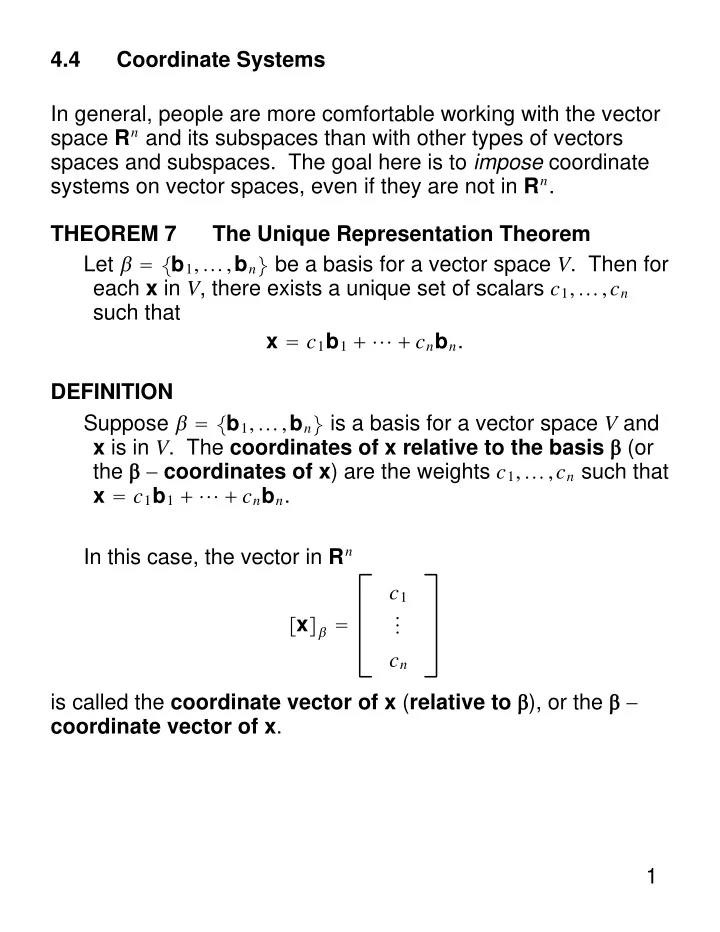
4.4 Coordinate Systems In general, people are more comfortable - PDF document

4.4 Coordinate Systems In general, people are more comfortable working with the vector space R n and its subspaces than with other types of vectors spaces and subspaces. The goal here is to impose coordinate systems on vector spaces, even if they are not in R n . THEOREM 7 The Unique Representation Theorem Let β =  b 1 , … , b n  be a basis for a vector space V . Then for each x in V , there exists a unique set of scalars c 1 , … , c n such that x = c 1 b 1 + ⋯ + c n b n . DEFINITION Suppose β =  b 1 , … , b n  is a basis for a vector space V and x is in V . The coordinates of x relative to the basis β (or the β − coordinates of x ) are the weights c 1 , … , c n such that x = c 1 b 1 + ⋯ + c n b n . In this case, the vector in R n c 1  x  β = ⋮ c n is called the coordinate vector of x ( relative to β ), or the β − coordinate vector of x . 1

3 EXAMPLE: Let β =  b 1 , b 2  where b 1 = and 1 0 1 b 2 = and let E =  e 1 , e 2  where e 1 = and 1 0 0 e 2 = . 1 Solution: 2 If  x  β = , then 3 3 0 x = ____ . + ____ = 1 1 6 If  x  E = , then 5 1 0 x = ____ + ____ . = 0 1 2

x 2 7 6 5 4 3 2 1 x 1 1 2 3 4 5 6 7 Standard graph paper β − graph paper 3

From the last example, 6 3 0 2 . = 5 1 1 3 For a basis β =  b 1 , … , b n  , let c 1 c 2 b 1 b 2 ⋯ b n and  x  β = P β = ⋮ c n Then x = P β  x  β . We call P β the change-of-coordinates matrix from β to the standard basis in R n . Then − 1 x  x  β = P β − 1 is a change-of-coordinates matrix from the and therefore P β standard basis in R n to the basis β . 4

3 0 EXAMPLE: Let b 1 = , b 2 = , β =  b 1 , b 2  and 1 1 6 x = . Find the change-of-coordinates matrix P β from β to 8 the standard basis in R 2 and change-of-coordinates matrix P β − 1 from the standard basis in R 2 to β . Solution b 1 b 2 and so P β = = − 1 1 0 3 0 3 − 1 = P β = − 1 1 1 1 3 6 2 − 1 to find  x  β = (b) If x = , then use P β . 8 6 1 0 6 3 − 1 x = Solution:  x  β = P β = − 1 1 8 3 5

Coordinate mappings allow us to introduce coordinate systems for unfamiliar vector spaces. Standard basis for P 2 :  p 1 , p 2 , p 3  =  1, t , t 2  Polynomials in P 2 behave like vectors in R 3 . Since a + bt + ct 2 = ____ p 1 + ____ p 2 + ____ p 3 , a  a + bt + ct 2  β = b c We say that the vector space R 3 is isomorphic to P 2 . 6

EXAMPLE: Parallel Worlds of R 3 and P 2 . Vector Space R 3 Vector Space P 2 a Vector Form: a + bt + bt 2 Vector Form: b c Vector Addition Example Vector Addition Example − 1 2 1  − 1 + 2 t − 3 t 2  +  2 + 3 t + 5 t 2  2 3 5 + = = 1 + 5 t + 2 t 2 − 3 5 2 Informally, we say that vector space V is isomorphic to W if every vector space calculation in V is accurately reproduced in W , and vice versa . Assume β is a basis set for vector space V . Exercise 25 (page 254) shows that a set  u 1 , u 2 , … , u p  in V is linearly independent if and only if  u 1  β ,  u 2  β , … ,  u p  β is linearly independent in R n . 7

EXAMPLE: Use coordinate vectors to determine if  p 1 , p 2 , p 3  is a linearly independent set, where p 1 = 1 − t , p 2 = 2 − t + t 2 , and p 3 = 2 t + 3 t 2 . Solution: The standard basis set for P 2 is β =  1, t , t 2  . So  p 1  β = ,  p 2  β = ,  p 3  β = Then 1 2 0 1 2 0 − 1 − 1 2 0 1 2  ⋯  0 1 3 0 0 1 By the IMT,  p 1  β ,  p 2  β ,  p 3  β is linearly ____________________ and therefore  p 1 , p 2 , p 3  is linearly ____________________. Coordinate vectors also allow us to associate vector spaces with subspaces of other vectors spaces. 8

3 EXAMPLE Let β =  b 1 , b 2  where b 1 = and 3 1 0 9 b 2 = and let H = span  b 1 , b 2  . Find  x  β , if x = . 1 13 3 15 Solution: (a) Find c 1 and c 2 such that 3 0 9 c 1 + c 2 3 1 13 = 1 3 15 Corresponding augmented matrix: 3 0 9 1 0 3 3 1 13 0 1 4 ∽ 1 3 15 0 0 0 Therefore c 1 = ____ and c 2 = _____ and so  x  β = . 9

x 3 x 2 x 1 9 3 in R 3 is associated with the vector in R 2 13 4 15 H is isomorphic to R 2 10