4/30/2014 Non-Volatile Storage: Magnetic Random Access Memory - PDF document

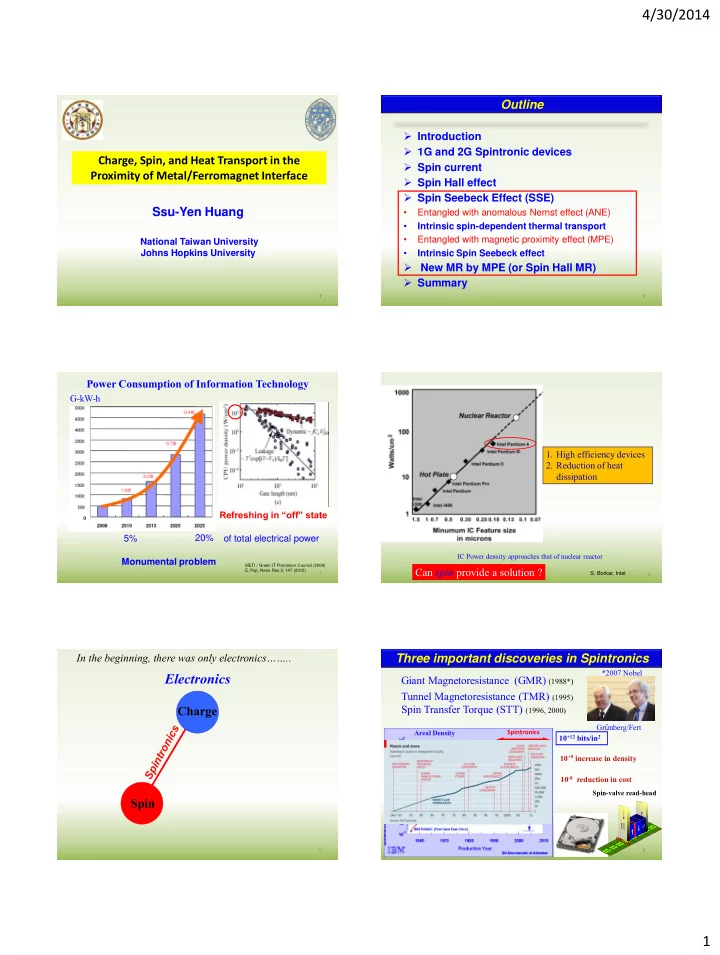
4/30/2014 Outline  Introduction  1G and 2G Spintronic devices Charge, Spin, and Heat Transport in the  Spin current Proximity of Metal/Ferromagnet Interface  Spin Hall effect  Spin Seebeck Effect (SSE) Ssu-Yen Huang • Entangled with anomalous Nernst effect (ANE) • Intrinsic spin-dependent thermal transport • Entangled with magnetic proximity effect (MPE) National Taiwan University • Johns Hopkins University Intrinsic Spin Seebeck effect  New MR by MPE (or Spin Hall MR)  Summary 1 1 2 2 Power Consumption of Information Technology G-kW-h 1. High efficiency devices 2. Reduction of heat dissipation Refreshing in “off” state 5% of total electrical power 20% IC Power density approaches that of nuclear reactor Monumental problem METI / Green IT Promotion Council (2008) Can spin provide a solution ? E. Pop, Nano Res 3, 147 (2010) 3 S. Borkar, Intel 4 In the beginning, there was only electronics…….. Three important discoveries in Spintronics Giant Magnetoresistance (GMR) (1988*) *2007 Nobel Electronics Tunnel Magnetoresistance (TMR) (1995) Charge Spin Transfer Torque (STT) (1996, 2000) Gr ü nberg/Fert Areal Density Spintronics 10 +12 bits/in 2 10 +9 increase in density GMR AMR 10 -8 reduction in cost Spin-valve read-head Spin 5 6 1

4/30/2014 Non-Volatile Storage: Magnetic Random Access Memory Spintronic GMR and TMR Devices (MRAM) GMR TMR P AP “1” Magnetic Tunnel Junction (MTJ) “0” “1” FM1 FM1 free Storage Write insulator metal Read high R Reference FM2 FM2 fixed Low R High R “0” Field Sensing & Non-Volatile Storage Spin-dependent Spin-selective low R scattering tunneling word / sense Advantages: lines Non-volatile memory Key Challenges: High density Short access time Eliminate field writing Low power consumption Universal memory: speed as SRAM, density as DRAM, rewritability as flash Field (1G) Devices 7 8 Spin transfer torque 1G and 2G Spintronic Devices Field Sensing “1” electrical current affects magnetic configurations & Non-Volatile Storage “0” I > I C Storage AP M P torque  sin  Reference High R  Low R Incident electron transmitted e - I reflected without a magnetic field Large M : spin polarizer Small M : M can be rotated (1G) Field Devices (2G) Current (STT )Devices Slonczewski, JMMM 159 , L1 (1996) Requires very large j c > 10 6 A/cm 2 !! Berger, PR B 54 , 9353 (1996), JAP 57 , 1266 (1984), JAP 49 , 2156 (1978) What are new Spintronic Effects for 3G devices? Waintal et al ., PRB 62 , 12317 (2000) 9 10 Various Hall effects Charge, Spin, Thermal Transport in thin films Ordinary Hall effect (E. H. Hall, 1879) y z B ( or M  ) V y x Anomalous Hall effect (E. H. Hall, 1880)  T x  T x j e j e Integer quantum Hall effect (von Klitzing, Nobel 1985) Fractional quantum Hall effect (Stormer, Tsui, Laughlin, Nobel 1998) V y Spin Hall effect E  V  B Inverse spin Hall effect Magnon Hall effect Edwin Hall (1879, 1880) Walther Nernst A student of Henry Rowland @ JHU Topological Hall effect  T x : Nernst Effect  V x : Hall Effect maybe more… 11 12 12 2

4/30/2014 Hall effect Anomalous Hall effect Spin Hall effect The mechanism of SHE Electron frame 1879 1880 2004 Spin-Orbit Coupling “sees” B  field with gradient B E + Spin-Orbit Coupling Lorentz Force nucleus Charge + Spin Only Spin Only Charge Detect by voltage Detect by voltage Why? Detect by what ? F =q (E + V  B) AHE can be either sign SHE can be either sign Definite Sign q ( v  B ) Definite Axis but Not Definite Sign - electron (Nagaosa et al.,) 13 14 Direct Spin Hall vs. Inverse Spin Hall effects (Optical) Observation of Spin Hall effect Charge current  pure spin accumulation ISHE in Pt detects pure spin current Direct Spin Hall Inverse Spin Hall Spin Current Charge Current   Spin Dependent Scattering Transverse Transverse Charge Imbalance Spin Imbalance (measured by side voltage) (measured by what ?) Optical observation SHE in semiconductors How to detect ? 15 15 Kato et. al. Science 306, 1910 (2004) 16 Spin Calortronics Spin Seebeck Effect Electronics Charge       V S T V S T spin spin        j s j j ( S S )( T )       K. Uchida et al., Nature, 455 , 778, (2008). J c =0 v J c =0 J s  0 up down Spin Heat Spin Caloritronics  T  T Spin Seebeck effect Metals, insulator, or semiconductors Ferromagnetic metals 17 18 18 How to detect J S ? 3

4/30/2014 Detection of Spin Current by Inverse Spin Hall Effect Long transmission of Spin Current Mystery 2: spin current (mm’s >> spin diffusion length) without dissipation ? ISHE in Pt ( spin – orbit scattering ) converts a spin current into an electromotive force E SHE Cold side Hot side 6 mm 4 mm 4 mm 8 mm FM metals FM insulators ?     E E D J y SHE ISHE S Sign change Proportional to  T Asymmetric in H Mystery 1: Asymmetric in H Spin-wave Conduction-electron spin current Sign change spin current K. Uchida et al., Nature, 455 , 778, (2008). 19 19 K. Uchida et al ., Nature 455 , 778 (2008); Nature Mater. 9 ,894 (2010); Kajiwara et al ., Nature 464, 262 (2010) 20 20 Spin Seebeck effect in broken FM semiconductors Transverse (  x T ) and Longitudinal (  z T ) Spin Seebeck Transmission of spin currents ? GaMnAs/GaAs SSE in FM Metal, Insulator SSE in FM Insulator V j s V j s Pt Pt m m  x T FM metal FM insulator FM Metal    t z     y sd E ( t ) G T T ( t ) tanh( ) intentional vertical  z T th m p SH  t 2 sd x Revision 2 : magnon-phonon drag through substrate intentional in-plane  x T Where is intrinsic SSE? Transverse configuration Longitudinal configuration (  z T ) (  x T ) Adachi et al., APL 97, 252506 (2010) C. M. Jaworski et al ., Nature Materials, 9 , 898 (2010) Jaworski et al., PRL 106, 186601 (2011) 21 21 22 22 Pt strip and in-plane temperature gradient  x T indicated Intrinsic Caloritronic effect (not substrate dominated) ? Pt Pt strip detects j S Intrinsic spin Seebeck effect ? Intrinsic spin-dependent thermal transport ? Pt In-plane  x T v v Pt H Uchida et al., Nature 455 , 778 (2008)  FM  T in-plane Uchida et al., Nat. Mater 9 , 894 (2010) Huang, Wang, Lee, Kwo, and Chien, “Intrinsic spin -dependent thermal transport, ” PRL 107 , 216604 (2011) . Jaworski et al., Nat. Mater 9 , 898 (2010) 23 24 24 4

4/30/2014 Create in-plane gradient  x T Consistent, Robust, but Strange  Vth(H,  ) Results  V  sin  v Py/Si Asymmetric in H H θ H=2000 Oe Hot Cold 1 2 3 4 5 v Higher T Lower T Heat flow H But this is physically impossible ! θ e.g., opposite signals at  = 90 ° and  = 270 ° . v H H 1 2 3 4 5 Py Py Py  x T  x T 25 26 26 Reversed  T, Same  V !! Out-of-plane  z T !!  x T  x T H=2000 Oe H=2000 Oe Sign change No sign change This is anomalous Nernst effect with perpendicular  z T !! m  z T   x T  T must be out -of-plane ! Transverse geometry, V y (Top view) 27 28 Only  z T !! Thin film on substrate: in-plane and out-of-plane gradient Anomalous Nernst effect: sensitive detector of  z  and  z T Uniform Heating from substrate  z T E ANE   z T  m  z T due to substrate m  x T FM substrate intentional in-plane  x T Same ANE sign and value everywhere In the transverse configuration (  x T) : where does  z T come from? 29 30 30 5

4/30/2014 What causes out-of-plane gradient  zT ? Electric Current vs. Heat Current Electrical Current Heat Current exclusively in-plane NOT exclusively in-plane Electrical Thermal V - T - V + T + Resistivity (Ωcm) > 1 >1 5x10 -6 10 -6 Thermal conductivity 125 56 30 80 (W/m-K) Substrate Substrate (10 4 x thicker) (10 4 x thicker) Electrically Insulating Not thermally Insulating Thermal conduction through substrate overwhelms! 31 32 Entanglement of ANE (due to  z T) and SSE (due to  x T) Removal of out-of-plane gradient (  z T) Anomalous Nernst Effect (ANE) Spin Seebeck Effect (SSE) sensitive detector of  z  and  z T Substrate-Free sample (  x T only ) v v j s Pt v Planar Nernst Effect Thermal AMR v m m (Transverse) (Longitudinal) z y m FM FM x  x T  x T FM  x T 10 4 x thicker !! E ANE   z T  m ( E SSE ) Pt  j s  m Both along y substrate V ANE and ( V SSE ) Pt additive , both are asymmetric in m (or H) In transverse configuration: SSE and ANE are entangled 33 33 34 S. Y. Huang et. al, Phys. Rev. Lett. 107, 216604 (2011) Intrinsic spin transport properties with in-plane  x T Spin Seebec effects with in-plane  x T and out-of-plane  z T SSE in FM Metal SSE in FM Insulator Substrate-free limit cos 2  M No strong evidence of SSE V j s V j s Longitudinal voltage: thermal AMR Pt Pt m m V th = V th  + ( V th  - V th|| ) cos 2  M Symmetric in H by using a substrate free sample  x T FM metal FM insulator sin2  M  FM Metal z y intentional vertical  z T x intentional in-plane  x T Transverse voltage: Planar Nernst effect PRB 83 , 224401 (2011), PRL 109 , 196602 Transverse configuration Longitudinal configuration sin2  M  (2012), PRL 111 , 187201 (2013), PRB 88 , SSE + ANE SSE 064410 (2013), PRB 88 214304 (2013), Necessary Signatures of FM film with in-plane  x T ! PRB 88 , 184425 (2013), and etc. 35 36 36 6