2 2 2 If x, y, and z are x y z + + = 1 principal - PowerPoint PPT Presentation

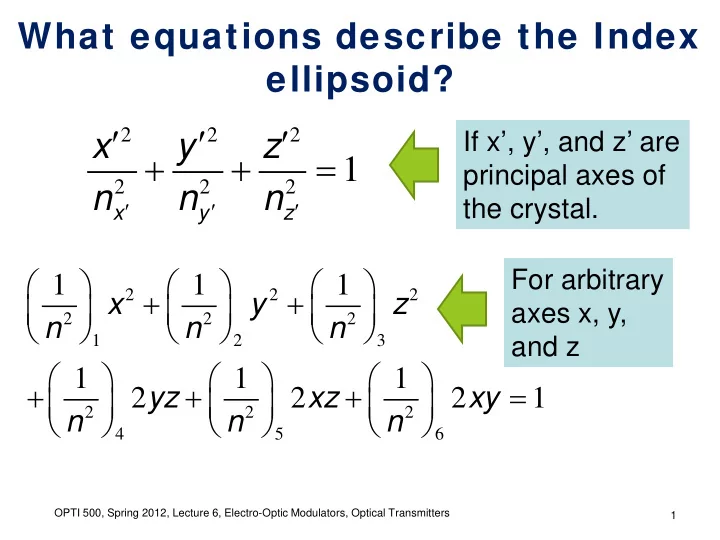
What equations describe the Index ellipsoid? ′ ′ ′ 2 2 2 If x’, y’, and z’ are x y z + + = 1 principal axes of 2 2 2 n n n ′ ′ ′ the crystal. x y z       For arbitrary 1 1 1 + + 2 2 2   x   y   z axes x, y,  2   2   2  n n n 1 2 3 and z       1 1 1 + + + = yz xz xy       2 2 2 1       2 2 2 n n n 4 5 6 OPTI 500, Spring 2012, Lecture 6, Electro-Optic Modulators, Optical Transmitters 1

How do w e use the index ellipsoid? k (0,0,n z ) z θ (0,n y ,0) y (n x ,0,0) x • The waves that can propagate as linearly polarized waves have polarization along the major and minor axes of the ellipse perpendicular to the wavevector k . OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 2

What is special about uni-axial crystals? k (0,0,n z ) z θ (0,n y ,0) y (n x ,0,0) x • n x = n y = n o • There is always a linearly polarized wave, called the ordinary wave, that “sees” a refractive index n o , regardless of the direction of propagation. OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 3

What changes in the modulator to cause constructive interference to become destructive? z Optical Electrode Waveguide Optical y x Input Metal Electrode Modulated Optical Output Electrode Radiation Modes • The magnitude and/or direction of the applied electric fields. OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 4

Are there any important corrections to the notes? 1 1 ( ) ( ) = − = − 2 3 n E n n r E n E n n r E 0 z 0 o 13 z o z o o 13 z 2 2 1 ( ) 1 ( ) = − 2 = − n E n n r E 3 n E n n r E e z e o 33 z 2 e z e e 33 z 2 • A large refractive index enhances the electro-optic effect. OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 5

How did w e get the equations for LiNbO 3 ?     1 ∆     2  n   1     1 ∆     −  2    n 0 r r   2 22 13           0 r r 1 1 1   ∆ ∆ = ∆ =     22 13   0     r E   13 z  2   2   2  n   0 0 r   n n = ⋅ ⇒ 3 33 1 2   0         0 r 0 1 1    ∆    ∆ = 51   E    r E   33 z z   2    2  r 0 0 n n 4  51  3   −    0 0  r 1   ∆ 22     2  n 5     1   ∆     2   n  6 OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 6

How did w e get the equations for LiNbO 3 ?   1 ∆ =   r E 33 z  2  n 3 taking a derivative we find:   1 1 1 ∆ = ∆ = − ∆ 2   n e  2  2 3 n n n 3 e e 1 1 ( ) ⇒ ∆ = − = − 3 3 n n r E and n E n n r E e e 33 z e z e e 33 z 2 2 OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 7

How does LiNbO 3 compare w ith other electro-optic materials? From “Optoelectronics” by Pollock OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 8

Can w e have a quadratic electro- optic effect? ( ) = + + 2 n E n a E a E    0 1 2 Linear Kerr Refractive − Effect Electro Index optic Effect ( Pockels Effect ) OPTI 500, Spring 2012, Lecture 6, Electo-Optic Modulators, Optical Transmitters 9