1
play

1 can define x = x . 1 By adding an element 1 to the real - PowerPoint PPT Presentation

Feb 27, 2024 •245 likes •323 views

Complex Numbers Idea: In the real numbers, negative numbers do not have square roots. However, if one defines an "imaginary" number 1 that has the 2 = 1 , then for any non-negative real number x , we property that 1 can

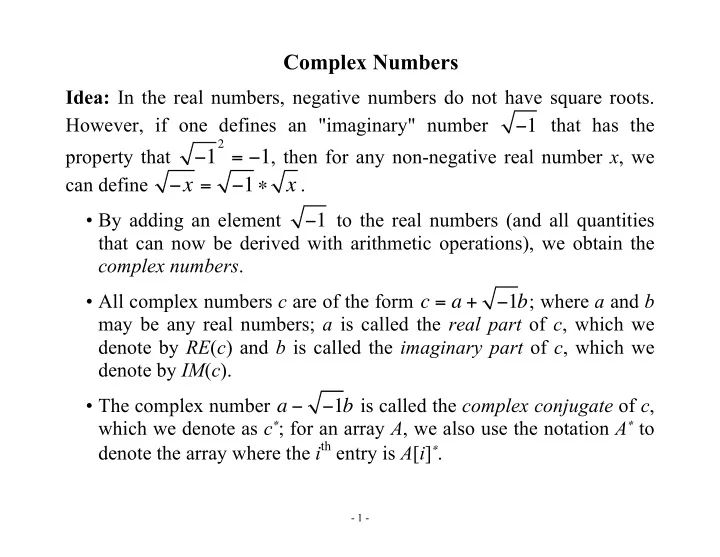
  1. Complex Numbers Idea: In the real numbers, negative numbers do not have square roots. However, if one defines an "imaginary" number − 1 that has the 2 = − 1 , then for any non-negative real number x , we property that − 1 can define − x = x . − 1 ∗ • By adding an element − 1 to the real numbers (and all quantities that can now be derived with arithmetic operations), we obtain the complex numbers . • All complex numbers c are of the form c = a + − 1 b ; where a and b may be any real numbers; a is called the real part of c , which we denote by RE ( c ) and b is called the imaginary part of c , which we denote by IM ( c ). • The complex number a − − 1 b is called the complex conjugate of c , which we denote as c ∗ ; for an array A , we also use the notation A ∗ to denote the array where the i th entry is A [ i ] ∗ . - 1 -

  2. Note: A complex number can be thought of as a way to store two real numbers at once (the real and imaginary part). For example, with an audio signal that is represented in the frequency domain, the real part can store the magnitude and the imaginary part can store the phase shift. - 2 -

  3. Polar Coordinates Instead of representing a complex number c = a + − 1 b in Cartesian coordinates by the two numbers ( a,b ), we can represent c in polar coordinates by the two numbers ( x,d ), where x is its angle from the real axis d is its distance from the origin: - 3 -

  4. Complex Exponentials (Recall that e denotes the natural logarithm base e= 2 . 71 .. .) − 1 x = cos( x ) + Theorem: For any real number x , e − 1sin( x ) . Proof: The Taylor Series for a continuously differentiable function f ( x ) is f (0) x 2 (0) x 3 (0) x 4 f f ′′ ′′′ ′′′′ + L f ( x ) = f (0) + f (0) x + ′ + 2! 3! 4! Since the derivative of e x is e x , the derivative of sin( x ) is cos( x ), and the derivative of cos( x ) is –sin( x ), it follows that − 1 x − x 2 − 1 x 3 + x 4 − 1 x 5 e − 1 x = 1 + − L 2 − 4! + 3! 5! cos( x ) = 1 − x 2 2 + x 4 4! − x 6 6! + x 8 8! − L sin( x ) = x − x 3 3! + x 5 5! − x 7 7! + x 9 9! − L - 4 -

  5. (complex exponential proof continued) and hence: − 1 x − x 2 − 1 x 3 + x 4 − 1 x 5 e − 1 x = 1 + − L 2 − 4! + 3! 5! = 1 − x 2 2 + x 4 − 1 x 3 − 1 x 5     4! − L − 1 x −  + + −    3! 5!     = 1 − x 2 2 + x 4 − 1 x − x 3 3! + x 5     4! − L  + 5! −        = cos( x ) + − 1sin( x ) - 5 -

  6. (complex exponentials continued) Intuition: • e − 1 x is a point on the unit diameter complex circle centered at the origin of the complex plane at angle x with real component cos( x ) and imaginary component sin( x ) . Idea: • Complex exponentials are a nice way to represent a point on the complex plane (to multiply two complex exponentials, we can just add the exponents). • Complex numbers are a nice way to store magnitude and phase information for points in the frequency domain of a digitally sampled analog signal. - 6 -

Recommend

More recommend