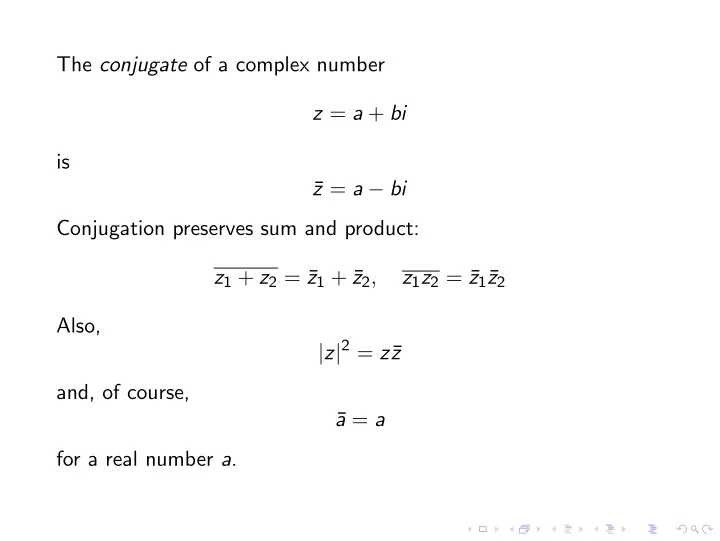
The conjugate of a complex number z = a + bi is z = a bi - PowerPoint PPT Presentation

The conjugate of a complex number z = a + bi is z = a − bi ¯ Conjugation preserves sum and product: z 1 + z 2 = ¯ z 1 + ¯ z 1 z 2 = ¯ z 1 ¯ z 2 , z 2 Also, | z | 2 = z ¯ z and, of course, a = a ¯ for a real number a .

Consequently, if p ( x ) = a n x n + a n − 1 x n − 1 + · · · + a 1 x + a 0 is a polynomial with real coefficients a 0 , . . . , a n ∈ R , then p ( z ) = p (¯ z ) for a complex number z . Thus, if α + β i is a complex root of p ( x ), then so is α − β i . I.e., properly complex roots of a real polynomial come in conjugate pairs α ± β i .

Examples: (a) The roots of x 2 + 1 are ± i . (b) The roots of x 3 + x 2 − 2 are 1 and − 1 ± i . (c) The roots of x 2 + x + 1 are 1 � 2 ( − 1 ± i 3). (d) The roots of x 4 + 2 x 2 + 1 are ± i , each with multiplicity 2.

To handle complex roots of the auxiliary polynomial, we need the complex exponential ∞ z n e z = � n ! n =0 This power series is absolutely convergent of all z ∈ C , and hence convergent. It behaves basically like the usual exponential function, i.e., e z + w = e z e w For an imaginary number ix , we get ∞ ∞ ( ix ) n i n x n e ix = � � = n ! n ! n =0 n =0 ∞ ∞ ( − 1) k x 2 k ( − 1) k x 2 k +1 � � = + i (2 k )! (2 k + 1)! k =0 k =0 = cos( x ) + i sin( x )

and in general, e x + iy = e x e iy = e x � � cos( y ) + i sin( y )

For a complex number m = α + β i , we look at the function e mx = e ( α + β i ) x = e α x � � cos( β x ) + i sin( β x ) and find that ( e mx ) ′ = me mx So if m is a complex root of an auxiliary polynomial, then e mx is a solution of sorts to the differential equation, just not of the kind we would prefer.

But as ¯ m = α − β i is also a root, mx = e ( α − β i ) x = e α x � e ¯ � cos( β x ) − i sin( β x ) is also a solution to the differential equation, and if we combine them properly, all references to complex numbers disappear: � �� e α x cos( β x ) = 1 e α x � � + e α x � cos( β x )+ i sin( β x ) cos( β x ) − i sin( β x ) 2 and � �� e α x sin( β x ) = 1 e α x � − e α x � � cos( β x )+ i sin( β x ) cos( β x ) − i sin( β x ) 2 i

All in all, we get that a pair α ± β i of properly complex roots to the auxiliary polynomial gives us a pair e α x cos( β x ) , e α x sin( β x ) of solutions to the corresponding differential equation.

Example: For y ′′ + y = 0 we have the auxiliary polynomial m 2 + 1 with complex roots ± i . Thus, we get solutions e 0 x cos( x ) = cos( x ) e 0 x sin( x ) = sin( x ) and and a general solution y = a cos( x ) + b sin( x )

Example: We consider the IVP given by y ′′ − 2 y ′ + 2 y = 0 , y ′ (0) = 0 y (0) = 1 , The auxiliary polynomial for the differential equation is m 2 − 2 m + 2 = ( m − 1) 2 + 1 with complex roots 1 ± i , so we get a fundamental set e x cos( x ) , e x sin( x ) and a general solution y = e x � � a cos( x ) + b sin( x )

The first of the initial conditions now is y (0) = a = 1 And since y ′ = e x � � ( a + b ) cos( x ) + ( b − a ) sin( x ) the second condition is y ′ (0) = a + b = 0 so b = − 1 and the solution to the IVP is y = e x � � cos( x ) − sin( x )

Let us verify that y 1 = e x cos( x ) is a solution: We have 1 = e x cos( x ) − e x sin( x ) = e x � y ′ � cos( x ) − sin( x ) and = − 2 e x sin( x ) y ′′ 1 = e x � + e x � � � cos( x ) − sin( x ) − sin( x ) − cos( x ) so y ′′ 1 − 2 y ′ 1 + 2 y 1 = − 2 e x sin( x ) − 2 e x � + 2 e x cos( x ) = 0 � cos( x ) − sin( x )

Example: Consider the differential equation y ′′′ − 5 y ′′ + 9 y ′ − 5 = 0 The auxiliary polynomial is m 3 − 5 m 2 + 9 m − 5 = ( m − 1)( m 2 − 4 m + 5) which has roots 4 ± √− 4 = 4 ± 2 i 1 and = 2 ± i 2 2 so we get a fundamental system e 2 x cos( x ) , e 2 x sin( x ) e x , and a general solution y = ae x + be 2 x cos( x ) + ce 2 x sin( x )

Example: We look at y (4) + 6 y ′′ + 25 y = 0 The auxiliary polynomial is m 4 + 6 m 2 + 25 = ( m 2 + 2 x + 5)( m 2 − 2 x + 5) with roots ± 2 ± √− 16 = ± 2 ± 4 i = ± 1 ± 2 i 2 2 so we get a fundamental system e x cos(2 x ) , e x sin(2 x ) , e − x cos(2 x ) , e − x sin(2 x ) and a general solution y = ae x cos(2 x ) + be x sin(2 x ) + ce − x cos(2 x ) + de − x sin(2 x )

That leaves only multiple complex roots: x n + a n − 1 x n − 1 + · · · + a 1 x + a 0 = ( x − α ) 2 + β 2 � k g ( x ) , � k > 1 Here, we get solutions x i e ( α ± β i ) x , 0 ≤ i < k which we rearrange to get x i e α x cos( β x ) , x i e α x sin( β x ) , 0 ≤ i < k

Example: We look at y (4) + 2 y ′′ + y = 0 The auxiliary equation is m 4 + 2 m 2 + 1 = ( m 2 + 1) 2 = 0 so the solutions are ± i , both with multiplicity 2. Thus, a fundamental set is cos( x ) , x cos( x ) , sin( x ) , x sin( x ) and the general solution is y = a cos( x ) + bx cos( x ) + c sin( x ) + dx sin( x )

Initial conditions at x 0 = 0 are y (0) = y 0 = a y ′ (0) = y 1 = b + c y ′′ (0) = y 2 = − a + 2 d y ′′′ (0) = y 3 = − 3 b − c showing that the functions are linearly independent.