1
play

1 Prof. S. Ben-Yaakov , DC-DC Converters [10- 4] Closed Loop V o - PDF document

Feb 09, 2024 •685 likes •906 views

Prof. S. Ben-Yaakov , DC-DC Converters [10- 1] Power Converters Control Technique 10.1 The Dynamic Problem 10.2 Control 10.2.1 Modulator 10.2.2 Oscillator 10.2.3 Isolation 10.3 Design of feedback system 10.4

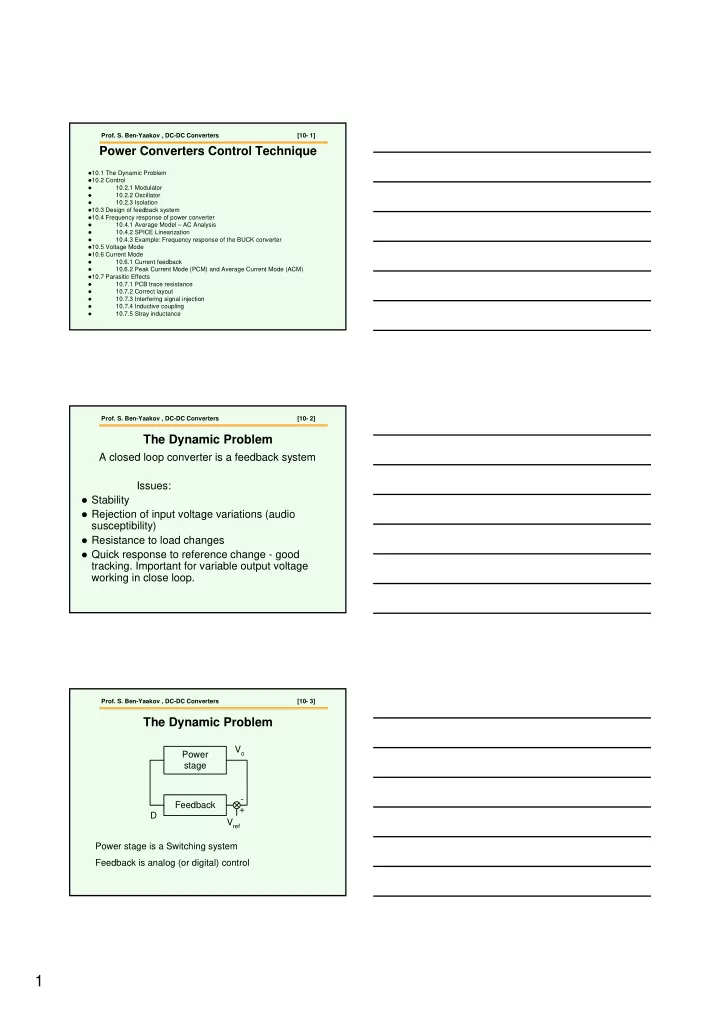
  1. Prof. S. Ben-Yaakov , DC-DC Converters [10- 1] Power Converters Control Technique � 10.1 The Dynamic Problem � 10.2 Control � 10.2.1 Modulator � 10.2.2 Oscillator � 10.2.3 Isolation � 10.3 Design of feedback system � 10.4 Frequency response of power converter � 10.4.1 Average Model – AC Analysis � 10.4.2 SPICE Linearization � 10.4.3 Example: Frequency response of the BUCK converter � 10.5 Voltage Mode � 10.6 Current Mode � 10.6.1 Current feedback � 10.6.2 Peak Current Mode (PCM) and Average Current Mode (ACM) � 10.7 Parasitic Effects � 10.7.1 PCB trace resistance � 10.7.2 Correct layout � 10.7.3 Interfering signal injection � 10.7.4 Inductive coupling � 10.7.5 Stray inductance Prof. S. Ben-Yaakov , DC-DC Converters [10- 2] The Dynamic Problem A closed loop converter is a feedback system Issues: � Stability � Rejection of input voltage variations (audio susceptibility) � Resistance to load changes � Quick response to reference change - good tracking. Important for variable output voltage working in close loop. Prof. S. Ben-Yaakov , DC-DC Converters [10- 3] The Dynamic Problem V o Power stage - Feedback + D V ref Power stage is a Switching system Feedback is analog (or digital) control 1

  2. Prof. S. Ben-Yaakov , DC-DC Converters [10- 4] Closed Loop V o Power v o stage R 1 - MOD + d D v e V e V ref R 2 β β e m v ( ) − o f Ana log Function v e Feedback factor v e (small signal) into d (small signal) Prof. S. Ben-Yaakov , DC-DC Converters [10- 5] The Concept of d V e t D Zoom t d D t d is the AC component of D Prof. S. Ben-Yaakov , DC-DC Converters [10- 6] Control V in ( power ) Duty cycle V O ( power ) Power stage C O R O Feedback The power conversion system k V O V e PWM Driver modulator error amp V ref 2

  3. Prof. S. Ben-Yaakov , DC-DC Converters [10- 7] Modulator comp ( ) V e − + V V t p v = + V V - t v T s V p ( ) − V e V V t = = p v on + V V V V v t e v Oscillator T s ( ) D − t V V = = on e v D 1 on − T V V s p v 0 V e V v V p Practical D on max ≈ 0.8 ÷ 0.9 Prof. S. Ben-Yaakov , DC-DC Converters [10- 8] Oscillator Prof. S. Ben-Yaakov , DC-DC Converters [10- 9] Complete controller - Voltage Mode (VM) � This controller does not include an error amplifier 3

  4. Prof. S. Ben-Yaakov , DC-DC Converters [10- 10] Primary to secondary isolation The problem : D R O Converter Filter V O Isolation barrier Prof. S. Ben-Yaakov , DC-DC Converters [10- 11] Feedback Alternatives P in A C V Power V o Power o stage stage isolation - - feedback feedback Gain + + V isolation ref V ref D B D V V Power D Power o o stage stage D + feedback - Gain - feedback + + isolation V isolation ref V ref Prof. S. Ben-Yaakov , DC-DC Converters [10- 12] Output Voltage Sampler ⎛ ⎞ β V A ⎜ o ⎟ = 1 1 V o ⎜ ⎟ + β ⎝ V ⎠ 1 A K A 1 ref 1 1 R K R β > A K 1 1 1 R β 1 V ref ⎛ ⎞ V 1 ⎜ o ⎟ = ⎜ ⎟ ⎝ ⎠ V K ref R 4

  5. Prof. S. Ben-Yaakov , DC-DC Converters [10- 13] LoopGain V ref β A 1 ( f ) ( f ) 1 K R Stability and dynamic response depend on Loop Gain (LG) ( ) ( ) = R β LG K f A f 1 1 General representation ( ) ( ) = β LG A f f Prof. S. Ben-Yaakov , DC-DC Converters [10- 14] Bode Plot db ( ) ( ) β |LG| f A f f φ o +180 In negative feedback f 0 o − φ = o 180 ( 180 ) systems At f → 0 Prof. S. Ben-Yaakov , DC-DC Converters [10- 15] Nyquist Criterion A ( s ) = A CL + 1 LG ( s ) � The system is unstable if {1+LG(s)} has roots in the right half of the complex plane. � Nyquist criterium is a test for location of {1+LG(s)} roots. � Nyquist criterium is normally translated into the Bode plane (frequency domain) 5

  6. Prof. S. Ben-Yaakov , DC-DC Converters [10- 16] Bode Presentation db β A β A = 1 f o φ 180 o already substracted f 0 ϕ m -180 ϕ = ϕ − − = ϕ + o o ( 180 ) 180 β = β = m | A | 1 | A | 1 Prof. S. Ben-Yaakov , DC-DC Converters [10- 17] The design problem Given A(f) Find β (f) ( ) 20 log A | β A | f ⎛ ⎞ 1 ( ) ⎛ ⎞ = ⎜ ⎟ 1 20 log A 20 log ⎜ ⎟ 20 log β ⎝ ⎠ β ⎝ ⎠ β A = 1 ⎛ ⎞ 1 ( ) ( ) − ⎜ ⎟ = β 20 log A 20 log 20 log A β ⎝ ⎠ Prof. S. Ben-Yaakov , DC-DC Converters [10- 18] LG=1 A db 1 | β db A | β f | β A | β A = 1 β A db = 0 6

  7. Prof. S. Ben-Yaakov , DC-DC Converters [10- 19] Rate of Closure β A − db 20 db dec − 20 db If rate of closure dec − db 40 system is stable dec + db 20 dec f rate of − db 20 dec closure 0 db dec − db 20 dec A s db u − db 40 s + dec db 20 u 0 db dec dec s − − 20 db 60 db dec dec f s − db 1 40 dec ( ) db β f Prof. S. Ben-Yaakov , DC-DC Converters [10- 20] Bandwidth ϕ = o 90 ϕ = o m 45 m ϕ = o 90 m ϕ = o 45 m ϕ = o 90 1 m ϕ = o β 45 m |A| f Close loop Bandwidth | β A | t as large as f shift possibe DC error Not important as low as possible Prof. S. Ben-Yaakov , DC-DC Converters [10- 21] Phase Margin Effects ϕ > o Choose 40 Exitation m Overshoot t M f Overshoot ϕ m o 60 M ϕ m 7

  8. Prof. S. Ben-Yaakov , DC-DC Converters [10- 22] DC LG ϕ = o 90 ϕ = o m 45 m ϕ = o 90 m ϕ = o 45 m ϕ = o 90 1 m ϕ = o β 45 m |A| f Close loop Bandwidth | β A | t as large as f shift possibe DC error Not important as low as possible Prof. S. Ben-Yaakov , DC-DC Converters [10- 23] Lag Lead 1 β f β − 20 db dec A 0 f 2 f f 1 A 2 Prof. S. Ben-Yaakov , DC-DC Converters [10- 24] Average Model – AC Analysis L S V o D R V C o in o = ⋅ L E V D V in in on o G = ⋅ C G I D b I o b L on V R L − → in E V V o E in o L in Polarity: (voltage and current sources) selected by inspection 8

  9. Prof. S. Ben-Yaakov , DC-DC Converters [10- 25] Linearization out V(in) I(3) = ∗ V ( out ) V ( in ) I ( 3 ) R ∂ ∂ ( V ( out )) ( V ( out )) = + d ( V ( out )) v ( in ) i ( 3 ) ∂ ∂ ( V ( in )) ( I ( 3 )) ∆ ∆ V ( out ) V ( out ) = + V ( out ) v ( in ) i ( 3 ) ∆ ∆ V ( in ) I ( 3 ) Prof. S. Ben-Yaakov , DC-DC Converters [10- 26] SPICE Linearization (AC Analysis) out out ⎡ ∆ ⎤ F ⋅ V(in) I(3) i ( 3 ) ⎢ ⎥ ∆ ⎣ ⎦ I ( 3 ) R o R ⎡ ∆ ⎤ F ⋅ V ( in ) ⎢ ⎥ ∆ ⎣ V ( in ) ⎦ o ∆ ∆ F = F = I ( 3 ) V ( in ) ∆ 0 ∆ 0 V ( in ) I ( 3 ) Prof. S. Ben-Yaakov , DC-DC Converters [10- 27] Buck linearization L in out in = E V D in C b = I o R G I D V in L o E L G in b ⎡ ∆ ⎤ E in ⎢ ⎥ I ∆ ⎣ ⎦ D L L in o out 0 ⋅ V ( in ) v ( d ) d 0 ⋅ V in R o V ( d ) i ( L ) C o VAC R 0 ⋅ 0 ⋅ V ( d ) v ( in ) I ( L ) v ( d ) V D ⎡ ⎤ ∆ G ∆ ⎡ ⎤ G b ⎡ ∆ ⎤ ⎢ ⎥ E b ∆ ⎢ ⎥ ⎣ I ⎦ ⎢ in ⎥ ⎣ ∆ ⎦ D L o ∆ ⎣ V ⎦ o in o 9

  10. Prof. S. Ben-Yaakov , DC-DC Converters [10- 28] Example: Buck Average Model Simulations NODESET= 5 V(Don)*I(Lout)/(V(Don)+V(Doff)) + b Rinductor a Vin_pulse Cout {Rinductor} GVALUE GVALUE + - {Cout} Ga c EL Lout Dbreak RLoad GVALUE Vin {Lout} IN- OUT- IN- OUT- IN+ OUT+ Resr + D1 OUT+ IN+ OUT+ IN+ IN- OUT- OUT+ IN+ {RLoad} {Vin} OUT- IN- {Resr} - EVALUE Gc Gb I(Lout) V(Don)*V(a,b)+V(Doff)*V(a,c) 0 V(Doff)*I(Lout)/(V(Don)+V(Doff)) 1V PARAMETERS: PARAMETERS: Don Doff EDoff VIN = 10v LOUT = 75u - + VDON = 0.5 COUT = 220u + Vexcitation IN+ OUT+ VDon RLOAD = 10 IN- OUT- - {VDon} etable PARAMETERS: PARAMETERS: RESR = 0.07 FS = 100k 0 RINDUCTOR = 0.1 TS = {1/fs} min(2*abs(I(Lout))*Lout/(Ts*(vin-V(a))*V(Don))-V(Don),1-V(Don)) Prof. S. Ben-Yaakov , DC-DC Converters [10- 29] Example: Buck DC Sweep Analysis (CCM/DCM) D on =0.5 V ( out ) 7 00m V ( in ) 6 00m border line 5 00m R LOAD DCM 4 00m V( a ) / V( B) D 60 0mV off 40 0mV CCM R LOAD SEL>> 15 0mV 10 20 3 0 40 50 60 70 80 90 100 V( Dof f ) RLoa d Prof. S. Ben-Yaakov , DC-DC Converters [10- 30] Example: Buck AC Analysis (CCM/DCM) ⎛ ⎞ v ( out ) ⎜ ⎟ 40 dB CCM : R load =10 ohm ⎝ ⎠ v ( d ) 20 0 - 20 DCM : R load =100 ohm - 40 1. 0Hz 10Hz 1 00Hz 1. 0KHz 10KHz 100KHz DB( V( a ) ) Fr e que nc y 10

Recommend

More recommend