1 Errata group 1: Complex integration [correction] z = x + iy dz = - PowerPoint PPT Presentation

Errata group 1: Complex integration ✗ 1

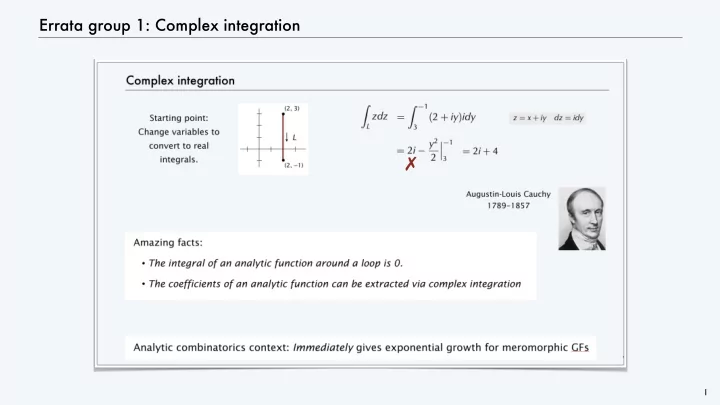
Errata group 1: Complex integration [correction] z = x + iy dz = idy (2, 3) Z − 1 2 iy − y 2 Z − 1 �� � zdz = (2 + iy ) idy = 3 = − 8 i + 4 � 2 � L ✓ L 3 (2, − 1) Much better approach. Complex integration works exactly as expected. Theorem. (cf. Stein, Theorem 3.2 ) If a continuous function f has an Z antiderivative F and 𝛿 is a curve from w 1 to w 2 then f ( z ) dz = F ( w 2 ) − F ( w 1 ) γ zdz = z 2 = 1 Z 2 − i � (2 − i ) 2 − (2 + 3 i ) 2 � � = − 8 i + 4 � 2 2 � 2+3 i L 2

Errata group 1: Complex integration (continued) ✗ ✗ ✗ ✗ ✗ 3

Errata group 1: Complex integration [improved] Ex 1. Integrate f ( z ) = z on a rectangle a b L 1 b ( − 4, 3) (2, 3) zdz = z 2 b 2 2 − a 2 � Z � R = � 2 2 � L 1 a L 4 L 2 c zdz = z 2 c 2 2 − b 2 � Z � = � 2 2 ( − 4, − 1) (2, − 1) � L 2 b L 3 d c d zdz = z 2 d 2 2 − c 2 � Z � = � 2 2 � L 3 c a zdz = z 2 a 2 2 − d 2 � Z � = � 2 2 � L 4 d Z Z zdz = zdz = 0 R L 1 + L 2 + L 3 + L 4 4

A new question was posted by Eric Neyman (April 2017). Residues Calculated Incorrectly I believe that every residue in the lectures is calculated incorrectly (off by a sign). The formula given for the residue is $$-\frac{f(\alpha)} {g'(\alpha)}$$, but this is incorrect. It should be $$\frac{f(\alpha)} {g'(\alpha)}$$. The error seems to be traceable back to Slide 59 of the Poles lecture slides, where instead of writing $$\frac{h_{-1}}{z - \alpha} $$, $$\frac{h_{-1}}{\alpha - z}$$ was written. But in fact the way residues are defined in Slide 51 (as in $$\frac{h_{-1}}{z - z_0}$$). I think that the reason that the asymptotics in the examples are right is because the constant $$c$$, which is claimed to be $$\frac{h_{-1}}{\alpha} $$, really should be $$(-1)^M \frac{h_{-1}}{\alpha}$$, and that these two mistakes cancel out.

Errata group 2: Residues ✗ should be z- 𝜷 everywhere leads to numerous sign errors in later slides similar error on p. 256 in book 6

Bottom line 1 ✗ ✗ should be 𝜷 1 -z should be -n-r f ( z ) rational with a single dominant pole α β N N M − 1 z → α ( α − z ) M f ( z ) [ z N ] f ( z ) = ( M − 1)! α M lim where β = 1 / α and M is the multiplicity of α 7

Bottom line 2 h ( z ) meromorphic with a single dominant pole α [ z N ] h ( z ) = ( − 1) M Mf ( α ) α M g ( M ) ( α ) β N N M − 1 where β = 1 / α and M is the multiplicity of α 8