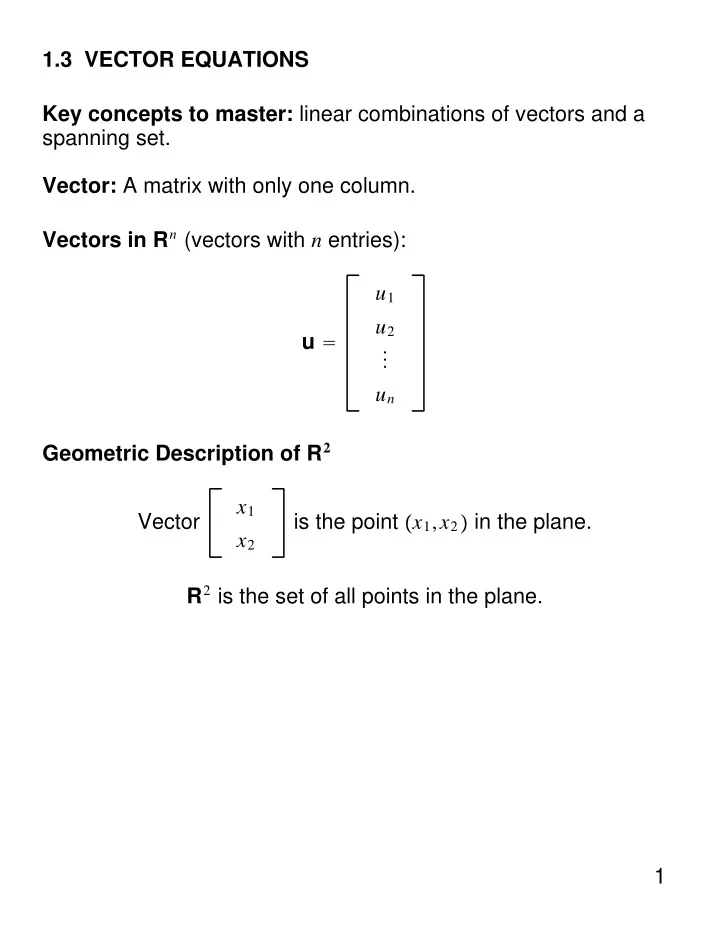
1.3 VECTOR EQUATIONS Key concepts to master: linear combinations of - PDF document

1.3 VECTOR EQUATIONS Key concepts to master: linear combinations of vectors and a spanning set. Vector: A matrix with only one column. Vectors in R n (vectors with n entries): u 1 u 2 u = ⋮ u n Geometric Description of R 2 x 1 Vector is the point  x 1 , x 2  in the plane. x 2 R 2 is the set of all points in the plane. 1

Parallelogram rule for addition of two vectors: If u and v in R 2 are represented as points in the plane, then u + v corresponds to the fourth vertex of the parallelogram 0 whose other vertices are 0 , u and v . (Note that 0 = .) 0 1 2 EXAMPLE: Let u = and v = . Graphs of u , v 3 1 and u + v are given below: x 2 4 3 2 1 4 x 1 1 2 3 Illustration of the Parallelogram Rule 2

1 − 3 EXAMPLE: Let u = . Express u , 2 u , and 2 u on a 2 graph. x 2 4 3 2 1 x 1 − 2 − 1 1 2 − 1 − 2 − 3 3

Linear Combinations DEFINITION Given vectors v 1 , v 2 , … , v p in R n and given scalars c 1 , c 2 , … , c p , the vector y defined by y = c 1 v 1 + c 2 v 2 + ⋯ + c p v p is called a linear combination of v 1 , v 2 , … , v p using weights c 1 , c 2 , … , c p . Examples of linear combinations of v 1 and v 2 : 1 3 v 1 + 2 v 2 , 3 v 1 , v 1 − 2 v 2 , 0 4

2 − 2 EXAMPLE: Let v 1 = and v 2 = . Express 1 2 each of the following as a linear combination of v 1 and v 2 : 0 − 4 6 7 a = , b = , c = , d = 3 1 6 − 4 x 2 8 6 4 2 x 1 − 8 − 6 − 4 − 2 2 4 6 8 − 2 − 4 − 6 − 8 5

1 4 3 EXAMPLE: Let a 1 = , a 2 = , a 3 = , 0 2 6 3 14 10 − 1 and b = . 8 − 5 Determine if b is a linear combination of a 1 , a 2 , and a 3 . Solution: Vector b is a linear combination of a 1 , a 2 , and a 3 if can we find weights x 1 , x 2 , x 3 such that x 1 a 1 + x 2 a 2 + x 3 a 3 = b . Vector Equation (fill-in): Corresponding System: 4 x 2 3 x 3 = − 1 x 1 + + 2 x 2 6 x 3 8 + = 3 x 1 14 x 2 + 10 x 3 = − 5 + 6

Corresponding Augmented Matrix: 1 4 3 − 1 1 0 0 1 x 1 = ___ 0 2 6 8 0 1 0 − 2 x 2 = ___   3 14 10 − 5 0 0 1 2 x 3 = ___ Review of the last example: a 1 , a 2 , a 3 and b are columns of the augmented matrix 1 4 3 − 1 0 2 6 8 3 14 10 − 5 ↑ ↑ ↑ ↑ a 1 a 2 a 3 b Solution to x 1 a 1 + x 2 a 2 + x 3 a 3 = b is found by solving the linear system whose augmented matrix is . a 1 a 2 a 3 b 7

A vector equation x 1 a 1 + x 2 a 2 + ⋯ + x n a n = b has the same solution set as the linear system whose augmented matrix is . a 1 a 2 ⋯ a n b In particular, b can be generated by a linear combination of a 1 , a 2 , … , a n if and only if there is a solution to the linear system corresponding to the augmented matrix. 8

The Span of a Set of Vectors 3 0 EXAMPLE: Let v = . Label the origin 4 0 5 0 together with v , 2 v and 1.5 v on the graph below. x 3 x 2 x 1 v , 2 v and 1.5 v all lie on the same line. Span  v  is the set of all vectors of the form c v . Here, Span  v  = a line through the origin. 9

EXAMPLE: Label u , v , u + v and 3 u + 4 v on the graph below. x 3 x 2 x 1 u , v , u + v and 3 u + 4 v all lie in the same plane. Span  u , v  is the set of all vectors of the form x 1 u + x 2 v . Here, Span  u , v  = a plane through the origin. 10

Definition Suppose v 1 , v 2 , … , v p are in R n ; then Span  v 1 , v 2 , … , v p  = set of all linear combinations of v 1 , v 2 , … , v p . Stated another way: Span  v 1 , v 2 , … , v p  is the collection of all vectors that can be written as x 1 v 1 + x 2 v 2 + ⋯ + x p v p where x 1 , x 2 , … , x p are scalars. 2 4 EXAMPLE: Let v 1 = and v 2 = . 1 2 (a) Find a vector in Span  v 1 , v 2  . (b) Describe Span  v 1 , v 2  geometrically. 11

Spanning Sets in R 3 x 3 v 2 v 1 x 2 x 1 v 2 is a multiple of v 1 Span  v 1 , v 2  = Span  v 1  = Span  v 2  (line through the origin) 12

x 3 x 2 x 1 v 2 v 1 v 2 is not a multiple of v 1 Span  v 1 , v 2  = plane through the origin 4 6 EXAMPLE: Let v 1 = and v 2 = . Is 2 3 2 3 Span  v 1 , v 2  a line or a plane? 13

1 2 8 EXAMPLE: Let A = and b = . Is b in 3 1 3 0 5 17 the plane spanned by the columns of A ? Solution: 1 2 8 b = A = 3 1 3 0 5 17 Do x 1 and x 2 exist so that Corresponding augmented matrix: 1 2 8 1 2 8 1 2 8 3 1 3 0 − 5 − 21 0 − 5 − 21   0 5 17 0 5 17 0 0 − 4 So b is not in the plane spanned by the columns of A 14