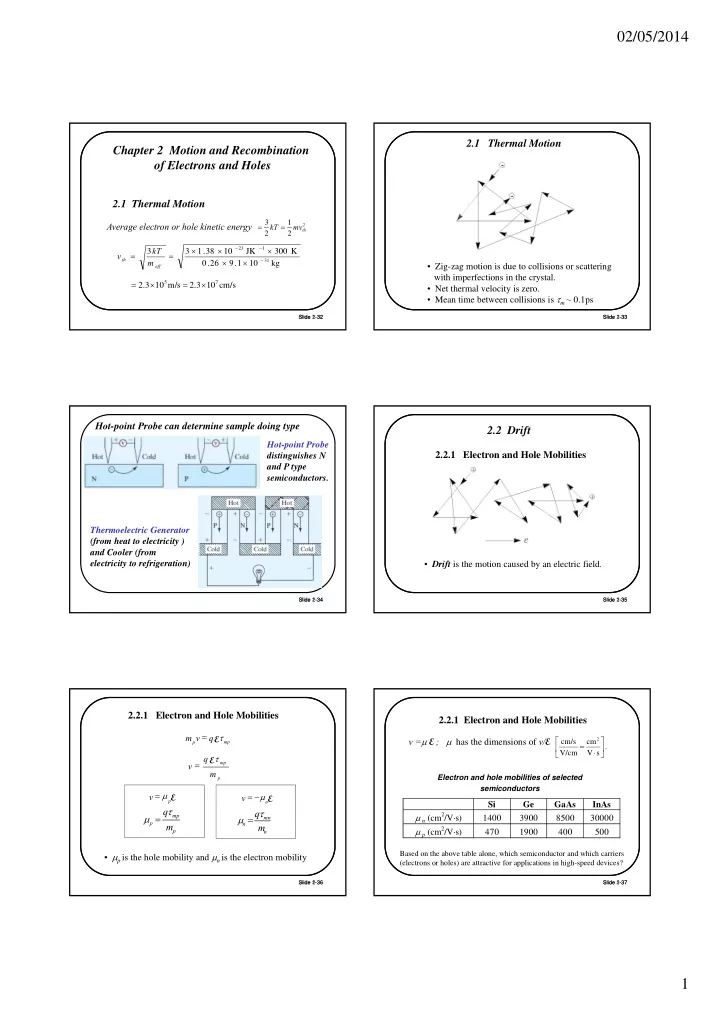
02/05/2014 2.1 Thermal Motion Chapter 2 Motion and Recombination - PDF document

02/05/2014 2.1 Thermal Motion Chapter 2 Motion and Recombination of Electrons and Holes 2.1 Thermal Motion 3 1 Average electron or hole kinetic energy  kT  2 mv 2 2 th    23  1  3 kT 3 1 . 38 10 JK 300 K   v th    31 m 0 . 26 9 . 1 10 kg • Zig-zag motion is due to collisions or scattering eff with imperfections in the crystal.   5   7 2 . 3 10 m/s 2 . 3 10 cm/s • Net thermal velocity is zero. • Mean time between collisions is  m ~ 0.1ps Slide 2-32 Slide 1-32 Slide 2-33 Slide 1-33 Hot-point Probe can determine sample doing type 2.2 Drift Hot-point Probe 2.2.1 Electron and Hole Mobilities distinguishes N and P type semiconductors. Thermoelectric Generator (from heat to electricity ) and Cooler (from • Drift is the motion caused by an electric field . electricity to refrigeration) Slide 1-34 Slide 2-34 Slide 1-35 Slide 2-35 2.2.1 Electron and Hole Mobilities 2.2.1 Electron and Hole Mobilities   m v q E v =  E ;  has the dimensions of v/ E   cm/s cm 2 mp p  .    V/cm V s   q  E  mp v m Electron and hole mobilities of selected p semiconductors      v E v E p n Si Ge GaAs InAs q  q   n (cm 2 /V·s)   mp 1400 3900 8500 30000   mn p m n m  p (cm 2 /V·s) p 470 1900 400 500 n Based on the above table alone, which semiconductor and which carriers •  p is the hole mobility and  n is the electron mobility (electrons or holes) are attractive for applications in high-speed devices? Slide 1-36 Slide 2-36 Slide 1-37 Slide 2-37 1

02/05/2014 Drift Velocity, Mean Free Time, Mean Free Path 2.2.2 Mechanisms of Carrier Scattering EXAMPLE: Given  p = 470 cm 2 /V·s, what is the hole drift velocity at There are two main causes of carrier scattering: E = 10 3 V/cm? What is  mp and what is the distance traveled between 1. Phonon Scattering collisions (called the mean free path )? Hint: When in doubt, use the 2. Ionized-Impurity (Coulombic) Scattering MKS system of units. Solution:  =  p E = 470 cm 2 /V·s  10 3 V/cm = 4.7  10 5 cm/s Phonon scattering mobility decreases when temperature rises :  mp =  p m p /q = 470 cm 2 /V ·s  0.39  9.1  10 -31 kg/1.6  10 -19 C = 0.047 m 2 /V ·s  2.2  10 -12 kg/C = 1  10 -13 s = 0.1 ps 1 1        3 / 2 T phonon phonon phonon density  carrier thermal velocity T  T 1 / 2 mean free path =  mh  th ~ 1  10 -13 s  2.2  10 7 cm/s = 2.2  10 -6 cm = 220 Å = 22 nm  = q  /m  T v th  T 1/2 This is smaller than the typical dimensions of devices, but getting close. Slide 1-38 Slide 2-38 Slide 2-39 Slide 1-39 Total Mobility Impurity (Dopant)-Ion Scattering or Coulombic Scattering 1600 1 1 1 Boron Ion Electron   _ - - 1400    + Electron phonon impurity 1200 Electrons 1 1 1 Arsenic   -1 ) Ion 1000 -1 s    2 V phonon impurity 800 Mobility (cm 600 There is less change in the direction of travel if the electron zips by 400 Holes the ion at a higher speed. 200 3 3 / 2 v T 0    th impurity   N N N N 1E14 1E15 1E16 1E17 1E18 1E19 1E20 a d a d N a + N d (cm -3 ) -3 ) Total Impurity Concenration (atoms cm Slide 2-40 Slide 1-40 Slide 2-41 Slide 1-41 Temperature Effect on Mobility Velocity Saturation • When the kinetic energy of a carrier exceeds a critical value, it generates an optical phonon and loses the kinetic energy. 10 15 • Therefore, the kinetic energy is capped at large E , and the velocity does not rise above a saturation velocity, v sat . • Velocity saturation has a deleterious effect on device speed as Question : shown in Ch. 6. What N d will make d  n / dT = 0 at room temperature? Slide 1-42 Slide 2-42 Slide 1-43 Slide 2-43 2

02/05/2014 Hall Effect Slide 2-44 Slide 1-44 Slide 2-45 Slide 1-45 Slide 2-46 Slide 1-46 Slide 1-47 Slide 2-47 2.2.3 Drift Current and Conductivity 2.2.3 Drift Current and Conductivity E J p J p,drift = qpv = qp  p E unit area + J n,drift = –qnv = qn  n E  + J drift = J n,drift + J p,drift =  E =(qn  n +qp  p ) E A/cm 2 or C/cm 2 ·sec Hole current density J p = qpv  If p = 10 15 cm -3 and v = 10 4 cm/s, then conductivity (1/ohm-cm) of a semiconductor is EXAMPLE:  = qn  n + qp  p J p = 1.6  10 -19 C  10 15 cm -3  10 4 cm/s =   1 . 6 C/s cm 2 1.6 A/cm 2 1/  = is resistivity (ohm-cm) Slide 1-48 Slide 2-48 Slide 2-49 Slide 1-49 3

02/05/2014 Relationship between Resistivity and Dopant Density EXAMPLE: Temperature Dependence of Resistance (a) What is the resistivity (  ) of silicon doped with 10 17 cm -3 of arsenic? (b) What is the resistance (R) of a piece of this DOPANT DENSITY cm -3 silicon material 1  m long and 0.1  m 2 in cross- P-type sectional area? N-type Solution: (a) Using the N-type curve in the previous figure, we find that  = 0.084  -cm. (b) R =  L/A = 0.084  -cm  1  m / 0.1  m 2 = 0.084  -cm  10 -4 cm/ 10 -10 cm 2 RESISTIVITY (  cm) = 8.4  10 -4   = 1/  Slide 1-50 Slide 2-50 Slide 2-51 Slide 1-51 2.3 Diffusion Current EXAMPLE: Temperature Dependence of Resistance By what factor will R increase or decrease from T=300 K to T=400 K? Solution: The temperature dependent factor in  (and therefore  ) is  n . From the mobility vs. temperature curve for 10 17 cm -3 , we find that  n decreases from 770 at 300K to 400 at 400K. As a result, R increases by 770  1 . 93 400 Particles diffuse from a higher-concentration location to a lower-concentration location. Slide 2-52 Slide 1-52 Slide 2-53 Slide 1-53 2.3 Diffusion Current Total Current – Review of Four Current Components dn dp    J qD J qD n , diffusion n p , diffusion p dx J TOTAL = J n + J p dx D is called the diffusion constant. Signs explained: dn n p J n = J n,drift + J n,diffusion = qn  n E + qD n dx dp J p = J p,drift + J p,diffusion = qp  p E – qD p dx x x Slide 1-54 Slide 2-54 Slide 2-55 Slide 1-55 4

02/05/2014 2.4 Relation Between the Energy 2.5 Einstein Relationship between D and  Diagram and V, E Consider a piece of non-uniformly doped semiconductor. V ( x ) 0.7eV   ( E E ) / kT  0.7V n N e N-type semiconductor c f n-type semiconductor c x + – 0 N type Si N- dn N dE  ( E c  E ) / kT   c e c f Decreasing donor concentration dx kT dx E c and E v vary in the opposite E n dE Ec (x) direction from the voltage. That E c (x)   - c Ef (x) kT dx is, E c and E v are higher where E f the voltage is lower. n Ev (x)   q E dV 1 dE 1 dE c  0.7V kT   v E (x)= + E v (x) dx q dx q dx x Modern Semiconductor Devices for Integrated Circuits (C. Hu) Slide 2-56 Slide 1-56 Slide 2-57 Slide 1-57 2.5 Einstein Relationship between D and  EXAMPLE: Diffusion Constant dn n   q E dx kT What is the hole diffusion constant in a piece of dn silicon with  p = 410 cm 2 V -1 s -1 ?     J qn E qD 0 at equilibrium. n n n dx qD Solution:    0 E n E qn qn n kT   kT          D ( 26 mV ) 410 cm 2 V 1 s 1 1 1 cm 2 /s   kT kT p p q       D Similarly, D n n p p q q Remember: kT/q = 26 mV at room temperature. These are known as the Einstein relationship. Slide 1-58 Slide 2-58 Slide 2-59 Slide 1-59 2.6 Electron-Hole Recombination Charge Neutrality •The equilibrium carrier concentrations are denoted with •Charge neutrality is satisfied at equilibrium n 0 and p 0 . ( n ′ = p ′ = 0) . •The total electron and hole concentrations can be different • When a non-zero n ′ is present, an equal p ′ may from n 0 and p 0 . be assumed to be present to maintain charge • T he differences are called the excess carrier equality and vice-versa. concentrations n’ and p’. •If charge neutrality is not satisfied, the net charge will attract or repel the (majority) carriers through   n n n ' the drift current until neutrality is restored. 0   p p p ' 0 n  ' p ' Slide 2-60 Slide 1-60 Slide 2-61 Slide 1-61 5