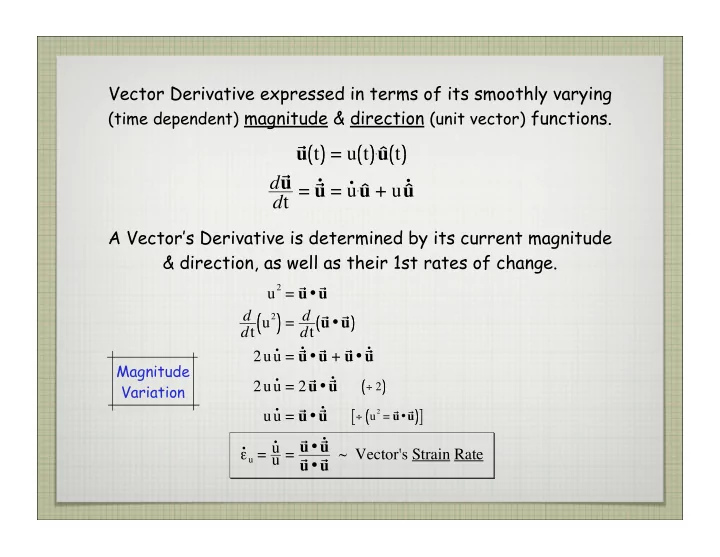
u t u t u t d u u
play

u t = u t u t d u = u u + u u d t = u A Vectors Derivative - PowerPoint PPT Presentation

Jan 03, 2023 •547 likes •662 views

Vector Derivative expressed in terms of its smoothly varying (time dependent) magnitude & direction (unit vector) functions. u t = u t u t d u = u u + u u d t = u A Vectors Derivative is determined by its current

  1. Vector Derivative expressed in terms of its smoothly varying (time dependent) magnitude & direction (unit vector) functions. u t � = u t � u t d u • � = u • • � u + u u d t = u A Vector’s Derivative is determined by its current magnitude & direction, as well as their 1st rates of change. 2 � = u • u u 2 � = d d d t u d t u • u • • u + u • u • • � = u 2uu Magnitude • • � = 2 u • u 2uu Variation ÷ 2 • • � = u • u uu 2 � = u • u ÷ u • • � = u • u u = u • u • u ~ Vector's Strain Rate � u

  2. • • � = u • u u = u • u • u ~ Vector's Strain Rate � u u acute acute • > 0 • u > 0 " increasing magnitude " � � u • u � � � • u • u obtuse • < 0 • u < 0 " decreasing magnitude " � � u • u � � � u u • = 0 • u = 0 " constant ( or extreme ) magnitude " � � u • u � � � • u In particular, for a (constant unit magnitude) unit vector function u = u t � � u = 1 = const . • = 0 � � • � u • = u • u u � u

  3. // to u � to u • = • • � u u u + u u • = • u • � u u u • u + u � u ; // - � decomposition with � = u • � u u u u • u = 1 = u • u + u � u ; u u u u • u + • � u 1 1 = 2 u • u 2 u � u u u • • u • u u � u 2 = u • u = u • u u + u • u � u ; u • • u � = u • u u • u = u • � u • = • u � u u + Q u � u ; • Q u � � u � u u • u = ? • u u u = + Q u � u • = • � u u u + Q u � u ; u = u u We will confirm • = • ÷ u Q u � u = ? u u ? Q u � u u � � check independently!

  4. Algebraic expansion of the Q (?) vector: • Q u = u � u u • u �� = 1 • • � u + u u 2 u u � u u � = 1 u u u � 1 • • � u + u u u u • � = u � u • � u + u u • • � = u � � u � u + u zero • • � = � u u � u + u � u • Q u = u � u • u • u �� = u � u Conclude: Q-vector is INDEPENDENT of u’s magnitude!!

  5. Moreover, • � u Q u � u � = u � u 2bl X-product switch • � u � = u � u DXP Identity • � u • • u u � = u • u u • � 0 u � = 1 u • Q u � u � = u So that, • • = Q u � u Q u � u � u • u • u �� = u � u & u which leads to the desired confirmation, viz • = u Q u � u = Q u � u u = Q u � u ; • u � = u u � check

  6. Summarize: • • � u + u u u • = u • • u � = u • u u • u = u • � u • u u + Q u � u ; � • • = ? Q u � � u � u u • u = u � u Next Issue: Geometric Interpretation of this Q-vector

  7. Geometric Interpretation of the Q-vector u (t) � u (t+ � t) � = 1 � 1 � sin �� N u � u + � u � = sin �� N N ~ RHRotation Rule Normal to u 's rotation plane 1 u � u + u �� u � = sin �� �� ~ angle of rotation (radians) �� N �� 0 + u �� u � = sin �� �� N u t+ � t = u + � u �� 1 � t � = sin �� �� �� u � � u u t = u � t N 1 �� e n l a P sin �� n i o �� a t o t � t � 0 u � � u R t � + t u lim � = lim � t N � t u �� � t � t � 0 1 Direction • n • � = � u � u Change • � ~ u 's instantaneous (inst.) angular rotation speed � n � ~ RHRotation Rule Normal to u 's inst. rotation plane

  8. This establishes the physical meaning of the Q-vector • • n • = � Q u � = u � u u • u = u � u as u’s instantaneous ANGULAR VELOCITY VECTOR - as indeed it is normal to u’s inst. plane of rotation, has a RHR rule sense, and a magnitude equal to u’s inst. angular rotation speed (rad/sec) . This crucial observation justifies the adoption of the formal name change Q u � � u

  9. and the consequent resummarization: • • � u + u u u • = u • • u � = u • u u • u = u • u ~ u 's strain rate � • u u + � u � u ; � • • n ~ u 's � • = � � u � = u � u u • u = u � u ) velocity vector

  10. n ~ RHRotation Rule normal to u 's inst. rotation plane 1 ~ u 's � TURN SIGNAL � � n � u � u 1 • u • � • u � u RHON triad 1 • = � u • • � u u // u u = u • u u = u (t) = u u � n � u 's instantaneous rotation plane • u � = � u � u • ~ u 's inst. angular rotation speed (rad/sec) • n � u u � = � � u ~ u 's inst. angular velocity vector � ~ u 's perpendicular (in-plane) "turning direction" • = u � n � u • = � • � • � • • • � u + u � u u u + � u � u = u u � = u �

  11. Rate of change of a Smoothly Varying Unit Vector Function n ~ RHRotation Rule normal to u 's inst. rotation plane 1 � � n � u ~ u 's � TURN SIGNAL � u 1 • u • � • � • 1 RHON triad � u u � n � u 's instantaneous rotation plane • ~ u 's inst. angular rotation speed (rad/sec) � � u ~ u 's inst. angular velocity vector • = � u � u = � • � � ~ u 's perpendicular (in-plane) "turning direction" u

Recommend

More recommend