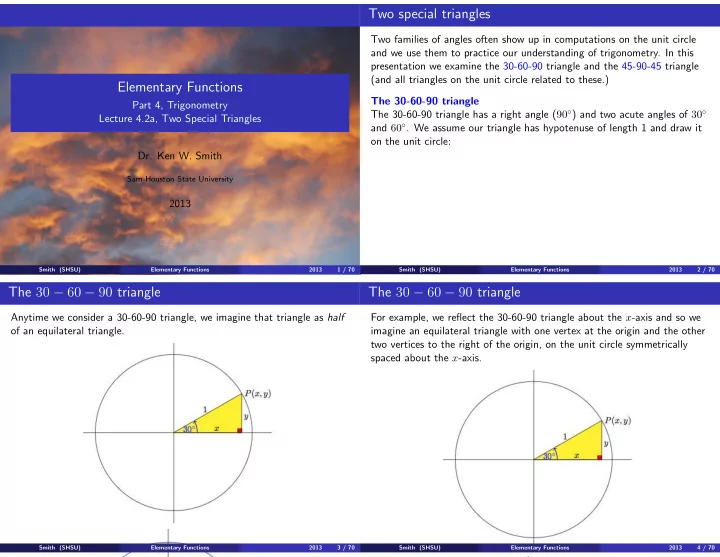
Two special triangles Two families of angles often show up in - PowerPoint PPT Presentation

Two special triangles Two families of angles often show up in computations on the unit circle and we use them to practice our understanding of trigonometry. In this presentation we examine the 30-60-90 triangle and the 45-90-45 triangle (and all triangles on the unit circle related to these.) Elementary Functions The 30-60-90 triangle Part 4, Trigonometry The 30-60-90 triangle has a right angle ( 90 ◦ ) and two acute angles of 30 ◦ Lecture 4.2a, Two Special Triangles and 60 ◦ . We assume our triangle has hypotenuse of length 1 and draw it on the unit circle: Dr. Ken W. Smith Sam Houston State University 2013 Smith (SHSU) Elementary Functions 2013 1 / 70 Smith (SHSU) Elementary Functions 2013 2 / 70 The 30 − 60 − 90 triangle The 30 − 60 − 90 triangle Anytime we consider a 30-60-90 triangle, we imagine that triangle as half For example, we reflect the 30-60-90 triangle about the x -axis and so we of an equilateral triangle. imagine an equilateral triangle with one vertex at the origin and the other two vertices to the right of the origin, on the unit circle symmetrically spaced about the x -axis. Smith (SHSU) Elementary Functions 2013 3 / 70 Smith (SHSU) Elementary Functions 2013 4 / 70

The 30 − 60 − 90 triangle The 30 − 60 − 90 triangle This triangle is an equilateral triangle with three equal sides of length 1 By the Pythagorean Theorem, unit. x 2 + ( 1 2 ) 2 = 1 2 so x 2 = 3 Since this equilateral triangle is symmetric about the x -axis, and since 4 each side has length 1, then the y -coordinates of the two vertices on the √ 3 and so the x -value of the points on the unit circle must be x = 2 . unit circle are ± 1 2 . Smith (SHSU) Elementary Functions 2013 5 / 70 Smith (SHSU) Elementary Functions 2013 6 / 70 The 30 − 60 − 90 triangle The 30 − 60 − 90 triangle √ √ 2 , 1 3 2 , − 1 3 The two points on the unit circle are P ( 2 ) and Q ( 2 ) . From this A similar argument with equilateral triangles works for an angle of 60 ◦ . √ we see that the cosine of 30 ◦ is 2 and the sine of 30 ◦ is 1 (We should always think of a 30 − 60 − 90 triangle as half of an equilateral 3 2 . triangle!) In the figure drawn below we see that the cosine of 60 ◦ is 1 2 and √ √ 3 2 and sin( − 30 ◦ ) = − 1 the sine of 60 ◦ is (Also cos( − 30 ◦ ) = 2 . ) 3 2 . Smith (SHSU) Elementary Functions 2013 7 / 70 Smith (SHSU) Elementary Functions 2013 8 / 70

The 30 − 60 − 90 triangle The 30 − 60 − 90 triangle and its siblings y (0 , 1) Any angle on the unit circle with a reference angle of 30 ◦ or 60 ◦ will have � √ � 2 , 1 3 √ coordinates that involve the numbers 1 3 2 2 and 2 . π 6 These angles include 30 ◦ , 60 ◦ , 120 ◦ , 150 ◦ , 210 ◦ , 240 ◦ , 300 ◦ 330 ◦ and so 30 ◦ ( − 1 , 0) (1 , 0) on.... x (0 , − 1) Smith (SHSU) Elementary Functions 2013 9 / 70 Smith (SHSU) Elementary Functions 2013 10 / 70 The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) (0 , 1) √ � � 1 3 2 , 2 π 2 π 3 90 ◦ 60 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 11 / 70 Smith (SHSU) Elementary Functions 2013 12 / 70

The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y √ (0 , 1) (0 , 1) � � − 1 3 2 , 2 √ � � 2 π 2 , 1 3 − 3 2 120 ◦ 5 π 6 150 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 13 / 70 Smith (SHSU) Elementary Functions 2013 14 / 70 The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) π x x 180 ◦ 210 ◦ 7 π 6 √ � � 2 , − 1 3 − 2 (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 15 / 70 Smith (SHSU) Elementary Functions 2013 16 / 70

The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x 240 ◦ 270 ◦ 4 π 3 3 π 2 √ � � − 1 3 2 , − 2 (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 17 / 70 Smith (SHSU) Elementary Functions 2013 18 / 70 The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x 330 ◦ 11 π 6 300 ◦ � √ � 5 π 2 , − 1 3 3 2 √ � � 1 3 2 , − (0 , − 1) 2 (0 , − 1) Smith (SHSU) Elementary Functions 2013 19 / 70 Smith (SHSU) Elementary Functions 2013 20 / 70

The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) (0 , 1) � √ � 2 , 1 3 2 13 π 6 390 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) 360 ◦ 2 π x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 21 / 70 Smith (SHSU) Elementary Functions 2013 22 / 70 The 30 − 60 − 90 triangle and its siblings The 30 − 60 − 90 triangle and its siblings y y (0 , 1) √ (0 , 1) � � 1 3 2 , 2 5 π 2 7 π 3 450 ◦ 420 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 23 / 70 Smith (SHSU) Elementary Functions 2013 24 / 70

√ √ cos π 2 , sin π 3 6 = 1 cos π 2 , sin π 3 6 = 1 6 = − 6 = 2 2 y y (0 , 1) (0 , 1) � √ √ � � � 2 , 1 3 2 , 1 3 − 2 2 π 5 π 6 6 30 ◦ 150 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 25 / 70 Smith (SHSU) Elementary Functions 2013 26 / 70 √ √ cos π 2 , sin π 3 6 = − 1 cos π 2 , sin π 3 6 = − 1 6 = − 6 = 2 2 y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x 210 ◦ 330 ◦ 7 π 11 π 6 6 � √ √ � � � 2 , − 1 3 2 , − 1 3 − 2 2 (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 27 / 70 Smith (SHSU) Elementary Functions 2013 28 / 70

√ √ cos π 6 = 1 2 , sin π 3 cos π 6 = − 1 2 , sin π 3 6 = 6 = 2 2 y y (0 , 1) √ (0 , 1) � � − 1 3 √ 2 , � � 1 3 2 , 2 2 π 2 π 3 3 60 ◦ 120 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 29 / 70 Smith (SHSU) Elementary Functions 2013 30 / 70 √ √ cos π 6 = − 1 2 , sin π 3 cos π 6 = 1 2 , sin π 3 6 = − 6 = − 2 2 y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x 240 ◦ 300 ◦ 4 π 5 π 3 3 √ √ � � � � − 1 3 1 3 2 , − 2 , − 2 (0 , − 1) (0 , − 1) 2 Smith (SHSU) Elementary Functions 2013 31 / 70 Smith (SHSU) Elementary Functions 2013 32 / 70

The 30 − 60 − 90 triangle The 45-90-45 triangle We collect this information in a table. The first two columns give the Another important triangle is the right triangle with both acute angles equal to 45 ◦ . Since this triangle has two equal angles then it is isoceles; it angle, first in degrees and then in radians. The last two columns give the also has two equal sides. We may assume that the hypotenuse has length x and y coordinates. 1 and so draw this triangle on the unit circle. cos( θ ) sin( θ ) θ θ √ π 3 1 30 ◦ 6 2 2 √ π 1 3 60 ◦ 3 2 2 √ 2 π 3 − 1 120 ◦ 3 2 2 √ 5 π 3 1 150 ◦ − 6 2 2 √ 7 π 3 − 1 210 ◦ − 6 2 2 √ 4 π 3 − 1 240 ◦ − 3 2 2 √ 5 π 1 3 300 ◦ − 3 2 2 √ 11 π 3 − 1 330 ◦ 6 2 2 I wouldn’t try to memorize this but instead be able to recreate it by proper use of the 30-60-90 triangle. Smith (SHSU) Elementary Functions 2013 33 / 70 Smith (SHSU) Elementary Functions 2013 34 / 70 The 45-90-45 triangle The 45-90-45 triangle Since the triangle is isoceles, its height y is equal to the base x . By the Pythagorean theorem, 1 cos(45 ◦ ) = sin(45 ◦ ) = √ 2 . x 2 + y 2 = 1 = ⇒ x 2 + x 2 = 1 = ⇒ 2 x 2 = 1 . ⇒ x 2 = 1 = 2 . 1 If we wish to get rid of the radical in the denominator, we can multiply = ⇒ x = 2 . √ √ numerator and denominator by 2 so that the equation becomes Since y = x then √ 2 1 cos(45 ◦ ) = sin(45 ◦ ) = 2 . √ cos(45 ◦ ) = sin(45 ◦ ) = 2 . Any angle θ in which the reference angle is 45 ◦ will have cosine and sine √ 2 equal to ± 2 . Here are some examples. Smith (SHSU) Elementary Functions 2013 35 / 70 Smith (SHSU) Elementary Functions 2013 36 / 70

The 45 − 90 − 45 triangle and its siblings The 45 − 90 − 45 triangle and its siblings y y (0 , 1) (0 , 1) � √ √ √ √ � � � 2 2 2 2 2 , − 2 , 2 2 π 3 π 4 4 45 ◦ 135 ◦ ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 37 / 70 Smith (SHSU) Elementary Functions 2013 38 / 70 The 45 − 90 − 45 triangle and its siblings The 45 − 90 − 45 triangle and its siblings y y (0 , 1) (0 , 1) ( − 1 , 0) (1 , 0) ( − 1 , 0) (1 , 0) x x 225 ◦ 315 ◦ 5 π 7 π 4 4 � √ √ √ √ � � � 2 2 2 2 − 2 , − 2 , − 2 2 (0 , − 1) (0 , − 1) Smith (SHSU) Elementary Functions 2013 39 / 70 Smith (SHSU) Elementary Functions 2013 40 / 70